How Logic "Really" Works: An Engineering Perspective

post by Daniil Strizhov (mila-dolontaeva) · 2025-04-16T05:34:09.443Z · LW · GW · 0 comments

Contents

  Symbol Legend: The Alphabet of Structural Thinking
  Where This Framework Shines
      Visual Pattern:
  The First Cut: Before "If-Then" Comes "This-Not This"
  From Boundaries to Forms (○)
  Reasoning's Architecture: How Forms (○) Enable Logic (⇒)
  Maps vs. Territories: Logic Guarantees Structure, Not Truth
  Fog & Phantom Bridges: Why Reasoning Fails (Unclear ↘)
      Live Example: "Does technology make people lonely?"
  The Structural Linter: Engineering Better Thinking by Debugging Distinctions
    The Linter Checklist: Your Structural Debugging Tool
    Example Application
  Resolving Disagreements: Finding the Underlying Distinctions (↘)
  The Echo of Distinction (↘): Developing Structural Intuition
    Practice Corner: Try the Linter
  Summary
None
No comments

When you hear "logic," what comes to mind? Probably strict rules, truth tables, or the classic "if A, then B." It all seems clear—until you try to apply it to real life, where things rarely fit so neatly.

But what if logic isn't just about formulas? What if, at its core, it's about something even simpler: noticing the difference between one thing and another?

Textbooks start with symbols: P → Q, ∀x, ∃y. But maybe real logic begins earlier—at the moment we first draw a line between "this" and "not this."

This article offers a look at logic from an engineering angle. The goal isn't to throw out what you know, but to add a new tool. Sometimes, even a small shift in perspective can make disagreements clearer and reasoning more robust.

From an engineering standpoint, this is like analyzing a bridge's aerodynamics without examining its foundation.

 

Symbol Legend: The Alphabet of Structural Thinking

Note:
The symbols are not the point—they are just a way to make invisible structure visible. The real value is in the clarity and robustness of your reasoning.

Before we dive in, a quick note: these symbols are used as signposts to help notice the structure beneath the surface. You don't need to memorize them—just refer back as needed.

Symbol	Name	Description	Role
↘	Distinction	The fundamental act of creating a boundary, separating 'this' from 'not-this'.	The initiator.
○	Form	That which is defined and bounded by a Distinction (↘).	The resulting element.
⇒	Transformation / Inference	An asserted link or valid transition between established Forms (○).	The connection/path.
∅	Void / Undefined	A gap where inference (⇒) fails due to unclear ↘ or ill-defined ○.	Error: missing base.
⟲	Loop	Circular reasoning; a ⇒ pattern returning to its start without progress.	Pattern: useless cycle.
✶	Goal	The intended target Form (○) or state of a reasoning process.	The target marker.
✘	Contradiction	A clash where Forms (○) or Transformations (⇒) are incompatible.	Error: internal conflict.

This table is here for quick reference. It helps map and debug the structure of thought as you read. Now, let's see where logic actually begins.

 

Where This Framework Shines

In precise, formalized problems (like mathematics or classical logic puzzles), standard inference rules (⇒) are usually enough: the boundaries and forms are already clear.

But in real-world, "chaotic" questions—where terms are vague, boundaries are fuzzy, and disagreements are common—reasoning often fails not because of faulty logic, but because the necessary distinctions (↘) and forms (○) are missing or unclear.
The structural framework is most powerful in these situations: it helps turn conceptual chaos into structured reasoning.

Visual Pattern:

• Precise: ↘ → ○ → ⇒ → ✶
• Chaotic: ∅, ↘?, ○?, ⇒??, ✘, ⟲

The goal of the framework is to transform the second line into the first.

 

The First Cut: Before "If-Then" Comes "This-Not This"

Imagine a world without differences—a perfect homogeneity where nothing can be distinguished from anything else. In such a world, could you form a single thought? Could you make a single statement? Could logic exist at all?

No. Because logic begins with a more basic act: recognizing that something is "not the same" as something else.

Before you can ever construct the familiar "If A, then B," you first need to establish that A is A and B is B, and implicitly, that "A is not B." Without this separation, logical operations have nothing to operate on—no identifiable elements to connect.

This root gesture—the creation of difference or separation—is what can be called ↘ (Distinction). It's more than just label assignment. It's the primitive cognitive act that carves structure from what is undifferentiated. Without it, information itself cannot exist.

Think about it: without separation, there is nothing to reason about—no subject, no object, no function. Even conceptualizing "nothingness" involves distinguishing it from "somethingness." The very concept of a void appears when a boundary is drawn around what is not there.

But distinction alone isn't enough. Something emerges from these separations.

 

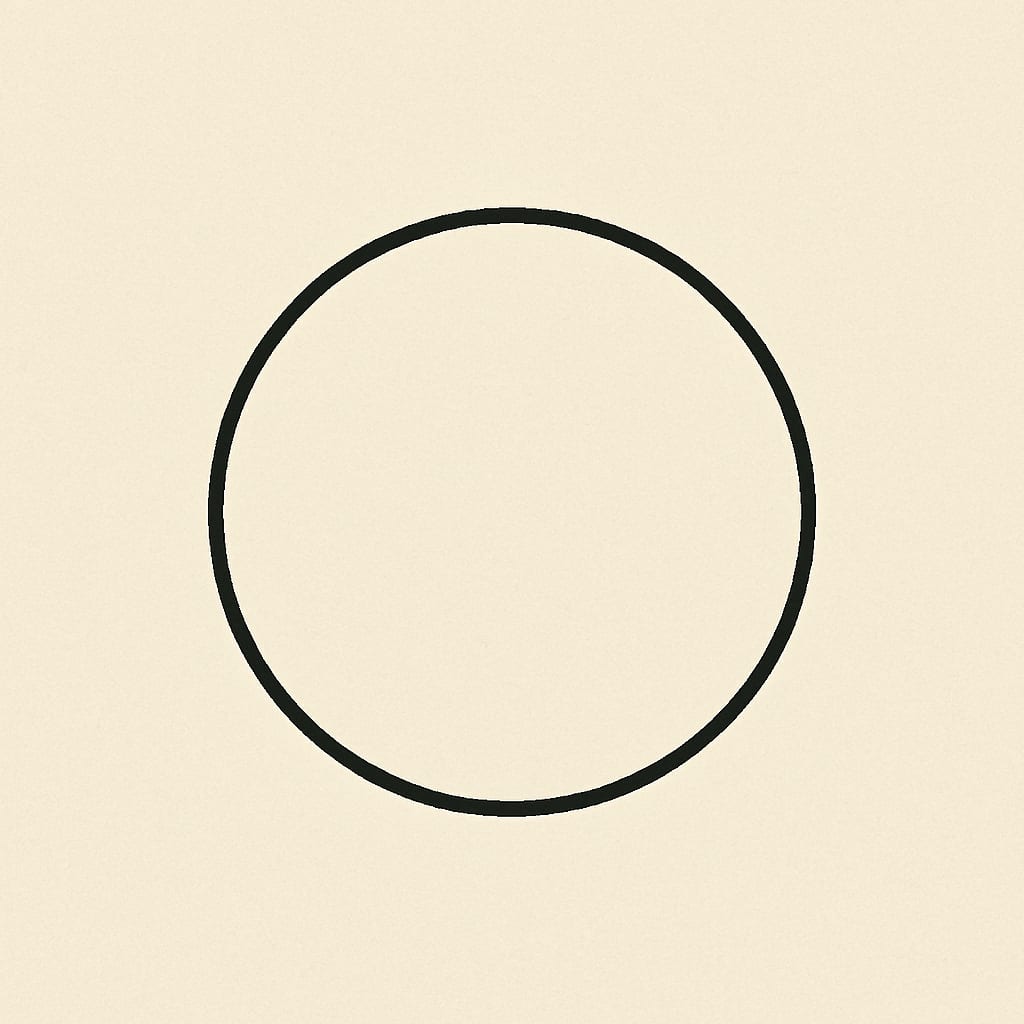
From Boundaries to Forms (○)

An act of distinction doesn't just create a boundary—it immediately brings forth a Form (○).

So, what is a Form (○)? It's not just "a thing" passively observed. Every entity or concept can be seen as what becomes visible when a boundary is drawn. The boundary isn't an afterthought; it constitutes the Form's (○) identity in that context.

Picture drawing a circle on a blank page. The circle exists because inside is distinguished from outside. The line is the creation of the Form (○).

A Form (○) can represent anything referenced in thought or language: physical objects like "this coffee cup" (distinguished from everything that is not this cup), abstract concepts like "justice" (separated from "injustice" or "mercy"), states like "user logged in" (versus "logged out"), or system boundaries like "inside the application" (versus the external environment).

This principle shows up in engineering too. When designing software, "inside the application" is separated from "outside the application." That shapes code structure, error handling, and dependencies. In hardware design, distinguishing "signal" from "noise" determines where shielding is placed. In systems thinking, defining system boundaries influences what is optimized for and what is treated as external.

Here's the key: Every concept, object, or state referenced is already a Form (○) — a remembered or currently active Distinction (↘).

Forms (○) aren't isolated. They can contain other Forms (○) through nested distinctions (↘). A "car" contains an "engine," "wheels," and other components, each requiring its own boundary. This recursive structure of separations within separations creates the complexity of the conceptual landscape.

Once Forms (○) are in place, we can look at how they become the building blocks of logical systems.

 

Reasoning's Architecture: How Forms (○) Enable Logic (⇒)

Only after establishing well-defined Forms (○) through clear Distinctions (↘) does logic operate. Logic doesn't generate truth from nothing. It describes valid transitions and relationships between Forms (○) that have already been distinguished.

This perspective doesn't invalidate formal logic but rather gives it a foundation. Take the classic syllogism: "Socrates is a man; all men are mortal; therefore, Socrates is mortal." This already assumes distinctions that define "men" as separate from "non-men" and "mortal" from "immortal." But what if formal logic only works because these separations are already in place?

When "If A, then B" is asserted, what is really being said? First, that A represents a well-distinguished Form (○). Second, that B also represents a well-distinguished Form (○). And third, that a valid transformation (⇒) actually connects Form A to Form B within the defined context.

The transformation ⇒ can mean many things: deductive entailment, causal connection, functional dependency, or other structured links. Different logical systems (deductive, inductive, abductive) essentially propose different kinds or strengths of transitions between established Forms (○).

The key engineering observation is:

The validity of any inference (⇒) depends entirely on the structural integrity of the Forms (○) it connects, which depends on the clarity of the underlying Distinctions (↘).

If Forms (○) are fuzzy or ill-defined (due to unclear ↘), inference (⇒) spans a void (∅). This doesn't mean the conclusion is necessarily wrong. It might feel intuitively right or persuade rhetorically. But structurally, the reasoning is unstable—built on shifting conceptual sand rather than solid distinctions.

So, if logic depends on the quality of distinctions, what is its relationship to truth?

 

Maps vs. Territories: Logic Guarantees Structure, Not Truth

Logic doesn't, by itself, prove anything true about the world. What it does is show structural consequences if Distinctions (↘), Forms (○), and transformations (⇒) hold. This key distinction is often missed.

Truth is about whether Forms (○) correspond to reality—this is the domain of epistemology. Logic, on the other hand, is about the internal consistency of a system—more like geometry.

Take an architectural blueprint. It's a logically consistent map (⇒ relationships between material Forms ○). But if the real materials are faulty or ground conditions differ (misaligned ○ due to bad initial ↘), the structure fails despite the blueprint's internal logic. The map was coherent, but didn't match the territory.

Or think of a GPS. It calculates routes (⇒) based on its map data (road Forms ○ from Distinctions ↘). Its internal logic can be perfect. But if the map (○) is outdated (a road closure missing), it leads nowhere (∅/✘ vs. Goal ✶). Again, coherent logic within the map doesn't guarantee real-world success if the Forms (○) misrepresent the territory.

Logic guarantees coherence within the defined system. It does not guarantee the system accurately maps to reality.

This aligns with the map-territory distinction, a concept popularized by Korzybski and often discussed in rationality circles, including LessWrong.

If misalignment with reality is not the most common flaw in reasoning, what is? This question helps explain why so many arguments feel unsatisfying despite appearing logical.

 

Fog & Phantom Bridges: Why Reasoning Fails (Unclear ↘)

The most common reasoning failure is not incorrect inference (⇒) but omission—trying to infer across a void (∅) where necessary Distinctions (↘) have not been clearly established, leaving the Forms (○) undefined.

Live Example: "Does technology make people lonely?"

• What is "technology"? (Is it social media, smartphones, all digital tools, or something else? The distinction ↘ is unclear.)
• What is "lonely"? (Is it subjective feeling, measurable isolation, or lack of meaningful connection? The form ○ is fuzzy.)
• What is the nature of the link? (Direct causation, correlation, or context-dependent? The transformation ⇒ is undefined.)

Without clarifying these, any argument about the claim is likely to span a void (∅) or result in contradiction (✘).
The framework helps reveal and repair these structural gaps.

Here's a more technical example: defining an API endpoint. Suppose the requirement is simply 'retrieve user data'. Without clear Distinctions (↘), what Form (○) does 'user data' represent? Does it include profile info? Activity logs? Purchase history? Security credentials? If different teams (frontend, backend, security) make different implicit distinctions (↘), the resulting API (⇒) might either expose too much data (security risk, ✘) or too little (functional gap, ∅), leading to integration failures. The root cause is not faulty implementation logic (⇒), but the initial lack of clear distinction (↘) for the required data Form (○).

Once the problem is diagnosed, the next step is to look for ways to make thinking more structurally sound.

 

The Structural Linter: Engineering Better Thinking by Debugging Distinctions

Instead of asking only "Is this argument true?", an engineering mindset asks: "Is this argument structurally sound?"

Note: This approach does not replace classical logic, but extends its applicability to cases where formal rules alone are powerless due to unclear distinctions and forms.

Practice shows: even when the analysis is performed by a language model, the structural checklist itself prevents key distinctions, voids, and boundaries from being missed. An LLM, following these points, begins to articulate what usually remains implicit—making the analysis deeper and clearer.

The Linter Checklist: Your Structural Debugging Tool

Reasoning can be treated like code that benefits from review. This checklist highlights common structural weaknesses:

1. Examine Core Distinctions (↘): Are the fundamental separations clear and consistent? Fuzzy or missing distinctions mean a void (∅) is present—clarity here is foundational.
2. Assess Defined Forms (○): Are the resulting concepts or entities stable and well-bounded? Ill-defined Forms (○) create shaky foundations (∅), making reliable inference difficult.
3. Validate Transformations (⇒): Are the asserted links appropriate for the connected Forms (○)? Unsupported leaps or assumptions may be hiding in the inference itself (∅).
4. Identify Voids (∅): Where does reasoning attempt to bridge undefined gaps, relying on unclear Distinctions (↘) or Forms (○)? This is a common structural failure point. The appearance of a void (∅) is not a logical error, but an invitation to revisit and clarify the underlying distinctions and forms.
5. Detect Loops (⟲): Does the reasoning (⇒) circle back on itself without producing new insight? Loops (⟲) often mask unexamined Distinctions (↘).
6. Check Goal (✶) Alignment: Is the stated conclusion (the target Form ○ or state) actually reachable via the proposed path (⇒)? Or is the structure inherently incapable of leading there (∅, ✘)?
7. Scan for Contradictions (✘): Does the structure contain internal inconsistencies, such as clashing Forms (○) or incompatible Transformations (⇒)? Contradictions (✘) signal deep structural flaws, but also point to places where distinctions or forms need to be re-examined.

This checklist is not about finding flaws for their own sake; it is a way to observe and refine distinctions. Here are a few ways to explore this:

• Question Boundaries: When encountering a key term (especially in disagreements or complex problems), consider: "What exactly is being separated from what here?" "Where is the line drawn?" "Could it be drawn differently?"
• Seek Operational Definitions: How might the distinction (↘) be measured or tested? Turning abstract concepts into concrete tests can clarify boundaries. If a distinction cannot be operationalized, its resulting Form (○) is likely too fuzzy (∅).
• Hunt for Edge Cases: What examples sit right on the boundary? Do they break the distinction? Edge cases can stress-test conceptual structures.
• Consider Alternatives: Are there other, perhaps more useful, ways to carve up this conceptual space?

This is a bit like practicing scales in music or reviewing code line-by-line. Deliberate attention to distinctions is a core skill for structural clarity.

Insight: An insight is not just a new thought, but the discovery of a previously unmarked distinction, form, or connection. Practically every breakthrough in understanding is the result of making explicit what was previously undifferentiated or unformed.

Example Application

Here is how this works in practice. Take the claim: "Video games cause violence in children."

Working through the checklist:

First, examining the Core Distinctions (↘): "Video games" draws a line (↘) between games and non-games, but this distinction is fuzzy. Similarly, "violence" is ambiguous. These unclear boundaries (↘) are the first warning sign.

Looking at the resulting Forms (○): Both "video games" and "violence in children" lack the clear boundaries needed for stable reasoning. Without specifying what counts, these Forms (○) are too unstable to support reliable inference (⇒).

The Transformation (⇒) claims a causal link, but this is not justified and ignores alternatives. It's a leap across a significant gap (∅).

Several Voids (∅) can be identified where the inference (⇒) tries to connect fuzzy Forms (○) without addressing context or mechanisms.

There aren't obvious Loops (⟲) initially, though defenders might introduce them.

The Goal (✶) of establishing causation is structurally unreachable via the path (⇒) offered due to fuzzy Distinctions (↘) and resulting Voids (∅).

Finally, while there are no internal Contradictions (✘) in this simple statement, empirical data might introduce them.

This analysis highlights the need for clarification at the foundation: the Distinctions (↘) themselves. Until they are clarified, the argument is built on fog (∅).

What changes in interactions when this approach is applied? The implications for resolving disagreements are significant.

 

Resolving Disagreements: Finding the Underlying Distinctions (↘)

When logic is seen as primary, disagreements often become battles over who has the "correct" rules or who applies them better. These battles can be endless and fruitless, with each side convinced of their logical superiority.

But when logic is understood as secondary to distinction, the focus shifts.

Instead of asking "Is your conclusion correct?", consider:

"What distinctions (↘) are being made that lead to this inference (⇒)?"

And reflexively:

"What distinctions (↘) am I making?"

Disagreements often do not exist at the level of inference (⇒). They reside in the initial, often unstated, distinctions (↘) that shape the very Forms (○) being discussed.

Two people arguing about the "best solution" might be operating with fundamentally different distinctions about what constitutes the "problem." Someone advocating for economic growth and someone advocating for environmental protection may appear to disagree on priorities, when in fact they've drawn different boundaries around the concept of "prosperity"—one excluding and one including environmental health.

When underlying distinctions are clarified first, logical structures often resolve naturally or reveal their exact points of divergence. This can shift conversations from battles to explorations, from "who's right" to "what are we actually talking about."

This leads to a new way of understanding what logic is and how to develop it as a skill.

 

The Echo of Distinction (↘): Developing Structural Intuition

Logic, as presented here, is not the source code of reason. It is not a set of prescriptive commands. It is not a guarantee of truth.

Logic is the echo of distinction (↘) — the trace left by the act of separation as it shapes conceptual space. It describes the structural relationships (⇒) that emerge between well-formed distinctions (○).

You can say that insight arises where attention (intention) meets a point of structural tension—an ambiguity, contradiction, or void. It is in these places that new distinctions and forms are born, making thinking more robust.

To reason effectively and build robust systems, it can be helpful to look deeper than surface transformations. Foundations can be examined. The quality of logic (⇒) is ultimately constrained by the quality of distinctions (↘).

A core practice is a constant, focused inquiry: "What boundary has actually been drawn here (↘)"? followed by, "Given only that boundary, what structure (○) and consequences (⇒) necessarily follow?"

Making good distinctions is a skill that develops through observation, precise questions, and deconstructing hidden assumptions. This art of separation invites further exploration.

As this awareness of distinction as the foundation of thought grows, structural integrity can become an intuition rather than a procedure. It changes not just what is thought, but how thinking itself is approached. The linter becomes internalized; structural weaknesses in reasoning are felt before they can be articulated. For example, there may be a vague discomfort when someone claims policy X is "better" than policy Y, but hasn't said what "better" means. That feeling can signal a missing distinction (↘) or an undefined form (○).

In this transformation lies the value of understanding logic as it really works—not as a set of rules to follow, but as the natural echo of how the world is carved up in the mind (↘ leading to ○ and ⇒).

---

Practice Corner: Try the Linter

Consider applying the linter checklist to these common assertions:

• "Early rising increases productivity."
• "Social media is harmful."

What Distinctions (↘) need clarification? What Forms (○) are ill-defined? Where do you spot potential Voids (∅)?

You may wish to compare a typical answer to a complex question with one structured by this checklist—the difference in depth and clarity will be obvious. Even a language model, without human understanding, produces a more detailed and useful analysis when it follows these steps.

Summary

In formal logic, the rules are enough. In real-world reasoning, structure is everything.
This framework is a tool for building that structure where it's needed most—especially in the messy, ambiguous, or "chaotic" problems of everyday life and complex debates.

This approach does not contradict classical logic, but rather makes its foundation—distinctions and forms—explicit and available for analysis. It is a practical extension for those cases where formal rules alone are not enough.

0 comments

Comments sorted by top scores.