Why the Kaldor-Hicks criterion can be non-transitive
post by Rupert · 2022-06-12T17:26:13.611Z · LW · GW · 10 commentsContents
10 comments
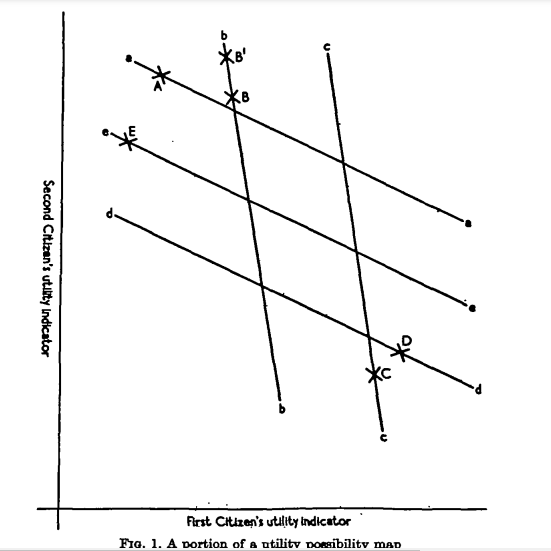
The following post aims to explain why the diagram below is a proof without words that the Kaldor-Hicks criterion for an improvement in the economy can be non-transitive. This is in response to a request for help from a student of mine who made the request on the Facebook group Bountied Rationality, and hoped that a LessWrong post could be drawn up explaining the matter in detail.
Let me briefly explain the meaning of the terms. A Pareto improvement in an economy is a change in the state of the economy where every individual in the economy is at least as well off as before, and some individuals are strictly better off. A Kaldor improvement in an economy is a change in the state of the economy where some individuals are better off, and a re-allocation of resources would be possible so that they would be able to compensate any individuals who have been made worse off by the change, so that the net result is a Pareto improvement. The criterion of a Kaldor improvement is not anti-symmetric; it is possible for there to be two distinct states of the economy A and B such that each one is a Kaldor improvement of the other. This motivates the following. We say that a state B is a Kaldor-Hicks improvement of A if B is a Kaldor improvement of A but A is not a Kaldor improvement of B. This criterion is anti-symmetric.
In the diagram below, the x-axis and y-axis respectively represent utilities for Citizen 1 and Citizen 2. A state of affairs further to the right is preferred by Citizen 1, a state of affairs further upwards is preferred by Citizen 2. Only ordinal relations between utilities matter; we are not assuming a cardinal utility measure. The curves represent sets of combinations of utilities attainable by re-distribution from a given state of the economy. It is possible for two distinct curves to have an intersection point, that means that there are two different possible states and allocation of resources of the economy which give rise to the same pair of utilities.
The point B' represents a Pareto improvement over the point A since it is both upwards and to the right. B is a Kaldor improvement of A since B' is attainable from B by re-distribution and B' is Pareto-better than A. On the other hand, no point on the curve passing through A is a Pareto-improvement over B, so A is not a Kaldor improvement over B. Thus B represents a Kaldor-Hicks improvement over A.
Using this kind of reasoning, one may confirm from the diagram that B is a Kaldor-Hicks improvement over A, C is a Kaldor-Hicks improvement over B, D is a Kaldor-Hicks improvement over C, but D is not a Kaldor-Hicks improvement over A. Thus this proves the non-transitivity of the relation in some possible circumstances.
10 comments
Comments sorted by top scores.
comment by Viliam · 2022-06-12T20:40:52.295Z · LW(p) · GW(p)
Why do you sometimes assume that re-distribution is possible along the lines a, e, d, and sometimes that it is possible along the lines b, c? It seems to me that the entire non-transitivity is explained by the fact that you changed the rules of re-distribution in the middle of the argument.
Replies from: Rupert↑ comment by Rupert · 2022-06-13T06:52:30.739Z · LW(p) · GW(p)
All of the curves represent states of the economy such that a re-distribution of resources will correspond to a movement along that curve. A change in state of the economy can be explained by a change in technological knowledge, a change in climate, the discovery of a deposit of a particular resource, stuff like that. "Re-allocation of resources" can correspond to re-allocation of quite a complex bundle of goods. The projections of the points on the co-ordinate axes merely represent the utilities of each of the two citizens, where only the order relations between utilities matters.
Replies from: Rupert↑ comment by Rupert · 2022-06-13T06:54:05.825Z · LW(p) · GW(p)
Oh yes, and when the economy is in a given state you are only allowed to move along one curve. The curves are allowed to intersect, but you can't change which curve you are moving along when doing re-distribution. The explanation I just added of why the curves are allowed to intersect may help.
comment by JBlack · 2022-06-13T03:33:51.326Z · LW(p) · GW(p)
I notice I am confused.
I don't see why A is not a Kaldor improvement over B. Economic redistribution can get us to the intersection of lines a and c and thence up to a Pareto improvement over B.
Is the idea that only the marked points can possibly exist? No, that can't be true since it is stated that C is a Kaldor improvement over B, which would require the existence of some unmarked state on line c that is a Pareto improvement over B.
I remain confused. Every marked point in this diagram seems to be reachable by economic redistribution from all the others so I don't see how any of them can be Kaldor-Hicks improvements.
Replies from: Rupert↑ comment by Rupert · 2022-06-13T06:55:10.639Z · LW(p) · GW(p)
Each curve corresponds to a "state of the economy". To get to a state where you could start re-distributing by moving along a different curve to the one you were originally moving along would require a change of state. When re-distributing, you can only move along one curve corresponding to the current state of the economy.
Replies from: JBlack↑ comment by JBlack · 2022-06-13T11:34:25.707Z · LW(p) · GW(p)
Oh, I see: Kaldor-Hicks improvement is a family of relations that depend upon the underlying economic state, not just a single relation. So in that diagram there are five states of the economy, and a separate Kaldor-Hicks relation for each one. In most of the economic states, only one of the points permits any redistribution at all.
This seems ... kinda useless? Are you sure you're interpreting the diagram correctly?
Replies from: Rupert↑ comment by Rupert · 2022-06-14T13:41:10.873Z · LW(p) · GW(p)
A Kaldor-Hicks improvement is a change of state of the economy from A to B such that B can be converted into a Pareto improvement by re-distribution, and such that A cannot be converted into a Pareto improvement of B by re-distribution.
Every labelled point on that diagram permits re-distribution (because it lies on a curve).
↑ comment by JBlack · 2022-06-14T23:23:10.935Z · LW(p) · GW(p)
They all permit re-distribution in different states of the economy though, so I'm not sure why they're even on the same diagram except to save the space of having a different diagram for each economic state.
So for example, there is a curve labelled a, which corresponds to a particular economic state and points can be moved only along that curve in that state (per your earlier clarification). Point C is not on curve a, and so no redistribution is possible from there in economic state a.
Replies from: Rupert↑ comment by Rupert · 2022-06-15T09:41:29.822Z · LW(p) · GW(p)
Point C is a particular combination of utilities. The particular combination of utilities is not attainable via re-distribution while the economy is in state a. If a change took place so that the economy was now in state c, then point C would be attainable by re-distribution.
(And there is a point common to both the curves a and c, but just from knowing that the utilities of Citizens 1 and 2 were at that particular point wouldn't allow you to know whether the economy is in state a or c, that would be extra information, and this extra information would be necessary in order to know which other points you could get to via re-distribution from your current situation.)
Replies from: JBlack↑ comment by JBlack · 2022-06-15T23:14:33.620Z · LW(p) · GW(p)
Ah good, I didn't misunderstand that then. I'm still confused as to why there are not 5 different Kaldor-Hicks improvement relations, one for each state of the economy, instead of just one. In the following passage in the post:
Using this kind of reasoning, one may confirm from the diagram that B is a Kaldor-Hicks improvement over A, C is a Kaldor-Hicks improvement over B, D is a Kaldor-Hicks improvement over C, but D is not a Kaldor-Hicks improvement over A.
B is a KHI over A in state b, but not in any other state. C is a KHI over B in state c, but not in any other state. As far as I can see, there in no economic state (and therefore no KHI relation) in which both B is a KHI over A and C is a KHI over B, and so the question of transitivity is irrelevant.