Build Your Number Sense

post by DirectedEvolution (AllAmericanBreakfast) · 2021-01-27T21:01:27.803Z · LW · GW · 4 comments

Contents

    Magnitude: The Difference Between 1 And 2
  1% is not a small number.
    Larger Magnitudes
    Part 2: Introduction
    Variance Is The Spice Of Life
  Math is as real as it gets.
None
4 comments

Nobody has ever called math sensual. But it is!

Magnitude: The Difference Between 1 And 2

Take a look at these two numbers:

1 vs. 2

Are these small numbers or large numbers? Is the difference between them important?

If they represent the number of dates you and I have been on, that's a meaningful difference! If they're counting the pennies we have in our pockets, it's probably not.

What if we attach one more character?

1% vs. 2%

If these figures represent your chances of winning a free box of Girl Scout cookies in one of two different raffles, it's probably not worth stressing over. If they represent your chance of dying from a surgical operation at two different hospitals, then maybe it is. After all, your chances of dying are twice as high in the second hospital as they are in the first.

Notice that when I said twice as high just now, I wasn't, strictly speaking, telling you anything new. I was trying to make you feel different about the numbers 1 and 2. What if I did it like this:

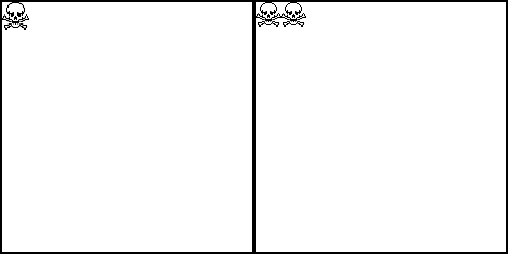
Or like this:

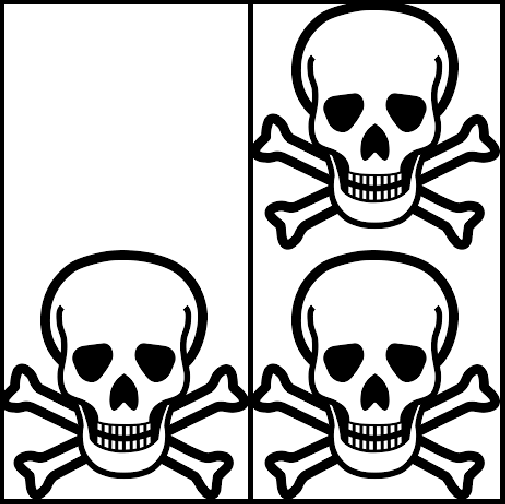
And here is a picture of some happy flowers just to lighten the mood:

Notice how your response changes to these numbers depending on the way I visually represent it. Numbers are blessed and cursed by the way we write them. 1 and 2 are handy little figures, easy to manipulate with equations. But they also are about the same size on the page, written in a way that's disconnected from the physical things they're representing.

The difference may or may not be important. What's "small" and what's "large?" Well, it depends on your intuitions about the subject we're talking about. In fact, 1 and 2 don't inherently have a property of "bigness" or "littleness" in any physical sense. The number 1 can be an absolutely gigantic number.

So let's say it together, loud and clear:

1% is not a small number.

---

Larger Magnitudes

This dilemma gets even more serious the higher we go. At the time of writing, here's what a thousand, a million, and a billion dollars can get you.

For a thousand dollars, you can buy this 2002 Subaru Outback:

For a million dollars, you can buy Pointe Au Baril island in British Columbia:

For a billion dollars, you can buy the Miami Marlins:

The size of our numbers doesn't scale with their magnitude. This is an extremely useful feature. But the drawback is that it strips away the visceral component, the number sense. A billion is a thousand millions. Here's what just 10% of $1,000,000,000 looks like:

$1,000,000 $1,000,000 $1,000,000 $1,000,000 $1,000,000 

$1,000,000 $1,000,000 $1,000,000 $1,000,000 $1,000,000 

$1,000,000 $1,000,000 $1,000,000 $1,000,000 $1,000,000 

$1,000,000 $1,000,000 $1,000,000 $1,000,000 $1,000,000 

$1,000,000 $1,000,000 $1,000,000 $1,000,000 $1,000,000 

$1,000,000 $1,000,000 $1,000,000 $1,000,000 $1,000,000 

$1,000,000 $1,000,000 $1,000,000 $1,000,000 $1,000,000 

$1,000,000 $1,000,000 $1,000,000 $1,000,000 $1,000,000 

$1,000,000 $1,000,000 $1,000,000 $1,000,000 $1,000,000 

$1,000,000 $1,000,000 $1,000,000 $1,000,000 $1,000,000 

$1,000,000 $1,000,000 $1,000,000 $1,000,000 $1,000,000 

$1,000,000 $1,000,000 $1,000,000 $1,000,000 $1,000,000 

$1,000,000 $1,000,000 $1,000,000 $1,000,000 $1,000,000 

$1,000,000 $1,000,000 $1,000,000 $1,000,000 $1,000,000 

$1,000,000 $1,000,000 $1,000,000 $1,000,000 $1,000,000 

$1,000,000 $1,000,000 $1,000,000 $1,000,000 $1,000,000 

$1,000,000 $1,000,000 $1,000,000 $1,000,000 $1,000,000 

$1,000,000 $1,000,000 $1,000,000 $1,000,000 $1,000,000 

$1,000,000 $1,000,000 $1,000,000 $1,000,000 $1,000,000 

$1,000,000 $1,000,000 $1,000,000 $1,000,000 $1,000,000

Just 9 more blocks of this and we'll get to a billion!

And finally, here's a billion dollars in hundred dollar bills:

As my linear algebra teacher told me, "if a thousand dollars is your arm span, a billion dollars is down the block."

And that was an understatement!

Unfortunately, we're stuck. There are huge advantages to being able to write numbers the way we usually do, in Arabic numerals. It was honestly kind of a hassle for me to come up with all these pictures, and you can't expect that other people are going to do you that kind of favor. Not even when your life depends on it.

Finally, note that this holds true in the opposite direction. A millionth is tiny relative to a thousandth just as much as a thousand is tiny relative to a million. Try visiting the 1/1,000th, 1/10,000th, and 1/100,000th-scale worlds to start getting a feel for a fraction of the difference between a thousandth and a millionth.

---

Part 2: Introduction

Let's look closely at three sets of numbers:

Set 1: 1, 4, 7, 8

Set 2: 2, 2, 8, 4

Set 3: 1, 13, 1, 5

Before I tell you what this is all about, what do you notice? Possibly nothing. See any patterns?

What if I was handing you boxes that weighed this many pounds? Try to imagine what that would feel like. First you hold a series of boxes that weigh 1, 4, 7, and 8 pounds. Then I hand you a set of boxes that weigh 2, 2, 8, and 4 pounds. Finally, I hand you boxes that weigh 1, 13, 1, and 5 pounds. Do you think that one of these sets of boxes is not like the others?

---

Variance Is The Spice Of Life

Which set do you think is more "spread out?" In which set are the numbers more "closely packed together?" If you have an intuition about the answer, remember it for later.

But what does that question even mean?

Let's give an example.

If you're a bantamweight boxer, you'll be fighting people who are very close to the same weight as you, in the range of 116-118 pounds. Lightweight is 131-135 pounds. Heavyweight is 201 pounds or more. So here are the weights of some boxing champions:

Bantamweight: 116, 118, 117, 117

Lightweight: 131, 134, 135, 135

Heavyweight: 240, 254, 284, 220

Notice how the fact that "heavyweight" has no upper limit means that the numbers can vary much more widely. You can intuitively feel that, just by looking at them in comparison with the bantamweight and lightweight weigh-in numbers.

Coming back to our first set of numbers, it turns out that set 3 is most "spread out," while set 2 is most "closely packed." We can calculate the amount of spreading with something called the variance, but I'll spare you. Let's just say that heavyweight boxers have more variance in their weights than the other weight classes.

The important point here is that your "number sense" can detect how spread out these numbers are. But you might not pick up on it unless you know what you're looking for. Sometimes, as with the boxing weight classes, the variance might be so obvious that even without having it pointed out, you might notice it, even if you didn't have a good word to use to describe what you saw.

Other times, as in set 2 vs. set 3 in the first set of numbers, you might be able to tell the difference only if your attention is called to the variance first. And other times, you might need a precise mathematical formula to be sure that there's a real difference in the variance. Take another look at that first set, and see if your gut can give you a sense for whether set 1 or set 2 has greater variance:

Set 1: 1, 4, 7, 8

Set 2: 2, 2, 8, 4

Set 3: 1, 13, 1, 5

Honestly, even if I think about it really hard, looking for patterns and justifications for why we'd consider set 1 to be more spread out than set 2, it's very hard to tell. Turns out there is a precise mathematical formula to calculate the variance. But what it's doing is helping us to give structure and precision to our "number sense."

If I told you that the "total variance" of this little set of numbers was equal to 50, it would be precisely correct, but wouldn't hit home with your number sense. If you're ever studying math, it can help to try to directly see the patterns that the precise formulas are teasing out of the numbers.

But you can't always count on other people to activate your number sense when they show you statistics, or teach you a formula. So I leave you with this:

Math is as real as it gets.

Make it real for yourself!

 

---

Note: As Daniel points out in the comments, Number Sense is already a subject of psychological research. You can find a book on it here.

4 comments

Comments sorted by top scores.