[Retracted] Newton's law of cooling from first principles
post by Nisan · 2024-01-16T04:21:11.094Z · LW · GW · 15 commentsContents
Rates of change The Lambert cooling law Experimental support? Conclusion None 15 comments
This post is wrong. See this comment [LW(p) · GW(p)].
A high-school physics textbook will tell you that, according to Newton's law of cooling, a hot thing will cool down according to
where is heat, is time, is temperature, and is the temperature of the environment. But this is slightly wrong! It turns out that the correct cooling rate, which I'll call Lambert's law, is
.
(Newton's law approximates this pretty well.) Let's derive this from first principles, and try to test it empirically.
Epistemic status: Logical deductions from a speculative physical model. I don't actually know nonequilibrium thermodynamics.
Rates of change
First, a lemma: I claim that if is any macroscopic variable, then the rate of change of is proportional to the derivative of the entropy with respect to :
Why? Suppose our system changes in discrete timesteps, and can only change in steps of size . And suppose the probability of a transition is proportional to the number of microstates . Then you can calculate the expected change in per timestep, and from there prove the lemma.[1]
The Lambert cooling law
Now let our system comprise two subsystems. There's a fixed amount of heat split between the subsystems. Apply our lemma, taking to be , the amount of heat in subsystem 1. We find that[2]
Here the inverse of temperature, or coldness, shows up. The coldness of a system is defined to be the derivative of entropy with respect to energy. (The only energy here is heat.)
Now if subsystem 2 is very big, its temperature doesn't change much. So let's rename to , to ; and to , because that's the temperature subsystem 1 will have in the limit:
We can use this to get an expression for involving the second derivative of entropy, which is related to the heat capacity:
However, textbooks tend to assume that the heat capacity is temperature-independent, in which case heat is roughly proportional to temperature. So we have
This differential equation has a neat solution after a change of variables. Let
Then the solution is exponential decay:
where is the value of at , and is a constant. If you want to express the solution in terms of , you'll need the Lambert W function.
Experimental support?
Newton's law is a linear approximation of the Lambert law. If is close to (and both are far from absolute zero), then the two laws are hard to distinguish.
I tried to distinguish them anyway, by measuring a hot baking sheet with a cooking thermometer. A best-fit Newton law predicts the data pretty well. The best-fit Lambert law is quite close to Newton (within 3 degrees Kelvin), but is a *worse* fit than Newton. I think my experimental setup has too much error to distinguish the two laws.
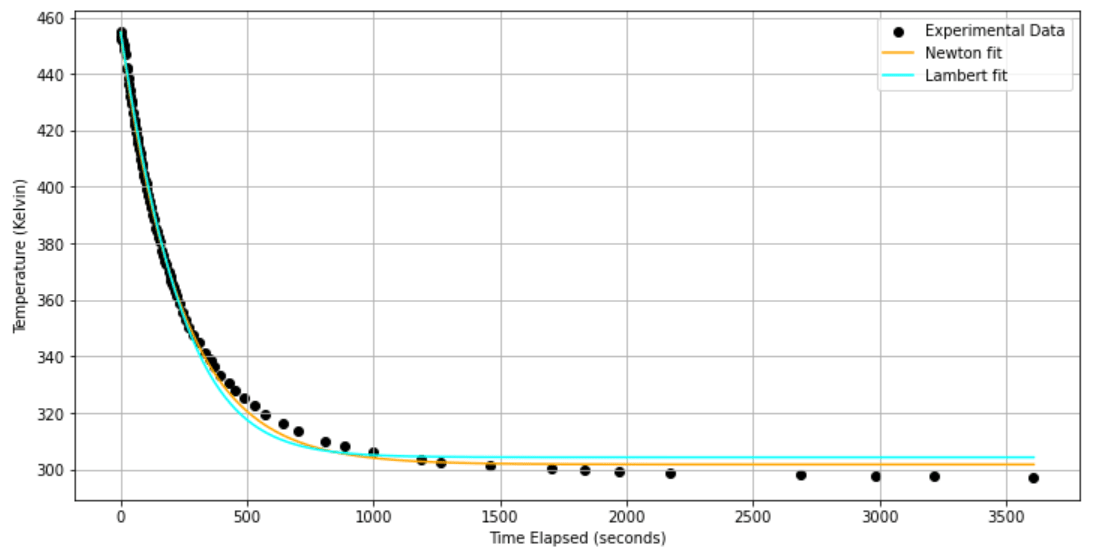
I'd guess a proper experiment would have to control convection, prevent the environment from heating up, and explicitly account for changing heat capacity. Cooling down to liquid nitrogen temperatures would make Lambert easier to distinguish from Newton, but that might be harder to work with.
Conclusion
If this cooling law is described somewhere in the nonequilibrium thermodynamics literature, I'd love to see a reference to it. Also, I'd love to know more about the assumption I started with: That a macroscopic variable must change gradually, and that the transition probabilities are proportional to the number of microstates; it seems relevant to dynamics in the same way that ergodicity is relevant to equilibrium.
- ^
Hints: Remember that . Also, to first order, the denominator is approximately .
- ^
Hints: The entropy of the whole system is the sum of the entropies of the subsystems, . And .
15 comments
Comments sorted by top scores.
comment by Nisan · 2024-07-20T00:42:58.428Z · LW(p) · GW(p)
This post is wrong. Thanks to SymplecticMan [LW(p) · GW(p)] for the thought experiment demonstrating that a mixture of ideal gases follows a law rather than my proposed law. (It's also different from Newton's law.)
I made a pretty but unjustified assumption — that a cooling baking sheet can be modeled as a dynamical system where each possible transition is equally likely and in which heat is transferred in fixed quanta, one at a time. This contradicted Newton's law, and I got excited when I realized that Newton's law was merely a first-order approximation.
My mistake was not noticing that Newton's law is a first-order approximation to any model of cooling where heat transfer increases with temperature difference, so I had not observed any reason to favor my model over any other.
In penance I have acquired a copy of Non-Equilibrium Thermodynamics by de Groot and Mazur, with the intention of eventually reading it.
Replies from: bhauthcomment by gjm · 2024-01-16T14:56:21.174Z · LW(p) · GW(p)
(Disclaimer: I am a mathematician, not a physicist, and I hated the one thermodynamics course I took at university several decades ago. If anything I write here looks wrong, it probably is.)
Let's try to do this from lower-level first principles.
The temperature is proportional to the average kinetic energy of the molecules.
Suppose you have particles with (mass,velocity) and and they collide perfectly elastically (which I believe is basically what happens for collisions between individual molecules). The centre-of-mass frame is moving with velocity relative to the rest frame, and there the velocities are and . In this frame, the collision simply negates both velocities (since this preserves the net momentum of zero and the net kinetic energy) so now they are and , so back in the rest frame the velocities are and . So the first particle's kinetic energy has changed by (scribble scribble) .
(I should say explicitly that these velocities are vectors and when I multiply two of them I mean the scalar product.)
Now, the particles with which any given particle of our cooling object comes into contact will have randomly varying velocities. I think the following may be bogus but let's suppose that the direction they're moving in is uniformly random, so the distribution of v2 is spherically symmetrical. (It may be bogus because it seems like two particles are more likely to collide when their velocities are opposed than when they are in the same direction, and if that's right then it will induce a bias in the distribution of v2.) In this case, the v1 v2 term averages out to zero and for any choice of |v2| we are left with constant . (KE2 - KE1). So as long as (1) our possibly-bogus assumption holds and (2) the rate at which object-particles and environment-particles interact isn't changing, the rate of kinetic energy change should be proportional to the average value of KE2-KE1, which is to say proportional to the difference in termperatures.
This is the (unmodified) Newton cooling law.
So if my calculations are right then any wrongness in the Newton law is a consequence of assumptions 1 and 2 above failing in whatever ways they fail.
I think assumption 2 is OK provided we keep the environment at constant temperature. (Imagine an environment-molecule somewhere near the surface of our object. If it's heading towards our object, then how long it takes before it collides with an object-molecule depends on how many object-molecules there are around, but not on how they're moving.)
I am suspicious of this "Lambert's law". Suppose the environment is at absolute zero -- nothing is moving at all. Then "Lambert's law" says that the rate of cooling should be infinite: our object should itself instantly drop to absolute zero once placed in an absolute-zero environment. Can that be right?
Replies from: Nisan, Nisan↑ comment by Nisan · 2024-01-17T08:17:55.361Z · LW(p) · GW(p)
I am suspicious of this "Lambert's law". Suppose the environment is at absolute zero -- nothing is moving at all. Then "Lambert's law" says that the rate of cooling should be infinite: our object should itself instantly drop to absolute zero once placed in an absolute-zero environment. Can that be right?
We're assuming the environment carries away excess heat instantly. In practice the immediate environment will warm up a bit and the cooling rate will become finite right away.
But in the ideal case, yeah, I think instant cooling makes sense. The environment's coldness is infinite!
↑ comment by Nisan · 2024-01-17T08:07:13.742Z · LW(p) · GW(p)
Oh neat! Very interesting. I believe your argument is correct for head-on collisions. What about glancing blows, though?
Assume two rigid, spherical particles with the same mass and radius.
Pick a coordinate system (at rest) where the collision normal vector is aligned with the x-axis.
Then move the coordinate system along the x axis so that the particles have equal and opposite x-velocities. (The y-velocities will be whatever.) In this frame, the elastic collision will negate the x-velocities and leave the y-velocities untouched.
Back in the rest frame, this means that the collision swaps the x-velocities and keeps the y-velocities the same. Thus the energy transfer is half the difference of the squared x-velocities, .
I'm not sure that's proportional to ? The square of the x-velocity does increase with temperature, but I'm not sure it's linear. If there's a big temperature difference, the collisions are ~uniformly distributed on the cold particle's surface, but not on the hot particle's surface.
Replies from: gjm↑ comment by gjm · 2024-01-17T12:13:20.816Z · LW(p) · GW(p)
Hmm. You're definitely right that my analysis (if it deserves so dignified a name) assumes all collisions are head-on, which is wrong. If "the x-axis" (i.e., the normal vector in the collision) is oriented randomly then everything still works out proportional to the kinetic energies, but as you say that might not be the case. I think this is basically the same issue as the possible bogosity of the "possibly-bogus assumption" in my original analysis.
Dealing with this all properly feels like more work than I want to do right now, though :-).
comment by Algon · 2024-01-16T13:04:24.623Z · LW(p) · GW(p)
.
How's this square up the the Stefan Bolztmann law? I.e., for a black body, the power radiated is proportional to . So if you had two black bodies radiating all their energy at each other, with one acting as a resevoir, you'd get a net outward flux of power of the form , right?
Replies from: Nisan, shankar-sivarajan↑ comment by Nisan · 2024-01-17T08:28:22.304Z · LW(p) · GW(p)
Yeah, as Shankar says, this is only for conduction (and maybe convection?). The assumption about transition probabilities is abstractly saying there's a lot of contact between the subsystems. If two objects contact each other in a small surface area, this post doesn't apply and you'll need to model the heat flow with the heat equation. I suppose radiative cooling acts abstractly like a narrow contact region, only allowing photons through.
↑ comment by Shankar Sivarajan (shankar-sivarajan) · 2024-01-17T04:05:35.525Z · LW(p) · GW(p)
Newton's law of cooling, which this is proposed as a correction to, only describes conduction. It's motivated by kinetic energies transferring via collisions.
comment by SymplecticMan · 2024-01-17T17:44:47.064Z · LW(p) · GW(p)
I'm going to open up with a technical point: it is important, not only in general but particularly in thermodynamics, to specify what quantities are being held fixed when taking partial derivatives. For example, you use this relation early on:
.
This is a relationship at constant volume. Specifically, the somewhat standard notation would be
,
where U is the internal energy. The change in internal energy at constant volume is equal to the heat transfer, so it reduces to the relationship you used.
That brings us to the lemma you wanted to use:
.
To get what you wanted, it has to actually be the derivative with constant volume on the right, but then there's a problem: it doesn't succeed in giving you the time derivative of V since .
Let's assume that problem with the lemma can somehow be fixed, though, for sake of discussion. There's another issue, which is that if the proportionality depends on thermodynamic variables, then you can have basically any relationship. For example, your heat equation:
.
If these proportionalities were and , it would actually give Newton's law of cooling. For an ideal gas, the equation of state means that the change in internal energy (which is just the heat transfer at constant volume) ought to be directly proportional to the temperature change, with no dependence on other thermodynamic variables (besides ) in the proportionality.
Now we have your formula for the derivative of temperature:
.
As a side note, I'm not sure how this is a heat capacity; it doesn't match any of the heat capacity formulas I remember. But the appearance of is notable; it makes it look a lot closer to Newton's law of cooling, and comparing it to the earlier equation for heat shows how the proposed proportionalities from the first lemma contain dependence on other thermodynamic variables. But you changed from and to and before this, so it's worth remembering that there should be a symmetric relationship between the two subsystems. Multiplying both of the inverse temperature terms by a single temperature produces an asymmetry in the time derivatives for the two subsystems.
This asymmetry in the temperature dependence would predict that one subsystem will heat faster than the other subsystem cools, which would tend to violate energy conservation. If we just imagine an ideal gas in two separate with an identical number of particles in each container, any temperature increase in one gas has to be exactly compensated by an identical magnitude temperature decrease in the other gas, since the internal energy is just proportional to temperature.
So I argue that this proposed law does not hold up.
Replies from: Nisan↑ comment by Nisan · 2024-01-17T20:01:08.689Z · LW(p) · GW(p)
constant volume
Ah, so I'm working at a level of generality that applies to all sorts of dynamical systems, including ones with no well-defined volume. As long as there's a conserved quantity , we can define the entropy as the log of the number of states with that value of . This is a univariate function of , and temperature can be defined as the multiplicative inverse of the derivative .
if the proportionality depends on thermodynamic variables
By
I mean
for some constant that doesn't vary with time. So it's incompatible with Newton's law.
This asymmetry in the temperature dependence would predict that one subsystem will heat faster than the other subsystem cools
Oh, the asymmetric formula relies on the assumption I made that subsystem 2 is so much bigger than subsystem 1 that its temperature doesn't change appreciably during the cooling process. I wasn't clear about that, sorry.
Replies from: adele-lopez-1, SymplecticMan↑ comment by Adele Lopez (adele-lopez-1) · 2024-01-18T05:38:04.902Z · LW(p) · GW(p)
in thermodynamics is not a conserved quantity, otherwise, heat engines couldn't work! It's not a function of microstates either.
See http://www.av8n.com/physics/thermo/path-cycle.html for details, or pages 240-242 of Kittel & Kroemer.
↑ comment by SymplecticMan · 2024-01-17T22:33:33.668Z · LW(p) · GW(p)
Ah, so I'm working at a level of generality that applies to all sorts of dynamical systems, including ones with no well-defined volume. As long as there's a conserved quantity , we can define the entropy as the log of the number of states with that value of . This is a univariate function of , and temperature can be defined as the multiplicative inverse of the derivative .
You still in general to specify which macroscopic variables are being held fixed when taking partial derivatives. Taking a derivative with volume held constant is different from one with pressure held constant, etc. It's not a universal fact that all such derivatives give temperature. The fact that we're talking about a thermodynamic system with some macroscopic quantities requires us to specify this, and we have various types of energy functions, related by Legendre transformations, defined based off which conjugate pairs of thermodynamic quantities they are functions.
By
I mean
for some constant that doesn't vary with time. So it's incompatible with Newton's law.
And I don't believe this proportionality holds, given what I demonstrated between the forms for what you get when applying this ansatz with versus with . Can you demonstrate, for example, that the two different proportionalities you get between and are consistent in the case of an ideal gas law, given that the two should differ only by a constant independent of thermodynamic quantities in that case?
Oh, the asymmetric formula relies on the assumption I made that subsystem 2 is so much bigger than subsystem 1 that its temperature doesn't change appreciably during the cooling process. I wasn't clear about that, sorry.
Since it seems like the non-idealized symmetric form would multiply one term by and the other term by , can you explain why the non-idealized version doesn't just reduce to something like Newton's law of cooling, then?
Here is some further discussion on issues with the law.
For an ideal gas, the root mean square velocity is proportional to . Scaling temperature up by a factor of 4 scales up all the velocities by a factor of 2, for example. This applies not just to the rms velocity but to the entire velocity distribution. The punchline is, looking at a video of a hot ideal gas is not distinguishable from looking at a sped-up video of a cold ideal gas, keeping the volume fixed.
Continuing this scaling investigation, for a gas with collisions, slowing down the playback of a video has the effect of increasing the time between collisions, and as discussed, slowing down the video should look like lowering the temperature. And given a hard sphere-like collision of two particles, scaling up the velocities of the particles involved also scales up the energy exchanged in the collision. So, just from kinetic theory, we see that the rate of heat transfer between two gases must increase if the temperature of both gases were increased by the same proportionality. This is what Newton's law of cooling says, and it is the opposite of what your proposed law says.
Here is a further oddity: your law predicts that an infinitely hot heat bath has a bounded rate of heat exchange with any system with a finite, non-zero temperature, which similar to the above, doesn't agree with how would understand it from the kinetic theory of gases.
Replies from: Nisan↑ comment by Nisan · 2024-01-18T00:20:00.801Z · LW(p) · GW(p)
Scaling temperature up by a factor of 4 scales up all the velocities by a factor of 2 [...] slowing down the playback of a video has the effect of increasing the time between collisions [....]
Oh, good point! But hm, scaling up temperature by 4x should increase velocities by 2x and energy transfer per collision by 4x. And it should increase the rate of collisions per time by 2x. So the rate of energy transfer per time should increase 8x. But that violates Newton's law as well. What am I missing here?
Replies from: SymplecticMan↑ comment by SymplecticMan · 2024-01-18T01:05:26.282Z · LW(p) · GW(p)
Material properties such as thermal conductivity can depend on temperature. The actual calculation of thermal conductivity of various materials is very much outside of my area, but Schroeder's "An Introduction to Thermal Physics" has a somewhat similar derivation showing the thermal conductivity of an ideal gas being proportional to based off the rms velocity and mean free path (which can be related to average time between collisions).