Seeking PCK (Pedagogical Content Knowledge)
post by CFAR!Duncan (CFAR 2017) · 2022-08-12T04:15:28.181Z · LW · GW · 11 commentsContents
Case study: understanding division None 11 comments
Author's note: This was originally included in the flash class section and has been broken out on advice from readers, to be placed at the front of the handbook. It was usually a full class at the start of the workshop, and was in the flash class section merely because no fully-fledged writeup exists.
A lot of teacher training in the USA focuses on broad teaching techniques that apply to just about any topic. Whether the topic is math, history, biology, or literature, teachers need to know how to design lesson plans and how to gain and keep control in the classroom. These domain-general teaching skills sometimes get referred to collectively as pedagogical knowledge (PK).
This is in contrast to content knowledge (CK), which is the teacher’s particular expertise in the topic being taught (e.g. knowledge of how to solve a quadratic equation).
However, in practice it’s helpful to notice that there’s a kind of knowledge that is both PK and CK. The educational profession refers to this as pedagogical content knowledge (PCK). This is knowledge about the topic being taught that is also about how students interact with the topic (and therefore how to teach that content more effectively).
For instance: what are common misconceptions about this domain? What are bad habits that typically need to be unlearned? What kinds of prompts or stimuli will actually help people identify and unlearn those bad habits, as opposed to sounding good while failing to do the trick? What's it like to be a beginner? What's it like to transition from beginner status to kind-of-sort-of having your feet under you (while still having lots of gaps or deficits)?
Teachers who actively try to develop PCK tend to gain a much more refined understanding of their topic, including a keen sense of which parts matter, how those parts connect, and in what order they must be explained (which is another way of saying which concepts are more fundamental, and which concepts require others as prerequisites). We encourage you to try keeping an eye out for PCK any time you begin to learn a new skill or start exploring a new domain. It will not only enrich your experience, but also make you much more likely to be able to pass the knowledge on to others.
Case study: understanding division
Suppose you’re trying to introduce the idea of division to elementary school students. You might start with a word problem like this one:
Johnny has 12 apples. He also has 4 friends who really love apples. If he gives all his apples away to his 4 friends and each friend gets the same number of apples, how many apples does each friend get?
Given some simple hands-on learning tools, a lot of elementary school students will want to count out twelve tokens and then sort them into four piles one at a time: “One for A, one for B, one for C, one for D, one for A...” They’ll stop when they run out of apple tokens, count the number in one pile, and conclude correctly that each friend gets three apples.
The teacher might then write the following on the board:
12 ÷ 4 = 3
... and say that what they’ve just done is “division,” which means that you are dividing some quantity into equal parts and looking at how much each part gets. This definition will work just fine, until the teacher introduces a problem that looks something like this:
Johnny has 12 apples. He wants to make gift bags that each contain 4 apples. How many gift bags can he make?
This will often befuddle students who have been taught that division is equal sharing. Given the problem above, the majority of elementary students tend to make one of two errors:
- Some students will gather twelve tokens and start counting them out into piles: “One for A, one for B, one for C... ” But after a while, they realize that they don’t know when to stop making piles—when to go back and put another token in pile A.
- Some students will notice that four is smaller than twelve, dutifully make four piles, and sort their twelve tokens into four piles. They note that there are three tokens per pile at the end, and they proudly (and almost correctly) say that the answer is three. But in this case, if the teacher follows up and asks "three what?" the student will often say "Three apples!"
The problem is that the process for using tokens to solve this problem looks fundamentally different: the student has to do something like gather four tokens at a time and set them aside, repeat this until there aren’t any tokens left, and then count the number of collections of four tokens that have been pulled aside.
It turns out that although both word problems are represented by the symbols 12 ÷ 4 = 3, the 4 and 3 mean different kinds of things in the two problems. In the first, the equation looks like this:
(# of items) ÷ (# of groups) = (# of items per group)
And in the second, the equation looks like this:
(# of items) ÷ (# of items per group) = (# of groups)
The first version is called partitive division (after “partition”), or “equal sharing.” The second one is quotitive division (after “quotient”), or “repeated subtraction.” And even though they’re both technically forms of division and there’s a mathematical isomorphism between the two operations, they are cognitively different. In practice, you have to teach young children about these two kinds of division separately first, before you start trying to show them that they’re both unified by an underlying concept.
In this case, the PCK is awareness of the fact that there are two different kinds of division, and that students get confused if you introduce them under the same umbrella. It’s knowledge about content (partitive and quotitive division) that is relevant to knowledge about pedagogy (successful teaching requires careful disambiguation).
The main tool for developing PCK is cultivating curiosity about the students’ experience. When teaching or tutoring, rather than asking “How can I convey this idea?” or “How can I correct this person’s mistake?”, instead ask “What is it like for this person, as they encounter this material?”
The PCK on the two types of division came in part from interviewing students who were working on division problems. Sometimes the students would make errors, and the interviewer would become curious. They’d wonder what thought processes might have caused the child to make the particular mistake they did, and then try to figure out ways of testing their guesses.
For instance, maybe a child uses an equal-sharing process with tokens to solve a repeated-subtraction word problem. Rather than trying to correct the child, the interviewer might investigate whether the child is running an algorithm, asking “So, what do these tokens in these piles mean to you?”
In education research, this is sometimes called clinical interviewing, and it’s a skill that requires attention and practice. For instance, the interviewer in the above example will be less effective if they spend part of the time trying to point out the error. Instead, the interviewer has to be simply wondering— being actually curious about the child’s thinking. If the curiosity is genuine and central in the interviewer’s mind, they’re more likely to notice interesting threads to pursue and to think of useful questions to pose.
This also tends to encourage a certain kind of reflection in the student. For instance, when a child in a clinical interview thinks the interviewer is trying to get them to do something or correct a mistake, they will often start to focus on pleasing the interviewer instead of focusing on whether or not things make sense. Sometimes they become nervous or self-conscious, and other times they sacrifice effort for appearance. In contrast, a curious and effective interviewer keeps the child engaged with the problem and pointed toward comprehension.
This isn’t always the best teaching method—sometimes, it’s helpful just to give direct and clear instruction. But in general, both you and those whom you teach will gain a lot more from the experience if you keep yourself curious about the learning experience rather than on whether information has been dutifully presented.
And this goes double when the person you're teaching is yourself.
11 comments
Comments sorted by top scores.
comment by Tanya Grinyuk (Cuprum Buddy) (tatyana-grinyuk) · 2023-06-16T12:32:58.778Z · LW(p) · GW(p)
I believe that PCK itself is incredibly helpful, but, unfortunately, there isn't much material on how to actually use this technique as a self-learner when there's no teacher around to be curious about your mistakes and guide your way through your subject of choice.
I fully agree with the idea to be kind to yourself and reflect on what exactly went wrong in your line of thought instead of blaming yourself for errors, but sometimes you just get stuck at some point: you know that something isn't right, but you don't know what exactly even when you can fully recall what you were doing, so you can't define the mistake without help and, consequently, fix it. In my opinion, such situations can be crucially demotivating, and I wonder whether there's a way to use PCK more effectively aside from making self-reflection a habit.
comment by Viliam · 2022-08-12T13:31:12.672Z · LW(p) · GW(p)
I think that in Slovakia and Czechia, this style of teaching is referred to as "constructivist education".
On the other hand, for English-speaking audience, the word "constructivism" seems to refer to quite different things (1, 2, 3). And when I try to explain concepts like this in English, I sometimes get surprising responses when people seem to automatically run along the chain of associations: "trying to understand the student's model" = "constructivism" = "you should never explain math" = "math wars" = "total failure".
I tried to figure out how this could have happened, and my current best guess is that in the past some people in USA promoted some really stupid and harmful ideas under the banner of "constructivism", which made people associate the word "constructivism" with those stupid ideas. Meanwhile, some of the original good ideas are still taught, but carefully under different labels. (Longer version here.) So perhaps the people who want to learn how to teach well, could find something useful in the writings of Piaget and Vygotsky. However, a Google search for "constructivism" might just return a list of horror stories.
By the way, I would expect pedagogical content knowledge of STEM topics to be super rare, because it requires an intersection of being good at psychology and math. And, at least in my experience, psychologists are often quite math- and tech-phobic. On the other hand, people good at math often fail to empathize with the beginners, and just keep writing complex equations, preferably without explanation of what the symbols mean.
Replies from: Valentine↑ comment by Valentine · 2022-08-12T16:11:50.354Z · LW(p) · GW(p)
On the other hand, for English-speaking audience, the word "constructivism" seems to refer to quite different things (1, 2, 3). And when I try to explain concepts like this in English, I sometimes get surprising responses when people seem to automatically run along the chain of associations: "trying to understand the student's model" = "constructivism" = "you should never explain math" = "math wars" = "total failure".
IIRC, this was the result of trying to implement the good version of constructivism in the USA. It wasn't just that some people had bad ideas and called those "constructivism" too. It's that when math education reformers tried to spread constructivism, it kept getting watered down at each step.
This is actually what caused me to abandon my career in academia. About a year before I finished my PhD (in math education), this picture clicked into place for me, and I realized that no amount of research into how to teach math better could possibly affect anything. If there was any leverage, it would be systemic and/or political, not in terms of the details of curriculum design or teacher education.
And yet, all my professors — from whom I learned this picture! — were researching exactly these pointless topics. All my time as a grad student research assistant was spent collecting & processing classroom data and discussing implications for curricular design and teacher education.
I was quite confused at the time and asked one of my professors about it. "What's the game plan?" I asked. "If the mechanisms behind the math wars are still present, but basically everyone is still researching better classroom technique… what's the vision? Are some people striving to change government too? Is there a PR effort? I don't follow."
A crestfallen look washed over him, and he replied:
"I try not to think about that. If I do, I start getting depressed."
That was the end of my giving a fuck about my academic field.
…which, incidentally, resulted in me joining forces with Anna to build CFAR.
By the way, I would expect pedagogical content knowledge of STEM topics to be super rare, because it requires an intersection of being good at psychology and math. And, at least in my experience, psychologists are often quite math- and tech-phobic. On the other hand, people good at math often fail to empathize with the beginners, and just keep writing complex equations, preferably without explanation of what the symbols mean.
In practice I agree.
There's actually quite a lot of known PCK in STEM. But in practice it can't get into the classrooms at any scale that matters.
The best book on math PCK I know of is Reconceptualizing Mathematics by Nickerson, Sowder & Sowder. Well, it's really the only book on math PCK I know of. But it's quite rich.
The book is based largely on Cognitively Guided Instruction, which is maybe the most thoroughly researched constructivist education method in math ed. Extremely good results across the board. The only trouble is that making it work requires a much more involved teacher education process than the current American (and more general?) incentive structures can allow for. The teacher education methods have also been carefully studied, and it looks to take about two years of classroom experience with regular check-ins and coaching.
But if you want to glean some marvelous math PCK on arithmetic, algebra, and geometry, I've never seen anything that can even hold a candle to this book.
And once you sort of develop an eye for PCK, you can start digging it up for yourself. It doesn't even require a ton of research (although doing things like clinical interviews really does go far, far deeper and gives you way more opportunities to falsify your hypotheses; confirmation bias is a bitch here). Just, if you're explaining some STEM concept and the person doesn't quite follow, ask yourself why. See if you can predict other points of confusion in them. Get curious about the other's mind.
…if you care.
(I think there's a good argument to be made that mastering PCK sight is key for a full Art of Rationality, especially anything you'd want to call "group rationality". But at this stage that's still little more than guesswork with a peppering of anecdotes.)
Replies from: Viliam↑ comment by Viliam · 2022-08-14T00:56:34.227Z · LW(p) · GW(p)
Thanks for the book recommendation!
when math education reformers tried to spread constructivism, it kept getting watered down at each step.
Could you please explain how specifically this happens? What exactly is the mechanism behind the math wars? Is it the fact that most math teachers suck at math, and don't even try to be better, and have no incentive to do so; or did you have (also) something else in mind?
As far as I know, most math teachers do suck at math, and don't even try to be better, but I have two hopes here. (1) It could be the case that there are two possible stable equilibria: "the population in general sucks at math, and the ones who are good at math have a huge financial incentive to do something other than teach math to the next generation" and "the population has a sufficiently large fraction of people good at math, so some of them become teachers, and make a comparable fraction of the next generation good at math". If that is indeed the case, this reduces to a chicken-and-egg problem how to get from the former equilibrium to the latter, and perhaps some one-time intervention could achieve that, at least locally. (2) Maybe using computers in education can make the math education scale better. For example, if your math teacher sucks, you also have the option of Khan Academy. And tomorrow, you could have something even better. So you might need fewer good math teachers to make the next generation math-savvy.
I can only watch the American situation from far far away, so I miss all the nuance. If there is a source that explains, step by step, how the good ideas were gradually turned into bad ones, I would like to see it. Right now, I am kinda familiar with the beginning and the end, but don't see the steps between; so I can't speculate on how they could be avoided. (The sources critical of the end result don't say anything about good beginnings; they assume it was all hopeless from the start.)
My very vague model is that there is a thing that often happens with ideas, that if you invent something, someone else will make a strawman of it and insist that it is an improvement of your ideas, the fully developed version thereof. Metaphorically, if you say "I believe that bread-and-butter tastes better than bread alone", inevitably someone else will yell "what he's actually trying to say is that butter alone tastes the best"; because the person interprets your statement as a movement in the direction of less bread and more butter, and is happy to run even further in that direction, believing that this is the true essence of what you wanted to say.
As an example, someone says "a map is not the territory", and someone else inevitably completes this as "what he's actually saying is that there are only maps all the way down". (If "maps and territory" is better than "territory which I perceive directly", then even more maps and even less territory must be even better.) And if someone says "instead of cramming as many facts as possible into children's brains, could you please slow down and let them think about it for a moment, then maybe they could understand it better", inevitably someone else will complete this as "what he's actually saying is that we should never teach the kids anything, because true understanding only comes from reinventing everything from scratch with zero help". (Which sounds to me like the difference between the constructivism-as-I-know-it and constructivism-as-practiced-during-math-wars.)
If this happens to be true and inevitable, how do you prevent the shift in that direction? Maybe by not talking about constructivism in general (and thus inviting the idiots to the debate), but by making a specific textbook and saying "use this". If people use your specific textbook, their options to misinterpret your words are more limited than if they merely use (their interpretation of) your philosophy.
So what exactly happened in USA? Were there at first some good constructivist textbooks which were later replaced by bad textbooks? (Could we still find and use those good textbooks?) Or did all constructivist textbooks suck, because the people who understood the good ideas never wrote the textbooks themselves? (In which case, would it help if I gave you a good textbook?)
Yet another idea is that if you can't fix the school system, you could try to develop an alternative system outside the school. Khan Academy is a step in this direction -- even if all schools are hopelessly bad, and all math teachers hopelessly stupid, a motivated conscientious child with internet connection still has an access to good math education. Maybe we can go even further in this direction, such as organizing local meetups of Khan Academy users (so now the kids will learn math because that is what their friends do), providing places for poor kids where they would have a free access to computers that only run Khan Academy and educational programs, etc. It won't be perfect, but I am curious how far we could go.
Replies from: Valentine↑ comment by Valentine · 2022-08-14T04:33:22.261Z · LW(p) · GW(p)
I like your thoughts. The short answer is a combo of "I don't know" and "I have some trouble remembering, but it was something like the following."
As I recall, there were something like five factions in the USA math wars:
- Mathematicians
- Math education researchers
- Politicians
- Teachers
- Parents
Mathematicians were sure that math ed research was pointless, because mathematicians didn't (and still mostly don't) understand survivorship bias. Last I checked, mathematicians' education theory still amounts to "State the math accurately, and those who can get it will, and those who can't won't. Some variation might help a little, but everyone has an innate math ceiling above which they just cannot go." You'll find some more optimism in some mathematicians, but the institution as a whole takes this kind of tone best as I can tell.
Math ed researchers actually try to do the empirical work of sorting out good education methods. They also are prone to ideological capture and fads, and are utter garbage at treating complex social systems as real. Many of them seem to think that things like "Have 1-on-1 weekly on-the-job training for every teacher in elementary school for two years" is a viable solution to the challenges of education, as though political money and give-a-fucks will just flow from academic peer-reviewed papers the way academic grants do.
Politicians just want to get reelected. Their job is to polish Good Guy™ badges. When it comes to the education system, their target audience is the parents. This results in them trying to show the parents that the politicians are Taking Things Seriously and are consulting the Correct Experts. Part of their job is to discern whom the parents will judge as the Correct Experts. (One of the fronts of the math wars was exactly this point. Now mathematicians are considered much better Experts on how to teach math than are math ed researchers — unless something massive has changed in the last decade while I've been out of this loop.)
Teachers struggle on the front lines, pulled between school administrators above them, parental demands around them, and the children's needs and behavior in front of them. Their job is to juggle all of that while looking professional and keeping everyone liking them. If they don't like their job, the illusion is that they can just be replaced. In practice this puts a powerful filter on the personality profile for teachers: They almost always care deeply about the kids, get meaning out of enormous self-sacrifice, and if they're aiming to teach at an elementary or middle school level are mildly to severely math-phobic. (I don't know why the math phobia is so systematically true, but anecdotally it very much is, and it's really not subtle.) The one point of power they have in terms of the math wars is that whatever gets taught, they have to teach. So if they can't or somehow muster the power to refuse, reform doesn't happen. Basically weak veto power.
Parents are… well, dumb. I'm not sure how else to say it. They don't see the system, they don't see the above constraints, they mostly don't understand math, they definitely don't understand education, they don't know that they don't know these things, and they do not care that they don't know. For the most part in practice they seem to be highly entitled. (And yes, of course, I'm overgeneralizing. I'm describing the collective of "parents" as a faction of the math wars, not a fact about each parent whose kid takes math classes in public schools.) Teachers are often quite skilled at forming good connections with parents — but if teachers start assigning kids homework that the parents can't understand, it often creates a backlash. E.g., one reform effort experimented with removing drilling times tables, and parents were in revolt about this. Why? Because they learned via times tables, so why wasn't that good enough for their kids?? This often causes parents put pressure on school administrators, who are something like intermediaries between politicians and teachers.
If you spend a few minutes thinking about these constraints, you might see it.
But let me answer your question more directly:
So what exactly happened in USA? Were there at first some good constructivist textbooks which were later replaced by bad textbooks? (Could we still find and use those good textbooks?) Or did all constructivist textbooks suck, because the people who understood the good ideas never wrote the textbooks themselves? (In which case, would it help if I gave you a good textbook?)
As far as I know, I've pointed you toward the only good textbook in English.
And sadly, because of how textbook distribution is some kind of corporate oligarchic monstrosity, and due to entrenched habits of teaching that are incompatible with deeper learning principles, I don't think handing the USA a better textbook would help the public school system whatsoever.
Some history:
The first wave of USA constructivism — the "new math" back in the 1960s — really was doomed from the beginning. That came from mathematicians reasoning from first principles, not from math ed researchers examining how people learn. There was actually a lot of optimism back then on mathematicians' part in terms of what kids might be able to learn.
But in their ivory tower brilliance, they figured it made sense to (for instance) teach set theory before teaching arithmetic. Why? Because that's how the math was constructed. (Hence "constructivism". Not the same meaning as Piaget-inspired work… but that's what happens when some mathematicians decide they've understood your psychological models and don't bother checking due to being so used to being the smartest people in the room.)
Of course, that just flopped. Teachers couldn't teach it, students had no damn clue what was going on, and eventually teachers kind of gave up and went back to drill-and-practice methods despite the push from school administrators. The kids had to be able to do at least some math, you see!
The new "new math" of the 1990s was more based on actual Piagetian constructivism. This was when math ed researchers got more recognition as the Correct Experts, in part because their ideological alignment was more compatible with the social and political milieu. (It's hard to make math postmodern, but it's easy to make education theories postmodern. It's actually hard to avoid these days.) So the stuff like Cognitively Guided Instruction (from the 1970s!) finally got a little breathing room and started touching classrooms.
…except it wasn't really CGI.
What happened was, some CGI researchers managed to impress some politicians, who put pressure on school administrators, who informed the teachers. CGI doesn't work without in-depth support for teachers to transform how they're teaching. (E.g., they've got to learn how to do real-time PCK-seeking and to prioritize doing that over informing their students about how to get the answer.) But that's expensive, and politicians believed (probably correctly) that parents wouldn't appreciate or approve that much of a jump in expense for education. (After all, how hard is it really to teach kids how to calculate things, right?)
So instead of doing actual CGI, schools started pushing rearrangements of the content of math courses in ways that looked kind of like what one might see in a CGI classroom.
The thing to understand here is, CGI is mostly inquiry-based. You come from such a rich foundation of PCK that you know what problems to present to your students to guide them to notice and sometimes reinvent the mathematical tools they need for their next stage of mathematical growth. If they get stuck, you either have the PCK to know what hint to give them, or you seek PCK in real time to see why they're stuck and what hint will actually help them think instead of just get the right answer. This doesn't always follow the linear progression of topics that's normal in a lecture-based math course, especially as conversation in the classroom meanders student attention and interest into a different direction.
So… politicians who are eager to show off to parents what Good Guys they are started pushing schools to Goodhart on following the Correct Experts' advice, which caused absurd textbooks to get produced that basically stirred all the math topics together into a kind of incoherent mess, and the teachers were dropped into a situation where they were supposed to "teach without telling" (because if you have really rich PCK and PCK-seeking skill you in fact don't need to tell so much — pure Goodharting).
When that failed abysmally, math ed researchers were dethroned as no longer being the Correct Experts. Mathematicians lauded their "I didn't encounter survivorship bias, and neither did my fellow math professors, so clearly it doesn't exist and math skill is innate and therefore math ed innovations are pointless" theory of education, and because parents are morons who forgot that all their math teachers were horrible at teaching math, they just went along with the obviously stupid argument that experts in a subject know best how to teach that subject.
Not that I have impassioned opinions about all this or anything.
This is something like a long-winded way of saying "We've never really tried Communism PCK-driven math." But that's not entirely fair. It's more like, our best effort at it was utterly shit, and all the systematic pieces are still in place to basically guarantee that every other effort at spreading any math ed reform through the school system will run into basically the same problem.
No one is the adult taking responsibility for the whole system. And no one can.
(This is a microcosm of what I see as the core problem behind AI risk too, by the way. Not just in education. It's the "No adults in the room" problem. AFAICT, the three options for AI are (1) we build FAI before uFAI comes online, (2) we create Actual Adults, or (3) we all die. I'm betting (2) is easier than (1) and might even be a functional prerequisite for (1), so it's where I'm pumping all my skill points.)
Yet another idea is that if you can't fix the school system, you could try to develop an alternative system outside the school.
I think this is the only hope.
I hope to have kids one day. If I do, I will move mountains and seas to make sure they're educated at home.
The only benefit of public schools anymore, from what I can tell, is that very wise and patient parents can use it to support their children in mastering Defense Against the Dark Arts.
Well, that and getting to play with other kids. Which is still pretty cool.
Replies from: Viliam↑ comment by Viliam · 2022-08-14T10:39:44.350Z · LW(p) · GW(p)
mathematicians didn't (and still mostly don't) understand survivorship bias.
Yes. To use the terms from the article, mathematicians have the content knowledge, but most of them miss the pedagogical knowledge. Also, a frequent objection to constructivist education of math in Czechia today is some high-status mathematician saying "I didn't need this at school, so what's the point?" And I feel like: "Dude, this is not about the few lucky people like you and me who got it (often because we had some kind of math education outside the school system, e.g. from parents) but about the 90% of kids who don't get math and then hate it."
Cannot comment on math ed researchers; haven't met any of them in person, other than the group of activists that promote the constructivist-math-as-I-know-it, which of course is an extremely biased sample.
Politicians, yeah. One Czech member of parliament keeps saying things like "experimenting on kids is dangerous" and "constructivism causes kids to have mental disorders"; and then the next day he goes "constructivism actually says nothing new, it's merely a summary of things that all good teachers already know". (Does he even realize that his opinions, taken together, imply that good teachers cause mental disorders?) His qualifications? He used to be a sales manager, and now is a politician... so the newspapers are interested in whatever he says, and then the (Czech) Wikipedia page quotes him in the "criticism" section, so the people who make their research by reading Wikipedia (yes, we were all told we shouldn't, but...) can hear both sides of the controversy.
E.g., one reform effort experimented with removing drilling times tables, and parents were in revolt about this. Why? Because they learned via times tables, so why wasn't that good enough for their kids??
I'm going to nitpick here. There is a difference between learning and practice. In my opinion, one should learn multiplication without the tables (by repeated addition), then explore it (e.g. notice that "10n" is just appending a zero, "9n" can be calculated as "10n - n", multiplication is commutative, etc.), but at some moment after the understanding is solid (maybe a year later, not sure) the kids should practice until they memorize it. The "tables" are merely a tool; one might use a spaced repetition software instead or whatever else, I don't care; the important part is that at some moment you hear "8×9" and respond "72" by instinct, otherwise you will have a problem focusing on complex tasks that require "8×9" somewhere in the middle. Put the understand first, sure. Removing the practice entirely is taking it ad absurdum.
Also, let's empathise with the parents a little. They don't get the math, because of how they were taught decades ago. Yet, they will have to answer their kids' questions at home. What if the child gets sick and misses a week or two at school? Tutoring may be too expensive for some. The problem is real, we can't just dismiss it. (A simple solution that took me 5 seconds to think of, would be to make a YouTube channel for each reform, containing videos that explain all the changed parts: the motivation, and how to teach it. The channel would be visibly mentioned in the textbook.)
I fully agree about the set theory; what kids do at elementary school has almost nothing in common with the set theory qua set theory (ZFC, etc.), they were just happy to use a high-status keyword.
The thing to understand here is, CGI is mostly inquiry-based. You come from such a rich foundation of PCK that you know what problems to present to your students to guide them to notice and sometimes reinvent the mathematical tools they need for their next stage of mathematical growth. If they get stuck, you either have the PCK to know what hint to give them, or you seek PCK in real time to see why they're stuck and what hint will actually help them think instead of just get the right answer. This doesn't always follow the linear progression of topics that's normal in a lecture-based math course, especially as conversation in the classroom meanders student attention and interest into a different direction.
Haven't read the textbook yet, but my first impression is that this is a weakness (which according to your story turned out to be fatal). Realistically, teachers need a textbook with a linear progression of topics, with the right problems already provided for them. It's great if they can improvise, but they shouldn't be required to, if the textbook can do this for them. "The method needs to work for average humans" is a sensible constraint.
I hope to have kids one day. If I do, I will move mountains and seas to make sure they're educated at home. The only benefit of public schools anymore, from what I can tell, is that very wise and patient parents can use it to support their children in mastering Defense Against the Dark Arts. Well, that and getting to play with other kids. Which is still pretty cool.
Actually, homeschooling is even better for "playing with other kids"; you just need to have other non-crazy homeschooling families in your neighborhood. Then the kids can play all day long (and also get huge discounts at various private playgrounds which are mostly empty in the morning).
My daughter just finished her first grade. So far, she is quite happy at school, because everything is super easy for her... because she already knew it before she went there. Well, if she is happy, then I am happy, too. No need to homeschool now. But of course, this may change later.
*
Now, one thing that is missing from your story, and which seems very relevant to me. The Wikipedia page on constructivism mentions something called "radical constructivism", which allegedly "rejects an objective reality independent of human perception or reason" and "looks to break with the conception of knowledge as a correspondence between a knower's understanding of their experience and the world beyond that experience". They claim to draw on the work of Piaget, but also call his version of constructivism "trivial".
Wikipedia says they were "influential in educational research and the philosophy of science". Their online journal is called "Constructivist Foundations".
This seems to contradict your framing, which -- if I may simplify it so -- says that the theory was good, and everyone tried to be a good Piagetian at first, but then... arrogant mathematicians, incompetent teachers, limited budgets, Goodharting.
But this information from Wikipedia suggests that the bad (from my perspective, anti-rational) ideas were already out there, and presented themselves as the true constructivism (as opposed to silly "trivial" Piaget who naively believed that there is such a thing as reality, ha ha). I admit I have never heard of them before, but Wikipedia says that they were influential, and... some stupid ideas used in the math-wars textbooks actually make sense from their perspective. Like, what is the point of actually teaching proper math, if even the reality itself is not real... just let the kids do whatever comes to their minds, and signal that we are more constructivist than Piaget by carefully avoiding anything that might resemble actual teaching.
So to me it seems like the teachers / politicians / school administrators were told to do "constructivism", but then there came two groups of people claiming to be experts, who gave them completely contradictory advice on what "constructivism" actually is. And the bad guys had greater political clout, or maybe just their ideas seemed easier and cheaper to implement, so most people went with their version... and then it turned out that "constructivism" is a bad idea.
(BTW I didn't "×-vote" your comment. Saying it explicitly, because now it's just the two of us debating.)
Replies from: Valentine↑ comment by Valentine · 2022-08-15T02:20:16.855Z · LW(p) · GW(p)
I'm going to nitpick here.
Cool. Have fun.
but at some moment after the understanding is solid (maybe a year later, not sure) the kids should practice until they memorize it.
I totally disagree. This alienates kids from their developing inner agency. "You'll now learn this because we said so." As opposed to learning what they need because they can see & feel their own need for it.
Also, let's empathise with the parents a little.
Obviously.
I'm not going to filter my speech to be appropriate across all dimensions. I don't regret what I said or how I said it.
But obviously I agree with you.
(I mean, it's kind of the main point of the PCK stuff!)
Realistically, teachers need a textbook with a linear progression of topics, with the right problems already provided for them.
Only if you're teaching computation classes instead of mathematics.
Notice this isn't an issue in English literature, or music.
But I agree that given the current bonkers goals — both overt and implicit — for math classes, this kind of linearity is key.
I just also think those goals are bollocks and in some cases downright nasty.
Now, one thing that is missing from your story, and which seems very relevant to me. The Wikipedia page on constructivism mentions something called "radical constructivism"…
Oh jeez. I honestly just forgot about those guys.
The heyday of radical constructivism was before my time. But my impression was that it was mostly philosophy that math ed researchers used to confused each other. Kind of like how modern art is some bizarre combo of oneupmanship amongst artists plus a front for tax evasion. Radical constructivism was part of the wave of postmodern philosophy justifying grants for all kinds of weird research projects. It offered context for endless intelligent-sounding debate.
It had incoherent suggestions about what to do for education. It was kind of the behaviorism of education theory. It sounded technically right and quite compelling if you didn't look too closely. Once you started trying to figure out what to actually do with it though… :-P
But I don't recall it claiming there's no objective reality. I remember it rejecting the ability to know about any objective reality, so talking about it directly is a kind of absurdity in their view. But like all postmodern philosophies, it runs into a basic paradox: Is that claim absolutely true, meaning you can access some parts of objective reality, thereby invalidating the point? Or is it only sometimes true, which also implies there are some objective truths you can access somewhere sometimes?
(I could totally believe some radical constructivists thought about this and tried to get around it by claiming there's no objective reality. This is exactly the same postmodern move as when radical social relativism insists that the laws of physics are just a bunch of ideas imposed by the racist patriarchy to support the established social structures of power. It tries to sidestep the logical challenge by shattering the mind's ability to follow reason at all.)
I don't recall the radical constructivists having an oversized effect on the math wars. They affected how math ed researchers thought and talked, but roughly the kind of way behaviorism affected how psychologists thought. I think it'd be pretty weird to list behaviorism as an influence on the math wars (even though it actually did play a pretty big role for context-setting in decades prior).
But hey, I could be mistaken. Maybe the postmodern philosophers of math ed research shoved themselves to the front and started babbling gibberish at teachers until everything fell apart. And maybe if we could own that and stop it from happening again, we could get sane policies into schools.
I'm not holding my breath for that one though.
So to me it seems like the teachers / politicians / school administrators were told to do "constructivism", but then there came two groups of people claiming to be experts…
I honestly wasn't aware that the word "constructivism" was a hot topic. I wouldn't have thought there'd be much point in talking about "constructivism" explicitly when proposing education policy. That'd be like a plumber trying to explain viscosity and Bernoulli's principle to house owners.
But given that math ed researchers did, I think the cause is messier than what you're describing.
Basically anyone who was inspired by Piaget called their thing "constructivism". There are lots of variations. The "radical" vs. "trivial" split isn't the only one. Like I hinted at before, mathematicians created a "constructivist" approach based on constructing (!) mathematical objects and operations in the minds of children following the same rigorous pathway the mathematicians used to derive their tools from ZMF.
I faintly remember encountering at least one paper that claimed that it was constructivist because it was looking at levels of development. Because Piaget inspired the idea of levels of development, you see, and that's based on constructing understanding, and so with "Piaget" and "construction" in the same idea cluster surely that makes you a constructivist, right?
I remember one of my teachers was basically a Piaget scholar and encouraged us to refer to Piagetian Constructivism, and to dissect various papers for where their implicit philosophy or explicit methodology differed from key points of Piaget's approach (as she interpreted it). Frankly there weren't many papers that survived that kind of analysis.
It's possible the word "constructivism" also underwent something kind of like "nanotechnology" did: If funding appeared for "constructivist" approaches, then anyone who wanted funding could get it by finding a way to describe their pet project as "constructivist", thus diluting the term.
For the most part I never want to use that word in educational contexts. It's a messy ball of confusion just begging for pointless arguments. Some of the reason I honed in on Gears [LW · GW] is precisely because it promises to cut through… gosh, I don't know, but my gut claims it's something like 2/3 of the babbling nonsense that shows up in education spheres once someone brings up "constructivism" (or "understanding").
(BTW I didn't "×-vote" your comment. Saying it explicitly, because now it's just the two of us debating.)
Okay. I wasn't worried about it. But thanks for mentioning.
And… honestly I really dislike framing this as a debate. I guess it is now? I wasn't debating you before this. Just letting you know my impressions. I thought we were just comparing notes.
Replies from: Viliam↑ comment by Viliam · 2022-08-15T22:49:50.799Z · LW(p) · GW(p)
This alienates kids from their developing inner agency.
If you have 20-30 kids in the classroom, and an externally given list of goals to achieve, this puts a constraint on agency.
Also, some kids have an aversion against practicing stuff. Often the smart ones -- they sometimes identify as "intelligent", and it is a part of their self-image that they are supposed to learn things by mere understanding; anything that resembles work means for them that they have failed, because they were supposed to learn it without working hard. I knew very smart kids who just couldn't learn a foreign language, because the idea of "memorizing by repetition" horrified them, and... nothing else worked. Their less smart classmates already learned the languages by practicing.
There are schools that try to maximize agency. And there is also unschooling, with the same goal. I suspect that kids who learn this way, will usually miss all the stuff that has very long inferential distances -- because to get there, you need to walk a long way, and not each step is intrinsically exciting. (Reminds me of people in Mensa who can spend endless hours debating relativity or quantum physics, but never find time to read a textbook and fix their elementary misconceptions.)
So... yeah, I would seek some compromise between agency and knowledge. I might be convinced otherwise by some research that would show that average unschooled kids are more successful along some dimension than average school kids. It seems to me that unschooling is more enjoyable, but does not typically translate into following one's own educational goals or projects.
Basically anyone who was inspired by Piaget called their thing "constructivism".
If the label is diluted to uselessness, we need some new way to talk about the useful parts. One possibility is to just list the useful parts individually, without having an umbrella term. Not sure how well this would work... I guess I would need to compile the list first.
Replies from: Valentine↑ comment by Valentine · 2022-08-16T00:17:33.399Z · LW(p) · GW(p)
So... yeah, I would seek some compromise between agency and knowledge.
To each their own. I don't value any knowledge so dearly that it's worth sacrificing chunks of children's agency to make sure they have said knowledge. The willingness to make that trade is key to the lifecycle of that which would create unFriendly AI [LW · GW].
If the label is diluted to uselessness, we need some new way to talk about the useful parts. One possibility is to just list the useful parts individually, without having an umbrella term.
Well… if you look above, you'll see that you were the one who introduced the label!
As I said, I gave up using "constructivism" to describe things in this space years ago.
comment by Leon Lang (leon-lang) · 2023-01-12T19:20:22.383Z · LW(p) · GW(p)
Summary:
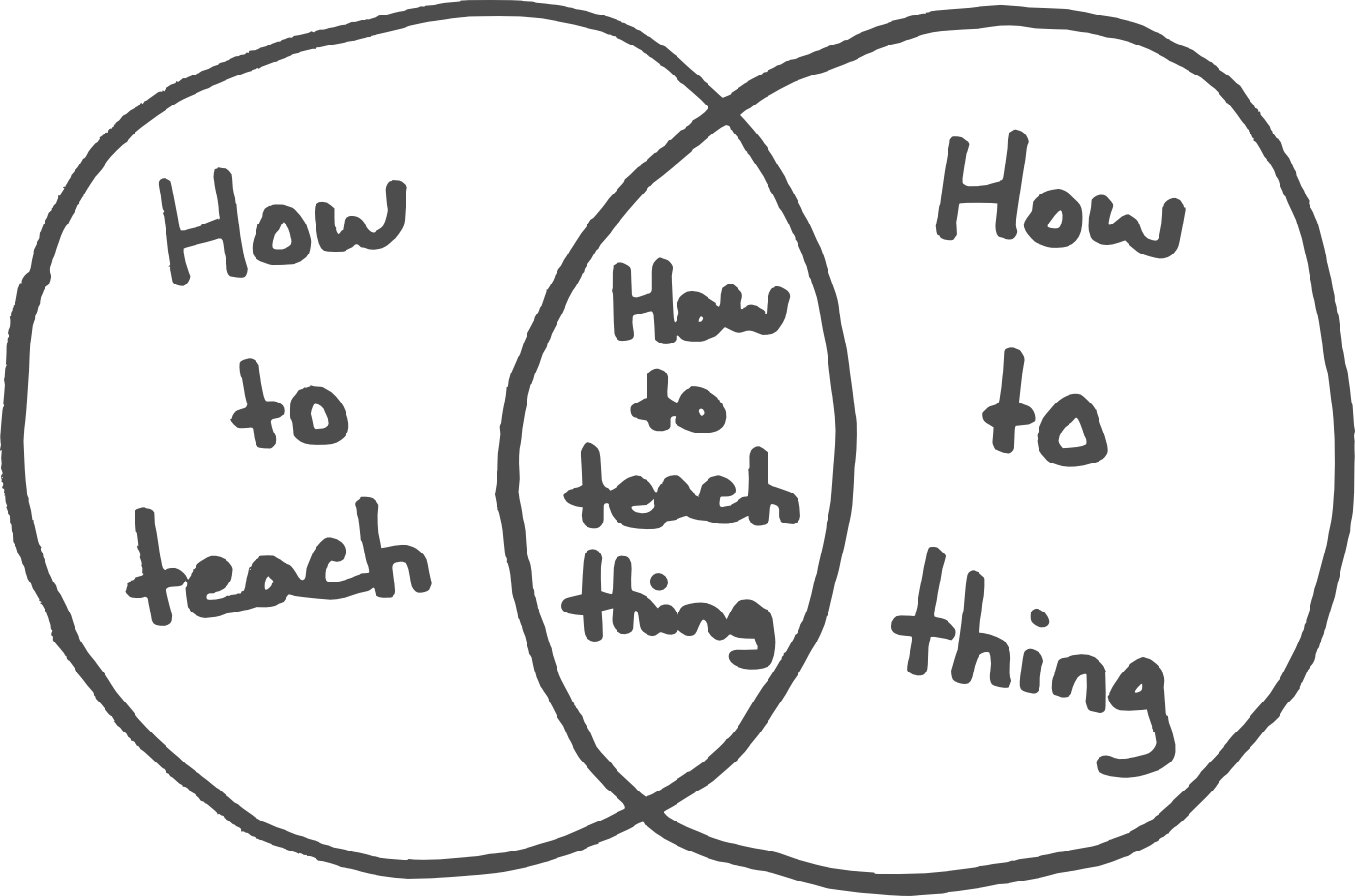
- Pedagogical Knowledge (PK): How to teach
- Content Knowledge (CK): How to thing
- Pedagogical Content Knowledge (PCK): How to teach thing
- This includes: What’s fundamental, what requires other prerequisites?
- Look out for that when learning a new topic
- Example: Partitive division vs. quotitive division: equal sharing vs. repeated subtraction example.
- PCK in this context is awareness of the fact that there are two division operations
- Strategies in teaching:
- Ask: “What’s it like for a learner to encounter this material?”
- Be curious about thinking processes (instead of pointing out errors)
- This tends to encourage reflection instead of pleasing
- Apply these strategies when teaching yourself
- This includes: What’s fundamental, what requires other prerequisites?