Practicing Bayesian Epistemology with "Two Boys" Probability Puzzles

post by Liron · 2025-01-02T04:42:20.362Z · LW · GW · 13 comments

Contents

  The Puzzles
  The Solution
    Now the tricky part…
    Now the confusing part...
  More Bayesian analysis
None
13 comments

The Puzzles

There's a simple Monty Hall adjacent probability puzzle that goes like this:

Puzzle 1
I have two children, at least one of whom is a boy. What is the probability that both children are boys?

A more complex variation recently went viral on Twitter:

Puzzle 2
I have two children, (at least) one of whom is a boy born on a Tuesday — what is the probability that both children are boys?

Then Isaac King [LW · GW] tweeted an even more complex variation:

Puzzle 3
I have two children, at least one of whom is a boy born on a day that I'll tell you in 5 minutes. What is the chance that both are boys, and what will the chance be after I tell you the day?

All three versions are fun and worth a try if you want to learn and practice Bayesian reasoning.

Personally, I found Isaac's version MUCH harder than the others. I was surprised how hard it stumped me since I had a pretty easy time with the first two (LessWrong trained me well). As I stared at it for longer than I want to admit, the gears of my brain kept jamming. I couldn't see a coherent non-paradoxical story for what the Bayesian updates should look like.

I recommend giving Puzzle 3 a try before reading my solution.

By the way, I tested it on GPT-o1 and Claude 3.5 Sonnet and they only give incorrectly-reasoned wrong answers. It'll be interesting to see if o3 can do better.

 

The Solution

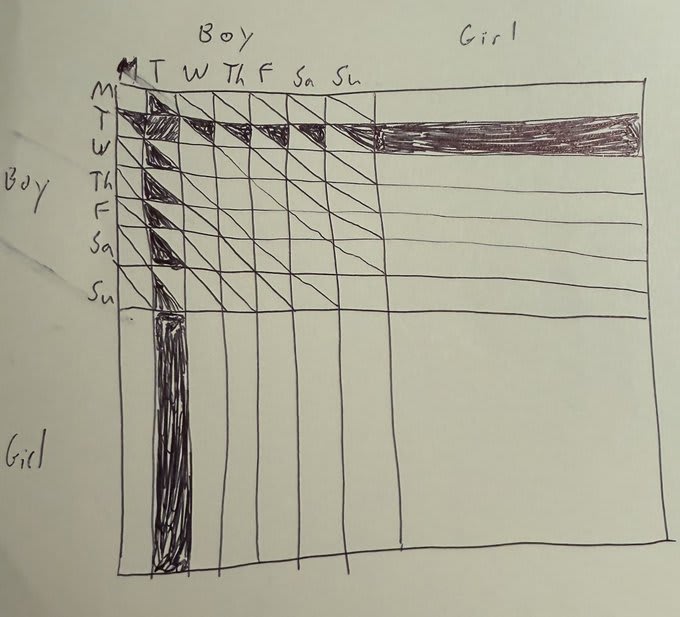
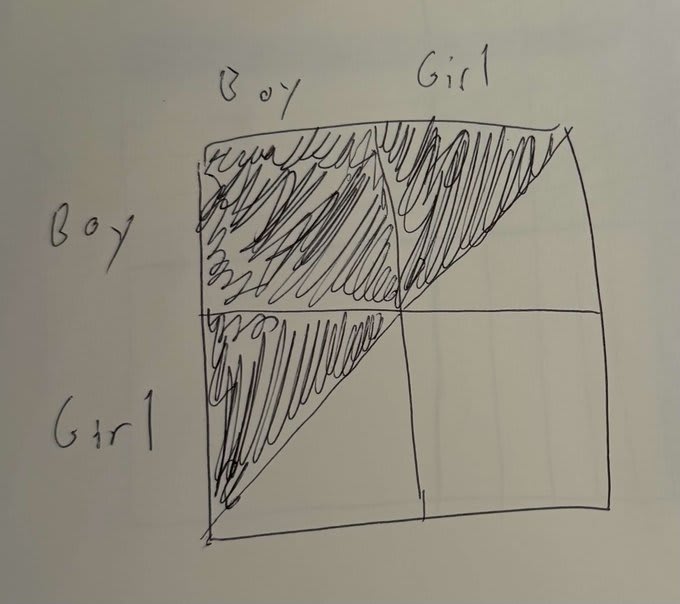
First, when we hear the "I have two children, at least one of whom is a boy" part, we set the probability of two boys to 1/3 because the possibilities {(boy, girl), (girl, boy), (boy, boy)} are a-priori equally likely and we haven't had a reason to update their relative likelihoods.

Then when we hear "I'll tell you the day that at least one was born on", we don't need to update the three relative likelihoods because it's a statement we were equally likely to hear in all three possible worlds.

Now the tricky part…

When we subsequently hear a particular day, e.g. "Friday", how should we update the relative probabilities?

It seems like we shouldn't update, because hearing any weekday was a-priori equally likely to hear, and if hearing any weekday was going to update us in any particular direction, why couldn't we have just made that update before we heard the particular day?

In other words, why wouldn't we pretend like the parent mumbled the day and we couldn't make out the word, but update anyway, since it's going to be the same update regardless of which day he says?

Indeed, the correct answer is going to be that hearing the particular day doesn't trigger an update. The correct answer to the puzzle is the intuitive one…

ANSWER:
The probability that both children are boys stays 1/3 the whole time

Now the confusing part...

The confusing part is that when we compare the answer of "don't update on the birth day of the week information" to Puzzle 2's answer, it seems inconsistent or paradoxical.

Puzzle 2 asks:

I have two children, (at least) one of whom is a boy born on a Tuesday - what is the probability that both children are boys?

Puzzle 2's answer is larger than 1/3; the (boy, boy) world gets more likelihood for being more consistent with the evidence of having at least one boy born on a Tuesday: 13/49 for (boy, boy), compared to 1/7 (i.e. 7/49) each for (boy, girl) and (girl, boy).  

The posterior probability of the (boy, boy) world is thus 13 / (13 + 7 + 7) = 13/27.

But why doesn't this same visualization and this same calculation apply to the new puzzle (Isaac's twist)? If we hear "Tuesday" in the new puzzle, shouldn't we similarly update our probability of two boys from 1/3 to 13/27 ???

IMO this is quite a juicy apparent paradox, and gets to the heart of why most people underestimate Bayesian epistemology. People don't realize how subtle and powerful it is when wielded by a trained practitioner.

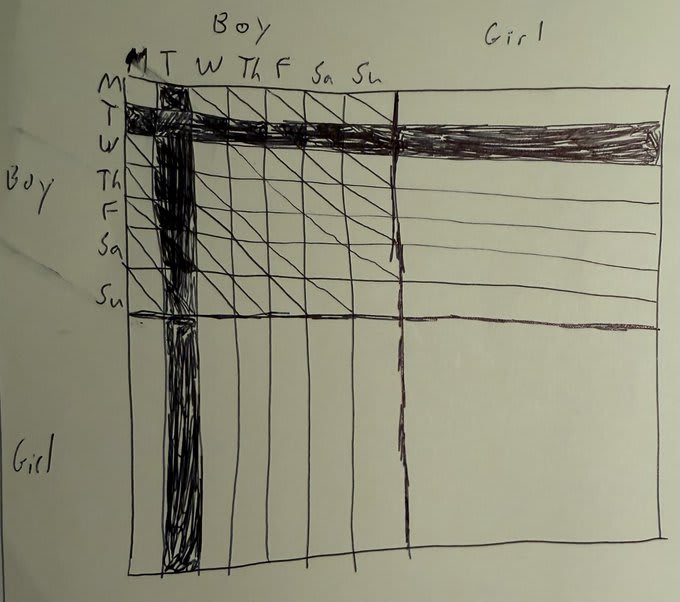
Let's think about the scenarios that make the parent in the new puzzle say "Tuesday":

1. We're in the (boy, girl) world and the boy is born on Tuesday
2. We're in the (girl, boy) world and the boy is born on Tuesday
3. We're in the (boy, boy) world and only the older boy is born on Tuesday
4. We're in the (boy, boy) world and only the younger boy is born on Tuesday
5. We're in the (boy, boy) world and both boys are born on Tuesday

So far, the diagram above that we used for the original puzzle still looks like it models the situation…

The key is to realize that in scenarios #3 and #4, we don't always hear the parent say "Tuesday". Half the time, we hear the parent say the name of the weekday that the other boy was born on!

In the diagram below, the shading of squares in the (boy, boy) quadrant doesn't just represent the fraction of scenarios wherein the parent could say "Tuesday", it represents the probabilistically weighted fraction of scenarios wherein the parent does say "Tuesday":  

The shaded half-squares conveniently make the (boy, boy) quadrant's shaded part add up to 1/49 + 12(0.5/49) = 7/49, just like the (boy, girl) and the (girl, girl) quadrants' masses do, allowing us to rationally answer the puzzle with our a-priori probability of 1/3.

QED

 

More Bayesian analysis

Now that we're over the hump — problem solved, paradox resolved — let's see what insights we can glean about Bayesian reasoning.

Consider the humble Puzzle 1:

I have two children, at least one of whom is a boy. What is the probability that both children are boys?

I explained above that it's 1/3 because the possibilities {(boy, girl), (girl, boy), (boy, boy)} are equally likely.

But in fact, the parents I know are much more likely to ask you that question in the first place in worlds where they have two boys. Then they'll smirk and say "wanna bet?" and you'll lose the bet.

But if you don't see that coming, don't blame Bayesian reasoning; blame your own lack of mastery of Bayesian reasoning. If the naive calculation gives you 1:2 odds of (boy, boy), but your understanding of parent humor tells you it's 3 times more likely that parents with two boys would spring that puzzle on you, then you should actually be assigning 3:2 odds of (boy, boy), not 1:2.

You might be thinking: Fine, but can't “I have two children, at least one of whom is a boy; what's the probability that both are boys" still be interpreted as a problem of pure math? Why go on a tangent to talk about real-life parents?”

Well, actually the puzzle statement contains a default assumption which — while clear enough — is not at the level of a rock-solid default assumption to accept.

The implied assumption is about how the reality of the parent's kids affects what the parent says to you. Basically:

1. If we're in the (boy, girl), (girl, boy) or (boy, boy) world, then the parent asks you the puzzle.
2. If we're in the (girl, girl) world, then the parent says nothing or asks you a different puzzle, perhaps one about girl children.

But consider an alternative assumption. What if we assume that the relationship between the reality of the parent's kids and the puzzle he gives you is as follows:

1. If we're in the (boy, boy) or (girl, girl) world, then he challenges you with the puzzle about his boy or girl children, respectively.
2. If we're in the (boy, girl) or (girl, boy) world, then he randomly selects which gendered version of the puzzle to challenge you with.

Let's assume the parent operates in this "equal-opportunity gendered puzzle" mode, and now consider what it means when he asks you Puzzle 1:

I have two children, at least one of whom is a boy. What is the probability that both children are boys?

It's still true that {(boy, girl), (girl, boy), (boy, boy)} were a-priori equally likely possibilities. But now you have to consider that half the probability of the (girl, boy) or (boy, girl) worlds flowed into worlds where the parent gives you the girl version of the puzzle, so only half the original probability of those squares flows into the world where you receive the evidence of the parent giving you the particular puzzle that you're hearing.  

Under our new assumption, the answer to the easy puzzle is arguably more intuitive than the result of the original puzzle: The probability that the parent has two boys is 1 / (1 + 0.5 + 0.5) = 1/2, not 1/3.

Ok, but why is the "default assumption" the one that gets you 1/3 as the answer to the easy puzzle? Especially since it's the less intuitive answer (IMO)?

I think it's because any time you hear a piece of information in a math puzzle context, you're supposed to assume that the correct way to calculate a posterior probability is to just count the number of possible world-states that are logically consistent with the puzzle's new object-level proposition. You do this kind of count twice to get probability as a fraction: once for the numerator and once for the denominator. I hear there are some quirky people called "frequentists" who consider the non-Bayesianness of the default interpretation to be a feature, not a bug, of these kinds of puzzles.

When Isaac twisted the puzzle by having the parent send us different pieces of information at different times, he made it impossible to only perform updates on the explicit content of the parent's words, because it's necessary to incorporate what we know (or rather, very reasonably assume) about how the parent's future statements are probabilistically related to the underlying facts about their kids.

---

I hope you've gained more appreciation for the power and subtlety of Bayesian epistemology by solving and analyzing these simple-looking puzzles.

13 comments

Comments sorted by top scores.