Productivity as a function of ability in theoretical fields
post by Stefan_Schubert · 2014-01-26T13:16:15.873Z · LW · GW · Legacy · 34 commentsContents
34 comments
I argued in this post that the differences in capability between different researchers are vast (Kaj Sotala provided me with some interesting empirical evidence that backs up this claim). Einstein's contributions to physics or John von Neumann's contributions to mathematics (and a number of other disciplines) are arguably at least hundreds of times greater than that of an average physicist or mathematician.
At the same time, Yudkowsky argues that "in the space of brain designs" the difference between the village idiot and Einstein is tiny. Their brains are extremely similar, with the exception of some "minor genetic tweaks". Hence we get the following picture:


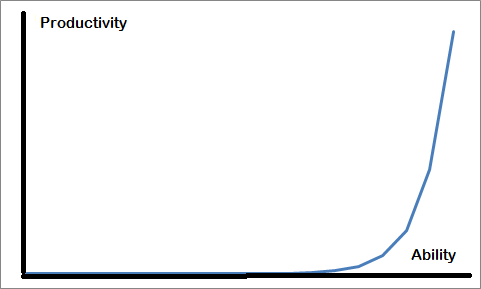
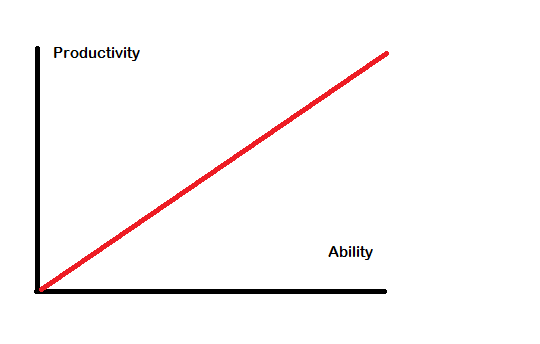
34 comments
Comments sorted by top scores.
comment by satt · 2014-01-26T20:41:06.355Z · LW(p) · GW(p)
A fair part of the abnormal productivity of (for example) Einstein & von Neumann is probably down to when they were born.
To have a hope in hell of being the Greatest Of All Time, a scientist has to work in a scientific culture with (1) a supply of big & solvable open problems to attack, (2) sensible earlier ideas & theories on which to build, and often also (3) good enough technology to collect evidence that might confirm new theories. But it follows that a scientist doesn't have a chance of being the GOAT unless they're born at the right time. If they're born too early they end up like, say, Aristotle, someone impressive and historically important but nonetheless long superseded; if they're born too late, most of the prestigious solvable problems will have been picked off by someone else, and even if the scientist picks one off themselves, they're still trying to stand out in a more crowded field.
If Einstein had been born in 1500, or 1950, he might've been a Galileo or a Copernicus, or a Bekenstein or a Perlmutter, but he wouldn't've been an Einstein.
Of course this can't the whole explanation for why scientists differ so much in productivity, because you still have big differences in productivity even within a cohort of scientists all the same age. Nonetheless, if we want to explain why we're talking about Einstein instead of e.g. John Archibald Wheeler, the fact that Einstein was in the right time and the right place probably has a lot to do with it. While I agree that part of the reason for von Neumann & Einstein's vast productivity is the nonlinearity of the ability-productivity relationship, I think it's worth bearing in mind the right-time-right-place effect as well. (Knowing about that effect also makes Yudkowsky's brain-line diagram less surprising.)
Replies from: EHeller, AnthonyC↑ comment by EHeller · 2014-01-27T02:54:16.842Z · LW(p) · GW(p)
Along these lines, consider how productive all of physics was between 1905-1950 or so. Einstein and Von Neumann were incredibly productive, but they also lived during an incredibly productive time to be a physicist.
Also consider that the way physics is structured now (due to the post 1950s boom and then bust), its not clear either man would even try to be a physicist. Einstein only ever had one paper peer reviewed, and it upset him greatly.
Replies from: None↑ comment by [deleted] · 2014-01-27T10:33:53.216Z · LW(p) · GW(p)
Einstein only ever had one paper peer reviewed, and it upset him greatly.
Well admittedly, one of the things one learns very early in a scientific career is that writing a paper good enough that peer reviewers won't absolutely hate it is a surprisingly high target to hit.
↑ comment by AnthonyC · 2014-01-27T14:03:56.898Z · LW(p) · GW(p)
I'm not sure I would agree with the premise that Aristotle is less important than Einstein. Einstein greatly accelerated several fields of physics. Aristotle has long since been superceded in essentially every field, but his ideas still inform (indirectly) more modern work in logic, ethics, metaphysics, and so on. He was certainly productive, no doubt about that.
Also, there's no finite set of important solvable problems. Today, the available solvable problems may or may not be in physics, but there are plenty on other fields.
Replies from: satt, EGarrett↑ comment by satt · 2014-01-29T23:54:32.186Z · LW(p) · GW(p)
I'm not sure I would agree with the premise that Aristotle is less important than Einstein. [...]
My physics-centric bias is showing! I was comparing Aristotle-as-physicist to Einstein, not comparing Aristotle-as-all-round-smart-guy to Einstein, and I should've consciously realized that's what I was doing.
Also, there's no finite set of important solvable problems. Today, the available solvable problems may or may not be in physics, but there are plenty on other fields.
I wonder how many of those big, solvable problems there are. It's not clear there are any (more) big unifying theories to be had in, say, macroeconomics or sociology. What would it mean to be a 21st century Einstein of one of those fields, I wonder?
Replies from: army1987↑ comment by A1987dM (army1987) · 2014-02-02T13:47:17.708Z · LW(p) · GW(p)
I wonder how many of those big, solvable problems there are. It's not clear there are any (more) big unifying theories to be had in, say, macroeconomics or sociology. What would it mean to be a 21st century Einstein of one of those fields, I wonder?
I'd start by looking at these lists.
comment by alex_zag_al · 2014-01-26T19:13:48.328Z · LW(p) · GW(p)
You're implicitly modeling difficulty as a single number, and ability as another single number that determines the maximum difficulty of the problems you can solve.
However, according to Feynman, you can sometimes solve problems that smarter people can't by having "a different box of tools". The quote:
That book also showed how to differentiate parameters under the integral sign - it's a certain operation. It turns out that's not taught very much in the universities; they don't emphasize it. But I caught on how to use that method, and I used that one damn tool again and again. So because I was self-taught using that book, I had peculiar methods of doing integrals.
The result was, when guys at MIT or Princeton had trouble doing a certain integral, it was because they couldn't do it with the standard methods they had learned in school. If it was contour integration, they would have found it; if it was a simple series expansion, they would have found it. Then I come along and try differentiating under the integral sign, and often it worked. So I got a great reputation for doing integrals, only because my box of tools was different from everybody else's, and they had tried all their tools on it before giving the problem to me.
I don't think this changes the picture very much, though. You just divide the people in the field according to their box of tools. Pick the group with the box of tools that can solve the problem, and your model applies to them.
comment by Kaj_Sotala · 2014-01-27T06:05:17.569Z · LW(p) · GW(p)
So how can they both be true? The answer is, obviously, that they are measuring different things.
When I first saw them, I assumed they were measuring the same thing, but your picture was just zoomed in.
Replies from: shokwave↑ comment by shokwave · 2014-01-27T06:34:32.979Z · LW(p) · GW(p)
Replies from: Stefan_Schubert, Kaj_Sotala↑ comment by Stefan_Schubert · 2014-01-27T12:10:29.727Z · LW(p) · GW(p)
Well not really. I think it's a bit unfair to the average physicist to say that he's closer in intelligence to the village idiot than to Einstein, don't you think...? Hence the average phycisist should be much further to the right on your scale. Thus zooming in rather illustrates what I wanted to say - that productivity increases massively beyond a certain level of ability.
Replies from: army1987, shokwave↑ comment by A1987dM (army1987) · 2014-01-28T06:58:49.669Z · LW(p) · GW(p)
It depends on whether you only count theoretical physicists or also experimental physicists in the average.
↑ comment by shokwave · 2014-01-28T04:22:33.624Z · LW(p) · GW(p)
I think it's a bit unfair to the average physicist to say that he's closer in intelligence to the village idiot than to Einstein
The average physicist's contribution to physics is closer to the village idiot's contribution than to Einstein's, no?
Replies from: satt↑ comment by satt · 2014-10-14T23:06:55.500Z · LW(p) · GW(p)
The average physicist's contribution to physics is closer to the village idiot's contribution than to Einstein's, no?
Depends on whether you use a log scale.
Replies from: James_Miller↑ comment by James_Miller · 2014-10-15T01:10:46.827Z · LW(p) · GW(p)
And if you count teaching as a contribution.
↑ comment by Kaj_Sotala · 2014-01-27T14:56:43.545Z · LW(p) · GW(p)
Exactly.
comment by Gunnar_Zarncke · 2014-01-27T00:13:14.626Z · LW(p) · GW(p)
What you effectively mean is that most people don't reach the edge described very nicely visually here:
http://www.businessinsider.com/the-illustrated-guide-to-a-phd-2012-3
Replies from: Apprentice↑ comment by Apprentice · 2014-01-29T11:07:35.866Z · LW(p) · GW(p)
While I do like that visualization a lot, I think it is misleading in some ways. It is trivial to add to the sum of human knowledge. Go and count the coins in your wallet. I don't know how many are in mine so I'll go and check. Okay, there are 18 coins in my wallet. Now we know something we didn't know before.
"Oh, but that's not knowledge, that's just data - and just one datum at that. By 'knowledge' we mean stuff you can get published in research papers - something containing analysis and requiring insight." Bah, I tell you. You totally could get a coins-in-wallet study peer-reviewed and published. You just have to look in more wallets. Imagine you gathered a sample from 100 people in your city. Take 100 people from another city. Add cities in other countries. Do it all again in a year. Keep doing it for 10 more years. Now you have lots of data. Go and write your paper. It won't require any insight or genius - it will practically write itself. Just present your data in some legible way with nice graphs and you will find someone interested enough to publish it. Maybe not a top journal but definitely a legitimate journal in a relevant field.
The boundary of knowledge isn't hard and doesn't require years until you come up with some "breakthrough". You can just start right away, collecting information no-one currently has.
Or if you'd like to define 'human knowledge' so restrictively that the coins-in-wallet study wouldn't count, then the average PhD won't count either. Look at this zombie. Isn’t it racist and sexist? Yes, it is.
comment by itaibn0 · 2014-01-26T20:10:52.081Z · LW(p) · GW(p)
Consider two models: In one model, the difference in intelligence between Einstein and a village idiot are tiny, but productivity in the sciences is highly sensitive to intelligence in such a way that a small number of slightly more intelligent people have a disproportionate impact. In the other model, the difference between Einstein and a village idiot are vast, and a scientist's impact is roughly proportional to their intelligence. How can you distinguish between these two models? You can't, because they aren't actually different models; they're the same model using different parametrizations for intelligence.
The reason Eliezer Yudkowsky makes these arguments is because he wants to make certain points about what happens when you extrapolate to much higher intelligence. However, you cannot make even qualitative points without understanding how things are parametrized. For example, in general relativity it is possible for points to appear to be singularities under one coordinate system but behave perfectly smoothly in another. Thus I am unsatisfied at how you sweep the issue of how ability is defined under the rug.
Replies from: None↑ comment by [deleted] · 2014-01-27T03:50:40.685Z · LW(p) · GW(p)
Consider a third model in which one in a dozen evenly matched scientists picks the direction and approach that actually leads to results while the rest happen not to find that particular gem.
It sure isn't the only thing, but random chance does play a part.
comment by Kawoomba · 2014-01-26T14:25:18.899Z · LW(p) · GW(p)
Compare:
I argued in this post that the differences in capability between different political leaders are vast. Genghis Khan's conquering of much of the known world or Alexander the Great's conquering of Persia (and a number of other countries) are arguably at least hundreds of times greater than that of an average Angela Merkel or David Cameron.
While capability certainly correlates with impact, the two are not synonymous by any means. Impact is a function of capability and circumstances, often "by luck" chancing upon a subproblem behind which a large discovery is hiding. Just as I wouldn't expect a modern day Genghis Khan to transform Italy into some conquering war machine, so it may be noted that Einstein chanced* upon fields of research that (in retrospect) were treasure troves of breakthrough discoveries.
* John von Neumann's universal genius may be used as a counterexample, but then "capability" may be too one-dimensional of a descriptor, instead maybe there is some quality of "able to sniff out suitable problems to work on", i.e. exceptional capability in task selection over exceptional capability "in general".
Replies from: private_messaging↑ comment by private_messaging · 2014-01-26T16:43:09.536Z · LW(p) · GW(p)
With all due respect to Khan, I don't think he could've done that much better in place of Merkel, and potentially could've done a lot worse if his talents weren't all that great at politics and such.
In place of Hitler, sure Genghis would do a lot better, assuming that he'd somehow rise to power, which is not a given (truly insane speeches wouldn't be something that a sane person is necessarily any good at imitating).
Replies from: Kawoomba↑ comment by Kawoomba · 2014-01-26T17:47:53.903Z · LW(p) · GW(p)
With all due respect to Khan, I don't think he could've done that much better in place of Merkel
Yes. Which is why it wouldn't make much sense comparing their respective "productivity", or "capability", when so much of it depends on the specific circumstances.
Replies from: private_messaging↑ comment by private_messaging · 2014-01-26T18:56:47.872Z · LW(p) · GW(p)
It's more amusing to picture Genghis in place of Hitler. Or vice versa. Hitler in place of Genghis would never even have gotten past the encounter with Tatars, while Genghis in place of Hitler would've been marching German and Polish military towards Moscow after a failed invasion attempt by Stalin, possibly even with US and British help, followed by going all the way into China.
comment by Thrasymachus · 2014-01-27T21:49:02.632Z · LW(p) · GW(p)
I've also been thinking about these issues for a while, and I'm glad you've taken the plunge and posted up some thoughts. I think one model that might be worth thinking of is that physics (and other fields) have a 'tournament game' dynamic, such that one gets significant positional rewards.
So the best physicists have much higher measures of productivity than average physicists not because they are so much smarter, nor because physics happens to be extraordinarily sensitive to differences in physics ability, but because things like publications, patents, fame etc. are strongly positional, and so the very best can get outsize rewards of these things. On this model, if there was never an Einstein (or a Neumann), their discoveries would have been made by others not long after they were actually discovered.
This 'tournament' model seems to do very well at things like sport and the arts. The reason Nadal et al. get so much more money and prestige than an average pro is that you might as well watch the very best (instead of the almost-best) play tennis against each other, and all the reward structures in pro tennis are positional rather than objective. You might want to use the same story in the arts, especially (if you buy Taleb) given how modern technology allows the best performers to leverage their output: why listen to a very good pianist in concert when you can have the world best on CD? Etc. Etc.
Ruling against this model for physics and science would be there is some objective measure of achievement in terms of theory, discovery, etc. But I think on the tournament model still makes a good fit: we should think things like discovery and theory generation have significant positional component (all the kudos goes to the first person there, and so the fractionally better physicists can capture outsize rewards by getting to the answer a couple of weeks before their not-quite-so-good fellows).
Perhaps the best evidence I can think of in support of the tournament model would be that you see very similar 'power law' dynamics in terms of fame or citation count across many fields across a large span of time: this fits a positional model (and, to be fair, a 'big difference in human ability' model), but seems harder to fit on an 'high sensitivity of productivity to ability' model, as it would seem odd that across so many different fields, across so much of their development, the 'sensitivity' area should remain constantly centered on the right-tail of human ability.
Replies from: Stefan_Schubert↑ comment by Stefan_Schubert · 2014-01-28T12:26:05.212Z · LW(p) · GW(p)
Hm, let's see if I understand you correctly. I don't see the difference between our positions on Nadal and art, etc. What I mean by "productive", in Nadal's case, is that simply that many people wants to watch his games. Hence when he plays and his games are broadcast, lots of preferences are satisfied.
Your idea about science is interesting, though. What you're saying is that one can't just look at the number of discoveries that, for instance, von Neumman did, but also at what would have happened if he hadn't made them. Say that von Neumann had tragically died when he was just a child prodigy; what would then have happened to science? Counterfactuals ("what would have happened if x never had happened?") are important for causal explanations ("y happened because of x").
This question is hard to answer, but given that von Neumann made so many discoveries in so many areas, it seems to me unlikely that science wouldn't have proceeded slower in the possible world where he had died as a child.
Anyway, let's assume for the sake of the argument that science really didn't proceed slower in that possible world. Now the next question is: who does all the discoveries that von Neumann did in the actual world in this possible world? If it's a guy as smart as von Neumann, then it seems your argument doesn't defeat my thesis (that beyond a certain level of ability, productivity increases massively). If it's a guy who's not as smart as von Neumann then that would be a problem for my thesis - but it seems to me unlikely that that would have happened.
It might be that the phenomenon you're pointing to means that productivity-as-a-function-of-ability curve is a bit less steep than it seems (though I'm not sure of it), but it seems to me unlikely that it could make it entirely linear.
Your last argument is interesting. Can you give me a link on the similar dynamics across fields?
Replies from: Jiro↑ comment by Jiro · 2014-01-28T19:39:58.267Z · LW(p) · GW(p)
Anyway, let's assume for the sake of the argument that science really didn't proceed slower in that possible world. Now the next question is: who does all the discoveries that von Neumann did in the actual world in this possible world? If it's a guy as smart as von Neumann, then it seems your argument doesn't defeat my thesis (that beyond a certain level of ability, productivity increases massively). If it's a guy who's not as smart as von Neumann then that would be a problem for my thesis - but it seems to me unlikely that that would have happened.
What if it's several guys rather than "a guy"? Then it is possible that each one of them is not as smart as von Neumann and each one makes fewer discoveries than him, but all together they make all of his discoveries.
comment by varsel · 2014-01-27T03:31:18.981Z · LW(p) · GW(p)
(It follows that an artificial intelligence just a tiny bit smarter than Einstein and von Neumann would be as much more productive than them as they are in relation to other mathematician/physicists).
I don't think this necessarily does follow. I think it only follows that such an AI would be much more productive than average in a population of Einsteins.
comment by johnswentworth · 2014-01-26T18:06:44.723Z · LW(p) · GW(p)
Interesting article, but I think there's a more useful conclusion to draw from the idea that major problems in technical fields are precisely those too difficult for most people to solve. While a little extra intelligence can make the difference, it's also likely that an unusual tool set will make the difference. The key is that you have to try something that nobody has tried before. If you want to solve big problems in technical fields, then your biggest relative advantage should come from studying a wide variety of other fields, in search of methods that can generalize to any of the problems you're interested in. On the other side of the equation, you need to know about lots of big problems, so you can try all your methods on a wide variety of problems.
In short, the key to solving hard problems isn't just more intelligence. The key is relative advantage. Intelligence can provide one relative advantage. Rationality and Bayesian probability/statistics can provide another, as Metamed is demonstrating. And of course, we need to find problems where our relative advantages give us the most leverage.
comment by Jonathan Paulson (jpaulson) · 2014-01-28T07:14:29.595Z · LW(p) · GW(p)
Reading this reminded me of Terrence Tao's blog post about how you don't have to be a genius to do math: http://terrytao.wordpress.com/career-advice/does-one-have-to-be-a-genius-to-do-maths/.
I think you are severely oversimplifying "intelligence" and "productivity" into 1-dimensional quantities. In my experience, "genius" (i.e. the insight that solves a problem) is about acquiring a bag of tricks to throw at new problems, and translating your insight into a solution is the result of practice.
Replies from: alex_zag_al, Stefan_Schubert↑ comment by alex_zag_al · 2014-01-30T19:46:51.965Z · LW(p) · GW(p)
I was actually surprised to read back over the post and see that it was about intelligence; in my memory I had translated it into an article about a one-dimensional scale of ability, which I also imagined including, as a critical component, ability to have effective collaborations.
I guess it was the example of Einstein that brought that to mind. Part of what impressed me the most about his career was that, when he was working on general relativity, he collaborated heavily with a mathematician. Marcel Grossman, I think.
There are two separate simplifying assumptions you can make, when modeling this situation. One is assuming intelligence is the most important factor. The other is assuming that ability is one-dimensional. I suppose that this post makes both of them.
↑ comment by Stefan_Schubert · 2014-01-28T11:39:07.826Z · LW(p) · GW(p)
My article is a bit oversimplifying but not because I think intelligence and productivity are "one-dimensional quantities", but rather because you have to simplify in a blog text - texts with endless qualifications become too long and tedious to read. Also I did say that other characteristics besides intelligence also are important.
Thanks for the link; interesting and sympathetic. I'm not saying you have to be a genius to do math: I'm saying some mathematicians are vastly much more productive than others. And I don't see anything in Tao's article that actually contradicts that.
comment by christopherj · 2014-01-31T05:28:26.777Z · LW(p) · GW(p)
There is a much simpler way in which "village idiot" is right next to "Einstein" on the intelligence graph. If you have done any work in computer vision, computer hearing, navigation, or robotics, you might realize that a mouse beats beats decades of work by the smartest people in your field. Most people wouldn't think to brag that they can find their way home from over a block away! If Einstein were missing, lets say, the visual cortex, auditory cortex, nor the chunk of brain required to even read braille, the village idiot would probably pass as smarter. It's only a small portion of the brain that is vaguely dedicated to the sort of thing the IQ test is supposed to measure, plus lots of the specialized bits of the brain can be commandeered to assist (eg the visual cortex for visualizing). Long story short, maybe 80% of Einstein's brain is specialized for tasks any village idiot can do, and his bonus to intelligence is mostly the other ~20% (prefrontal cortex) plus no doubt a better ability to commandeer the other parts into doing more interesting tasks.
To this, add as prerequisites some luck to have the right problems and resources, and the fact that you have to beat the rest of humanity to it, which requires way more work ethic than most humans have (and I get the impression mental work ethic is different than for physical labor). All this makes it really easy to greatly discount the village idiots intelligence and greatly overstate Einstein's intelligence. If there's a mouse on your intelligence chart, Einstein and the village idiot will be nearly touching.
comment by Shmi (shminux) · 2014-01-27T07:22:15.536Z · LW(p) · GW(p)
There hasn't been an Einstein in over 100 years... Are you saying there are no more extra smart people?