What is the alpha in one bit of evidence?
post by J Bostock (Jemist) · 2024-10-22T21:57:09.056Z · LW · GW · 11 commentsThis is a question post.
Contents
Answers 12 SimonM None 11 comments
Recently the whole "if your p(doom) is high, you should short the market" thing has been going around. Let's say the market prices in a 0.1% chance of extinction per century, and you think there's a 50% chance we're dead in the next 25 years. This is about 12 bits of evidence.
- How much profit is it even reasonable to expect someone to make from 1 bit of evidence?
- Is this a constant function of the bits which is calculable like e.g. a Kelly bet? Or does it depend on the market?
- If the latter, does it ever make sense to short things at all based on p(doom)? I assume not, since shorting is generally stupid high-risk for an uninitiated trader (as I understand it).
Consider: everyone else thinks a company share has a 0.1% chance of being worth $10 tomorrow and a 99.9% chance of being worth $0. I think the chance is 50:50. Therefore the stock price is $0.01 today. If I have $100 in the bank, I maximize expected log(money) by spending fully half of my money on it. By my reckoning the maximum log-expected money is $1581, which corresponds to about a 16-fold increase over $100. Which is not 10 bits of alpha!
PS not looking for investment advice here, just looking for maths.
Answers
The log-returns are not linear in the bits. (They aren't even constant for a given level of bits.)
For example, say the market is 1:1, and you have 1 bit of information: you think the odds are 1:2, then Kelly betting you will bet 1/3 of your bankroll and expect to make a ~20% log-return.
Say the market was 1:2, and you had 1 bit of information: you think the odds are 1:4, then Kelly betting, you will bet 1/5 of your bankroll and expect to make a ~27% log-return.
We've already determined that quite different returns can be obtained for the same amount of information.
To get some idea of what's going on, we can plot various scenarios, how much log-returns does the additional bit (or many bits) generate for a fixed market probability. (I've plotted these as two separate charts - one where the market already thinks an event is evens or better,
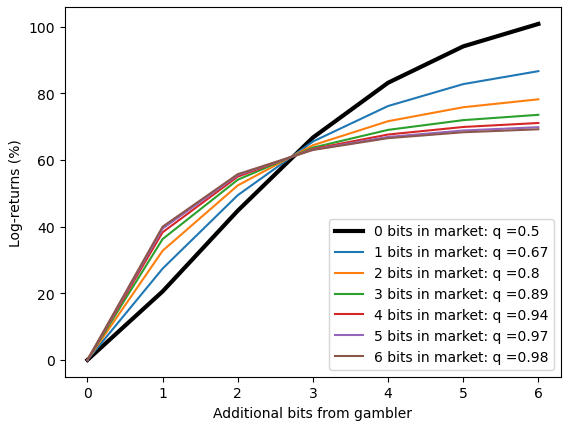
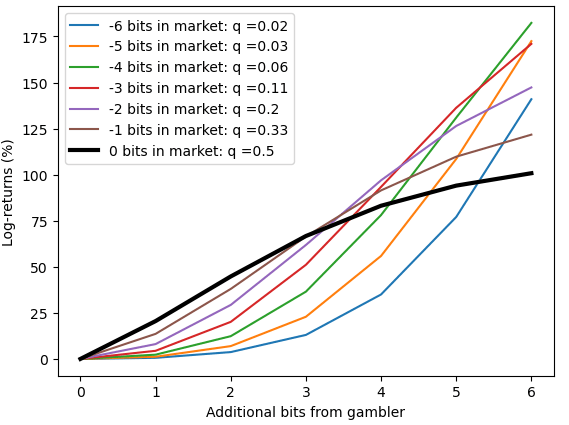
For example, we can spot where the as the market thinks an event is more likely (first chart, 0 bits in the market, 1 bit in the market, etc) and looking at the point where we have 1 bit, we can where we expect to make 20%, then 27% [the examples I worked through first], and more-and-more as the market becomes more confident.
The fact that 1 bit has relatively little value when the market has low probability is also fairly obvious. When there are fairly long odds, 2^(n+1):1 and you think it should be 2^n:1, you will bet approximately(!) 1/2^n, to approximately(!) either double your bankroll or leave it approximately(!) unchanged. So the log-return of these outcomes is capped, but the probability of success keeps declining
There's also a meta-question here which is - why would you expect a strict relationship between bits and return. Ignoring the Kelly framework, we could just look at the expected value of 1 bit of information. The expected value of a market currently priced at q, which should be priced at p is p-q.
When the market has no information (it's 1:1) a single bit is valuable, but when a market has lots of information the marginal bit doesn't move the probabilities as much (and therefore the expected value is smaller)
↑ comment by gwern · 2024-10-26T00:21:37.125Z · LW(p) · GW(p)
This is similar to the answer I got from o1-preview in ChatGPT when I originally asked with OP's post as the text, so that's pleasant to see. (I didn't post anything here because I was unsure and wasn't checking it in enough detail to repost, and so didn't believe in publishing it [LW(p) · GW(p)] without being able to improve it.)
I thought there might be some relationship at first with an appropriate transformation, but when I recalled how Kelly requires both edge and net worth, and the problem of frequency of payoffs, I lost my confidence that there would be any simple elegant relationship beyond a simple 'more information = more returns'. Why indeed would you expect 1 bit of information to be equally valuable for maximizing expected log growth in eg. both a 50:50 shot and a 1,000,000,000:1 shot? Or for a billionaire vs a bankrupt? (Another way to think of it: suppose you have 1 bit of information on both over the market and you earn the same amount. How many trades would it take before your more informed trade ever made a difference? In the first case, you quickly start earning a return and can compound that immediately; in the second case, you might live a hundred lives without ever once seeing a payoff.)
11 comments
Comments sorted by top scores.
comment by kave · 2024-10-23T02:55:32.931Z · LW(p) · GW(p)
I don't understand why you would short the market if your P(Doom) is high. I think most Dooms don't involve shorts paying off?
Replies from: quetzal_rainbow, korin43↑ comment by quetzal_rainbow · 2024-10-23T06:59:39.878Z · LW(p) · GW(p)
Theoretically, if everybody starts to believe in doom, they sell their assets to spend on consumption, so market crashes and shorts pay off.
Replies from: donald-hobson↑ comment by Donald Hobson (donald-hobson) · 2024-10-25T13:47:49.680Z · LW(p) · GW(p)
Problems with that.
Doom doesn't imply that everyone believes in doom before it happens.
Do you think that the evidence for doom will be more obvious than the evidence for atheism, while the world is not yet destroyed?
It's quite possible for doom to happen, and most people to have no clue beyond one article with a picture of red glowing eyed robots.
If everyone does believe in doom, there might be a bit of spending on consumption. But there will also be lots of riots, lots of lynching and burning down data centers and stuff like that.
In this bizarre hypothetical where everyone believes doom is coming soon and starts enjoying their money while they can, then society is starting to fall apart, and the price of luxuries is through the roof. Your opportunities to enjoy the money will be limited.
Replies from: quetzal_rainbow, Jemist↑ comment by quetzal_rainbow · 2024-10-25T14:22:06.248Z · LW(p) · GW(p)
I agree with your point! That's why I started with the word "theoretically".
↑ comment by J Bostock (Jemist) · 2024-10-25T14:03:48.158Z · LW(p) · GW(p)
Rob Miles also makes the point that if you expect people to accurately model the incoming doom, you should have a low p(doom). At the very least, worlds in which humanity is switched-on enough (and the AI takeover is slow enough) for both markets to crash and the world to have enough social order for your bet to come through are much more likely to survive. If enough people are selling assets to buy cocaine for the market to crash, either the AI takeover is remarkably slow indeed (comparable to a normal human-human war) or public opinion is so doomy pre-takeover that there would be enough political will to "assertively" shut down the datacenters.
↑ comment by Brendan Long (korin43) · 2024-10-23T15:09:56.023Z · LW(p) · GW(p)
I think the idea is that short position pays off up-front, and then you don't need to worry about the loan if everyone's dead.
If by paying off you mean this bet actually working I think you're right though. It seems more likely that the stock market would go up in the short term, forcing you to cover at a higher price and losing a bunch of money. And if the market stays flat, you'll still lose money on interest payments unless doom is coming this year.
Replies from: Jemist↑ comment by J Bostock (Jemist) · 2024-10-23T22:11:58.228Z · LW(p) · GW(p)
Also, in this case you want to actually spend the money before the world ends. So actually losing money on interests payments isn't the real problem, the real problem is that if you actually enjoy the money you risk losing everything and being bankrupt/in debtors prison for the last two years before the world ends. There's almost no situation in which you can be so sure of not needing to pay the money back that you can actually spend it risk-free. I think the riskiest short-ish thing that is even remotely reasonable is taking out a 30-year mortgage and paying just the minimum amount each year, such that the balance never decreases. Worst case you just end up with no house after 30 years, but not in crippling debt, and move back into the nearest rat group house.
comment by Michael Roe (michael-roe) · 2024-10-23T09:59:32.833Z · LW(p) · GW(p)
Also, there is counterparty risk if you bet on everyone dying.
(Yeah, yeah, you can bet on something like other peoples belief in the impednding apocalypse going up before it actually happens).
“Rapid takeoff” hypotheses are particularly hard to bet on.
Replies from: korin43↑ comment by Brendan Long (korin43) · 2024-10-23T15:12:21.521Z · LW(p) · GW(p)
This is actually why a short position (a complicated loan) would theoretically work. If we all die, then you, as someone else's counterparty, never need to pay your loan back.
(I think this is a bad idea, but not because of counterparty risk)
comment by Tripp Lyons (tripp-lyons) · 2024-11-21T23:30:42.844Z · LW(p) · GW(p)
Let's say the market prices in a 0.1% chance of extinction per century, and you think there's a 50% chance we're dead in the next 25 years. This is about 12 bits of evidence.
Where did the 12 bits number come from? I'm getting bits.
Edit: I think it comes from the odds ratio: bits.
comment by Michael Roe (michael-roe) · 2024-10-23T10:03:35.221Z · LW(p) · GW(p)
In any case, as a researcher currently working in this area, I am putting a big bet on moderate badness happening (in that I could be working on something else, and my time has value).