Posts
Comments
I am not sure that example fully makes sense. If trade is possible then two people with 11 units of resources can get together and do a cost 20 project. That is why companies have shares, they let people chip in so you can make a Suez Canal even if no single person on Earth is rich enough to afford a Suez Canal.
I suppose in extreme cases where everyone is on or near the breadline some of that "Stag Hunt" vs "Rabbit Hunt" stuff could apply.
I agree with you that, if we need to tax something to pay for our government services, then inheritance tax is arguably not a terrible choice.
But a lot of your arguments seem a bit problematic to me. First, as a point of basic practicality, why 100%? Couldn't most of your aims be achieved with a lesser percentage? That would also smooth out weird edge cases.
There is something fundamentally compelling about the idea that every generation should start fresh, free from the accumulated advantages or disadvantages of their ancestors.
This quote stood out to me as interesting. I know this isn't what you meant, but as a society it would be really weird to collectively decide "don't give the next generation fire, they need to start fresh and rediscover that for themselves. We shouldn't give them the accumulated advantages of their ancestors, send them to the wilderness and let them start fresh!".
I think I am not understanding the question this equation is supposed to be answer, as it seems wrong to me.
I think you are considering the case were we draw arrowheads on the lines? So each line is either an "input" or an "output", and we randomly connect inputs only to outputs, never connecting two inputs together or two outputs? With those assumptions I think the probability of only one loop on a shape with N inputs and N outputs (for a total of 2N "puts") is 1/N.
The equation I had ( (N-2)!! / (N-1)!!) is for N "points", which are not pre-assigned into inputs and outputs.
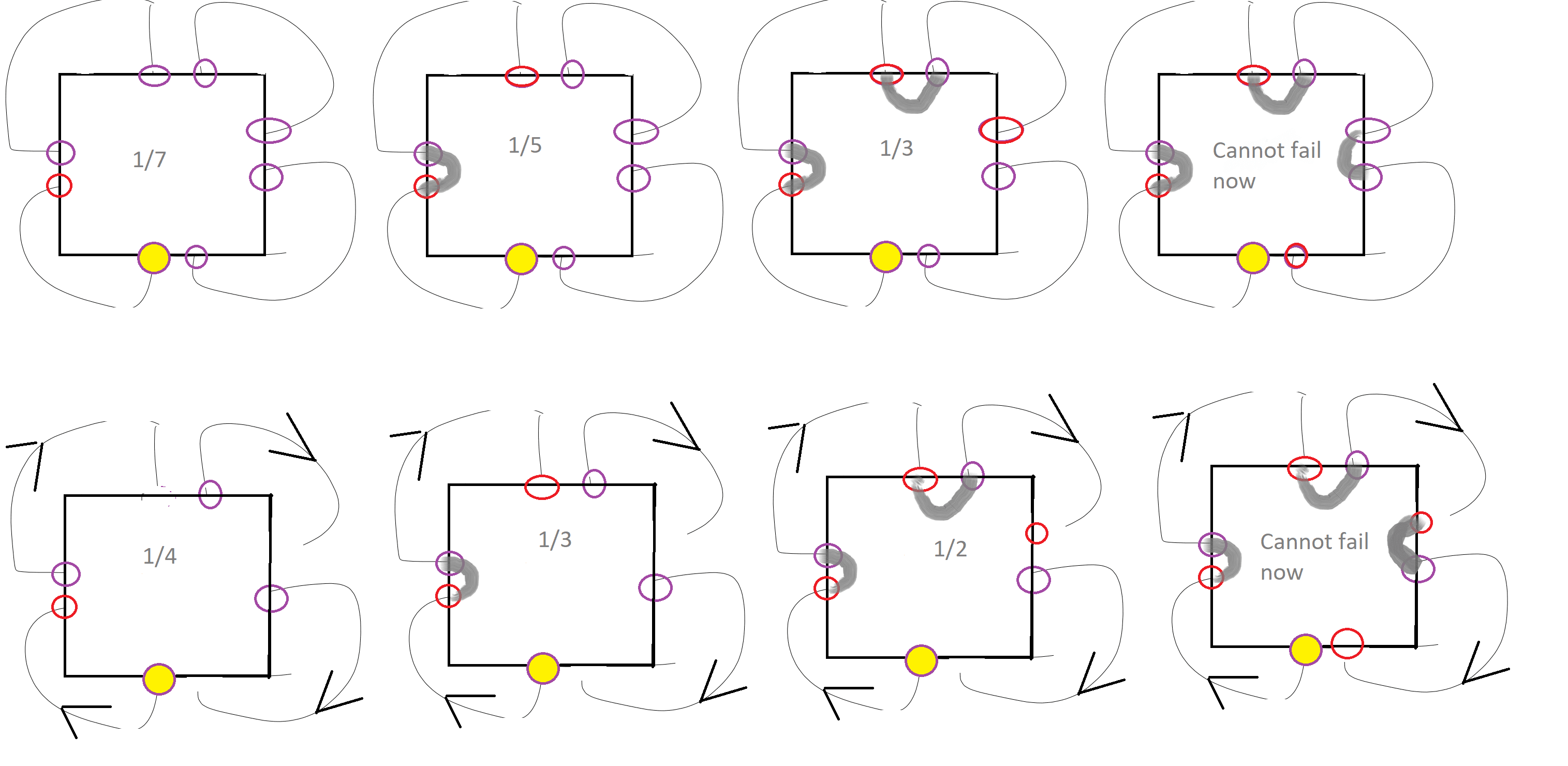
These diagrams explain my logic. On the top row is the "N puts" problem. First panel on the left, we pick a unmatched end (doesn't matter which, by symmetry), the one we picked is the red circle, and we look at the options of what to tie it to, the purple circles. One purple circle is filled with yellow, if we pick that one then we will end up with more than one loop. The probability of picking it randomly is 1/7 (as their are 6 other options). In the next panel we assume we didn't die. By symmetry again it doesn't matter which of the others we connected to, so I just picked the next clockwise. We will follow the loop around. We are now looking to match the newly-red point to another purple. Now their are 5 purples, the yellow is again a "dead end", ensuring more than one loop. We have a 1/5 chance of picking it at random. Continuing like this, we eventually find that the probability of having only one loop is just the probability of not picking badly at any step, (6/7)x(4/5)x(2/3) = (N-2)!! / (N-1)!!.
In the second row I do the same thing for the case where the lines have arrows, instead of 8 ports we have 4 input ports and 4 output ports, and inputs can only be linked to outputs. This changes things, because now each time we make a connection we only reduce the number of options by one at the next step. (Because our new input was never an option as an output). The one-loop chance here comes out as (3/4)x(2/3)x(1/2) = (N-1)! / N! = 1/N. Neither expression seems to match the equations you shared, so either I have gone wrong with my methods or you are answering a different question.
This is really wonderful, thank you so much for sharing. I have been playing with your code.
The probability that their is only one loop is also very interesting. I worked out something, which feels like it is probably already well known, but not to me until now, for the simplest case.
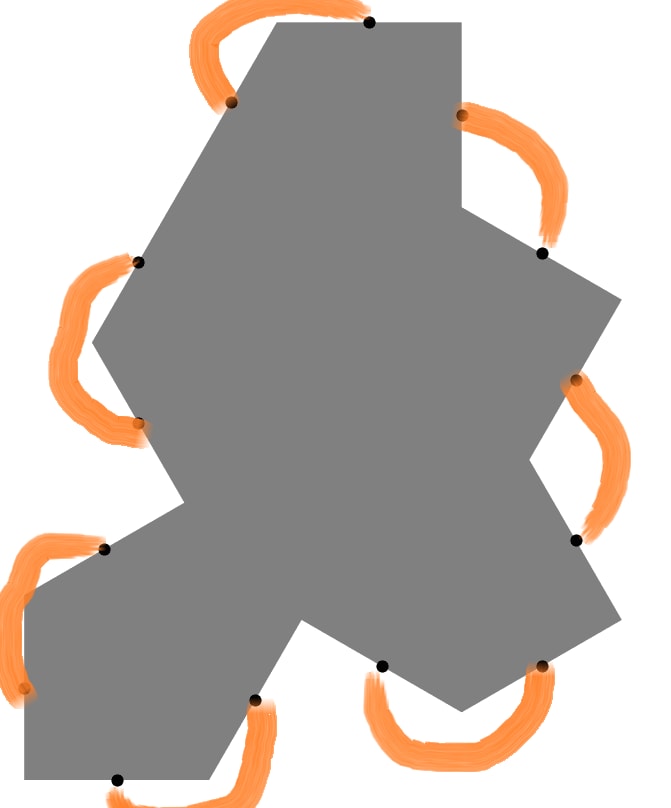
In the simplest case is one tile. The orange lines are the "edging rule". Pick one black point and connect it to another at random. This has a 1/13 chance of immediately creating a closed loop, meaning more than one loop total. Assuming it doesn't do that, the next connection we make has 1/11 chance of failure. The one after 1/9. Etc.
So the total probability of having only one loop is the product: (12/13) (10/11) (8/9) (6/7) (4/5) (2/3), which can be written as 12!! / 13!! (!! double factorial). For a single tile this comes out at 35% ish. (35% chance of only one loop).
If we had a single shape with N sides we would get a probability of (N-2)!! / (N-1)!! .
The probability for a collection of tiles is, as you say, much harder. Each edge point might not uniformly couple to all other edge points because of the multi-stepping in between. Also loops can form that never go to the edge. So the overall probability is most likely less than (N-2)!!/(N-1)!! for N edge dots.
That is a nice idea. The "two sides at 180 degrees" only occurred to me after I had finished. I may look into that one day, but with that many connections is needs to be automated.
In the 6 entries/exits ones above you pick one entry, you have 5 options of where to connect it. Then, you pick the next unused entry clockwise, and have 3 options for where to send it, then you have only one option for how to connect the last two. So its 5x3x1 = 15 different possible tiles.
With 14 entries/exits, its 13x11x9x7x5x3x1 = 135,135 different tiles. (13!!, for !! being double factorial).
You also have (I think) 13+12+11+10+... = 91 different connection pieces.
One day, I may try and write a code to make some of those. I strongly suspect that they won't look nice, but they might be interesting anyway.
I still find the effect weird, but something that I think makes it more clear is this phase space diagram:
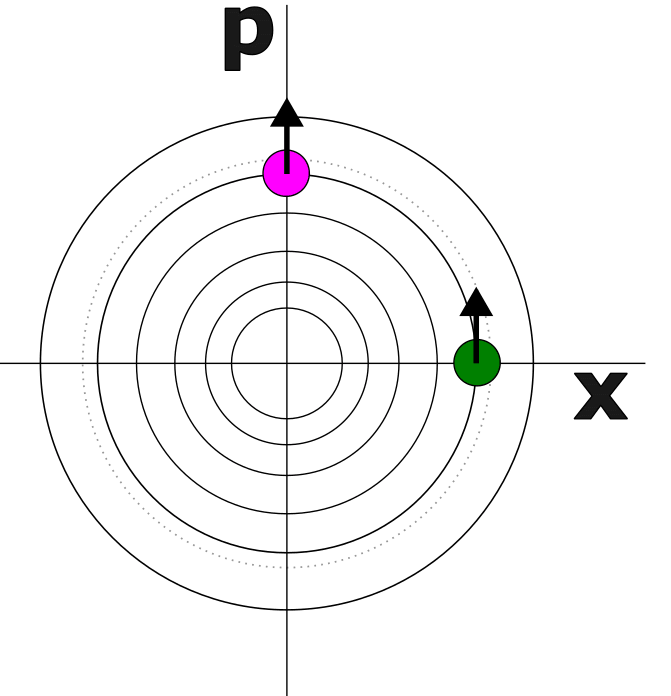
We are treating the situation as 1D, and the circles in the x, p space are energy contours. Total energy is distance from the origin. An object in orbit goes in circles with a fixed distance from the origin. (IE a fixed total energy).
The green and purple points are two points on the same orbit. At purple we have maximum momentum and minimum potential energy. At green its the other way around. The arrows show impulses, if we could suddenly add momentum of a fixed amount by firing the rocket those are the arrows.
Its visually clear that the arrow from the purple point is more efficient. It gets us more than one whole solid-black energy contour higher, in contrast the same length of arrow at the green position only gets us to the dashed orbit, which is lower.
Visually we can see that if we want to get away from the origin of that x, p coordinate system we should shoot when out boost vector aligns with out existing vector.
A weird consequence. Say our spaceship didn't have a rocket, but instead it had a machine that teleported the ship a fixed distance (say 100m). (A fixed change in position, instead of a fixed change in momentum). In this diagram that is just rotating the arrows 90 degrees. This implies the most efficient time to use the teleporting machine is when you are at the maximum distance from the planet (minimum kinetic energy, maximum potential). Mathematically this is because the potential energy has the same quadratic scaling as the kinetic. Visually, its because its where you are adding the new vector to your existing vector most efficiently.
I am not sure that is right. A very large percentage of people really don't think the rolls are independent. Have you ever met anyone who believed in fate, Karma, horoscopes , lucky objects or prayer? They don't think its (fully) random and independent. I think the majority of the human population believe in one or more of those things.
If someone spells a word wrong in a spelling test, then its possible they mistyped, but if its a word most people can't spell correctly then the hypothesis "they don't know the spelling' should dominate. Similarly, I think it is fair to say that a very large fraction of humans (over 50%?) don't actually think dice rolls or coin tosses are independent and random.
That is a cool idea! I started writing a reply, but it got a bit long so I decided to make it its own post in the end. ( https://www.lesswrong.com/posts/AhmZBCKXAeAitqAYz/celtic-knots-on-einstein-lattice )
I stuck to maximal density for two reaosns, (1) to continue the Celtic knot analogy (2) because it means all tiles are always compatible (you can fit two side by side at any orientation without loosing continuity). With tiles that dont use every facet this becomes an issue.
Thinking about it now, and without having checked carefully, I think this compatibilty does something topological and forces odd macrostructure. For example, if we have a line of 4-tiles in a sea of 6-tiles (4 tiles use four facets), then we cant end the line of 4 tiles without breaking continuity. So the wall has to loop, or go off the end. The 'missing lines' the 4 tiles lacked (that would have made them 6's) would have been looped through the 4-wall. So having those tiles available is kind of like being able to delete a few closed loops from a 6s structure.
I might try messing with 4s to see if you are right that they will be asthetically useful.
That's a very interesting idea. I tried going through the blue one at the end.
Its not possible in that case for each string to strictly alternate between going over and under, by any of the rules I have tried. In some cases two strings pass over/under one another, then those same two strings meet again when one has travelled two tiles and the other three. So they are de-synced. They both think its their turn to go over (or under).
The rules I tried to apply were (all of which I believe don't work):
- Over for one tile, under for the next (along each string)
- Over for one collision, under for the next (0, 1 or 2 collisions, are possible in a tile)
- Each string follows the sequence 'highest, middle, lowest, highest, middle lowest...' for each tile it enters.
My feeling having played with it for about 30-45 mins is that there probably is a rule nearby to those above that makes things nice, but I haven't yet found it.
I wasn't aware of that game. Yes it is identical in terms of the tile designs. Thank you for sharing that, it was very interesting and that Tantrix wiki page lead me to this one, https://en.wikipedia.org/wiki/Serpentiles , which goes into some interesting related stuff with two strings per side or differently shaped tiles.
Something related that I find interesting, for people inside a company, the real rival isn't another company doing the same thing, but people in your own company doing a different thing.
Imagine you work at Microsoft in the AI research team in 2021. Management want to cut R&D spending, so either your lot or the team doing quantum computer research are going to be redundant soon. Then, the timeline splits. In one universe, Open AI release Chat GPT, in the other PsiQuantum do something super impressive with quantum stuff. In which of those universes do the Microsoft AI team do well? In one, promotions and raises, in the other, redundancy.
People recognise this instinctively. Changing companies is much faster and easier than changing specialities. So people care primarily about their speciality doing well, their own specific company is a secondary concern.
A fusion expert can expert at a different fusion company way faster and more easily than they can become an expert in wind turbines. Therefore, to the fusion expert all fusion companies are on the same side against the abominable wind farmers. I suspect this is also true of most people in AI, although maybe when talking to the press they will be honour bound to claim otherwise.
I wonder if any of the perceived difference between fusion and AI might be which information sources are available to you. It sounds like you have met the fusion people, and read their trade magazines, and are comparing that to what mainstream news says about AI companies (which won't necessarily reflect the opinions of a median AI researcher.).
I think economics should be taught to children, not for the reasons you express, but because it seems perverse that I spent time at school learning about Vikings, Oxbow lakes, volcanoes, Shakespeare and Castles, but not about the economic system of resource distribution that surrounds me for the rest of my life. When I was about 9 I remember asking why 'they' didn't just print more money until everyone had enough. I was fortunate to have parents who could give a good answer, not everyone will be.
Stock buybacks! Thank you. That is definitely going to be a big part f the "I am missing something here" I was expressing above.
I freely admit to not really understanding how shares are priced. To me it seems like the value of a share should be related to the expected dividend pay-out of that share over the remaining lifetime of the company, with a discount rate applied on pay-outs that are expected to happen further in the future (IE dividend yields 100 years from now are valued much less than equivalent payments this year). By this measure, justifying the current price sounds hard.
Google says that the annual dividend on Nvidia shares is 0.032%. (Yes, the leading digits are 0.0). So, right now, you get a much better rate of return just leaving your money in your bank's current account. So, at least by this measure, Nvidia shares are ludicrously over-priced. You could argue that future Nvidia pay outs might be much larger than the historical ones due to some big AI related profits. But, I don't find this argument convincing. Are future pay outs going to be 100x bigger? It would require a 100-fold yield increase for it to just be competitive with a savings account. If you time discount a little (say those 100-fold increases don't materialise for 3 years) then it looks even worse.
Now, clearly the world doesn't value shares according to the same heuristics that make sense to a non-expert like me. For example, the method "time integrate future expected dividend pay outs with some kind of time discounting" tells us that cryptocurrencies are worthless, because they are like shares with zero dividends. But, people clearly do put a nonzero value on bitcoin - and there is no plausible way that many people are that wrong. So they are grasping something that I am missing, and that same thing is probably what allows company shares to be prices so high relative to the dividends.
That is very interesting! That does sound weird.
In some papers people write density operators using an enhanced "double ket" Dirac notation, where eg. density operators are written to look like |x>>, with two ">"'s. They do this exactly because the differential equations look more elegant.
I think in this notation measurements look like <<m|, but am not sure about that. The QuTiP software (which is very common in quantum modelling) uses something like this under-the-hood, where operators (eg density operators) are stored internally using 1d vectors, and the super-operators (maps from operators to operators) are stored as matrices.
So structuring the notation in other ways does happen, in ways that look quite reminiscent of your tensors (maybe the same).
Yes, in your example a recipient who doesn't know the seed models the light as unpolarised, and one who does as say, H-polarised in a given run. But for everyone who doesn't see the random seed its the same density matrix.
Lets replace that first machine with a similar one that produces a polarisation entangled photon pair, |HH> + |VV> (ignoring normalisation). If you have one of those photons it looks unpolarised (essentially your "ignorance of the random seed" can be thought of as your ignorance of the polarisation of the other photon).
If someone else (possibly outside your light cone) measures the other photon in the HV basis then half the time they will project your photon into |H> and half the time into |V>, each with 50% probability. This 50/50 appears in the density matrix, not the wavefunction, so is "ignorance probability".
In this case, by what I understand to be your position, the fact of the matter is either (1) that the photon is still entangled with a distant photon, or (2) that it has been projected into a specific polarisation by a measurement on that distant photon. Its not clear when the transformation from (1) to (2) takes place (if its instant, then in which reference frame?).
So, in the bigger context of this conversation,
OP: "You live in the density matrices (Neo)"
Charlie :"No, a density matrix incorporates my own ignorance so is not a sensible picture of the fundamental reality. I can use them mathematically, but the underlying reality is built of quantum states, and that randomness when I subject them to measurements is fundamentally part of the territory, not the map. Lets not mix the two things up."
Me: "Whether a given unit of randomness is in the map (IE ignorance), or the territory is subtle. Things that randomly combine quantum states (my first machine) have a symmetry over which underlying quantum states are being mixed that looks meaningful. Plus (this post), the randomness can move abruptly from the territory to the map due to events outside your own light cone (although the amount of randomness is conserved), so maybe worrying too much about the distinction isn't that helpful.
What is the Bayesian argument, if one exists, for why quantum dynamics breaks the “probability is in the mind” philosophy?
In my world-view the argument is based on Bell inequalities. Other answers mention them, I will try and give more of an introduction.
First, context. We can reason inside a theory, and we can reason about a theory. The two are completely different and give different intuitions. Anyone talking about "but the complex amplitudes exist" or "we are in one Everett branch" is reasoning inside the theory. The theory, as given in the textbooks, is accepted as true and interpretations built on.
However, both historically and (I think) more generally, we should also reason about theories. This means we need to look at experimental observations, and ask questions like "what is the most reasonable model?".
Many quantum experiments give random-looking results. As you point out, randomness is usually just "in the mind". Reality was deterministic, but we couldn't see everything. The terminology is "local hidden variable". For an experiment where you draw a card from a deck the "local hidden variable" was which card was on top. In a lottery with (assumedly deterministic) pinballs the local hidden variable is some very specific details of the initial momentums and positions of the balls. In other words the local hidden variable is the thing that you don't know, so to you it looks random. Its the seed of your pseudorandom number generator.
Entanglement - It is possible to prepare two (or more) particles in a state, such that measurements of those two particles gives very weird results. What do I mean by "very weird". Well, in a classical setting if Alice and Bob are measuring two separate objects then there are three possible (extremal) situations (1): Their results are completely uncorrelated, for example Alice is rolling a dice in Texas and Bob is rolling a different dice in London. (2) Correlated, for example, Alice is reading an email telling her she got a job she applied for, and Bob is reading an email telling him he failed to get the same job. (4) Signalling (we skipped 3 on purpose, we will get to that). Alice and Bob have phones, and so the data they receive is related to what the other of them is doing. Linear combinations of the above (eg noisy radio messages, correlation that is nor perfect etc) are also possible.
By very weird, I mean that quantum experiments give rise (in the raw experimental data, before any theory is glued on) to a fourth type of relation; (3): Non-locality. Alice and Bob's measurement outcomes (observations) are random, but the correlation between their observation's changes depending on the measurements they both chose to make (inputs). Mathematically its no more complex than the others, but its fiddly to get your head around because its not something seen in everyday life.
An important feature of (3) is that it cannot be used to create signalling (4). However, (3) cannot be created out of any mixture of (1) and (2). (Just like (4) cannot be created by mixing (1) and (2)). In short, if you have any one of these 4 things, you can use local actions to go "down hill" to lower numbers but you can't go up.
Anyway, "hidden variables" are shorthand for "(1) and (2)" (randomness and correlation). The "local" means "no signalling" (IE no (3), no radios). The reason we insist on no signalling is because the measurements Alice and Bob do on their particles could be outside one another's light cones (so even a lightspeed signal would not be fast enough to explain the statistics). The "no signalling" condition might sound artificial, but if you allow faster than light signalling then you are (by the standards of relativity) also allowing time travel.
Bell inequality experiments have been done. They measure result (3). (3) cannot be made out of ordinary "ignorance" probabilities (cannot be made from (2)). (3) could be made out of (4) (faster than light signalling), but we don't see the signalling itself, and assuming it exists entails time travel.
So, if we reject signalling, we know that whatever it is that is happening in a Bell inequality experiment it can't be merely apparent randomness due to our ignorance. We also know the individual results collected by Alice and Bob look random (but not the correlations between the results), this backs us into the corner of accepting that the randomness is somehow an intrinsic feature of the world, even the photon didn't "know" if it would go through the polariser until you tried it.
The wiki article on Bell inequalities isn't very good unfortunately.
Just the greentext. Yes, I totally agree that the study probably never happened. I just engaged with the actualy underling hypothesis, and to do so felt like some summary of the study helped. But I phrased it badly and it seems like I am claiming the study actually happened. I will edit.
I thought they were typically wavefunction to wavefunction maps, and they need some sort of sandwiching to apply to density matrices?
Yes, this is correct. My mistake, it does indeed need the sandwiching like this .
From your talk on tensors, I am sure it will not surprise you at all to know that the sandwhich thing itself (mapping from operators to operators) is often called a superoperator.
I think the reason it is as it is is their isn't a clear line between operators that modify the state and those that represent measurements. For example, the Hamiltonian operator evolves the state with time. But, taking the trace of the Hamiltonian operator applied to the state gives the expectation value of the energy.
The way it works normally is that you have a state , and its acted on by some operator, , which you can write as . But this doesn't give a number, it gives a new state like the old but different. (For example if a was the anhilation operator the new state is like the old state but with one fewer photons). This is how (for example) an operator acts on the state of the system to change that state. (Its a density matrix to density matrix map).
In dimensions terms this is: (1,1) = (1, 1) * (1,1)
(Two square matrices of size N multiply to give another square matrix of size N).
However, to get the expected outcome of a measurement on a particular state you take : where Tr is the trace. The trace basically gets the "plug" at the left hand side of a matrix and twists it around to plug it into the right hand side. So overall what is happening is that the operators and , each have shapes (1,1) and what we do is:
Tr( (1,1) * (1,1)) = Tr( (1, 1) ) = number.
The "inward facing" dimensions of each matrix get plugged into one another because the matrices multiply, and the outward facing dimensions get redirected by the trace operation to also plug into one another. (The Trace is like matrix multiplication but on paper that has been rolled up into a cylinder, so each of the two matrices inside sees the other on both sides). The net effect is exactly the same as if they had originally been organized into the shapes you suggest of (2,0) and (0,2) respectively.
So if the two "ports" are called A and B your way of doing it gives:
(AB, 0) * (0, AB) = (0, 0) IE number
The traditional way:
Tr( (A, B) * (B, A) ) = Tr( (A, A) ) = (0, 0) , IE number.
I haven't looked at tensors much but I think that in tensor-land this Trace operation takes the role of a really boring metric tensor that is just (1,1,1,1...) down the diagonal.
So (assuming I understand right) your way of doing it is cleaner and more elegant for getting the expectation value of a measurement. But the traditional system works more elegantly for applying an operator too a state to evolve it into another state.
You are completely correct in the "how does the machine work inside?" question. As you point out that density matrix has the exact form of something that is entangled with something else.
I think its very important to be discussing what is real, although as we always have a nonzero inferential distance between ourselves and the real the discussion has to be a little bit caveated and pragmatic.
I think the reason is that in quantum physics we also have operators representing processes (like the Hamiltonian operator making the system evolve with time, or the position operator that "measures" position, or the creation operator that adds a photon), and the density matrix has exactly the same mathematical form as these other operators (apart from the fact the density matrix needs to be normalized).
But that doesn't really solve the mystery fully, because they could all just be called "matrices" or "tensors" instead of "operators". (Maybe it gets us halfway to an explanation, because all of the ones other than the density operator look like they "operate" on the system to make it change its state.)
Speculatively, it might be to do with the fact that some of these operators are applied on continuous variables (like position), where the matrix representation has infinite rows and infinite columns - maybe their is some technicality where if you have an object like that you have to stop using the word "matrix" or the maths police lock you up.
There are some non-obvious issues with saying "the wavefunction really exists, but the density matrix is only a representation of our own ignorance". Its a perfectly defensible viewpoint, but I think it is interesting to look at some of its potential problems:
- A process or machine prepares either |0> or |1> at random, each with 50% probability. Another machine prepares either |+> or |-> based on a coin flick, where |+> = (|0> + |1>)/root2, and |+> = (|0> - |1>)/root2. In your ontology these are actually different machines that produce different states. In contrast, in the density matrix formulation these are alternative descriptions of the same machine. In any possible experiment, the two machines are identical. Exactly how much of a problem this is for believing in wavefuntions but not density matrices is debatable - "two things can look the same, big deal" vs "but, experiments are the ultimate arbiters of truth, if experiemnt says they are the same thing then they must be and the theory needs fixing."
- There are many different mathematical representations of quantum theory. For example, instead of states in Hilbert space we can use quasi-probability distributions in phase space, or path integrals. The relevance to this discussion is that the quasi-probability distributions in phase space are equivalent to density matrices, not wavefunctions. To exaggerate the case, imagine that we have a large number of different ways of putting quantum physics into a mathematical language, [A, B, C, D....] and so on. All of them are physically the same theory, just couched in different mathematics language, a bit like say, ["Hello", "Hola", "Bonjour", "Ciao"...] all mean the same thing in different languages. But, wavefunctions only exist as an entity separable from density matrices in some of those descriptions. If you had never seen another language maybe the fact that the word "Hello" contains the word "Hell" as a substring might seem to possibly correspond to something fundamental about what a greeting is (after all, "Hell is other people"). But its just a feature of English, and languages with an equal ability to greet don't have it. Within the Hilbert space language it looks like wavefunctions might have a level of existence that is higher than that of density matrices, but why are you privileging that specific language over others?
- In a wavefunction-only ontology we have two types of randomness, that is normal ignorance and the weird fundamental quantum uncertainty. In the density matrix ontology we have the total probability, plus some weird quantum thing called "coherence" that means some portion of that probability can cancel out when we might otherwise expect it to add together. Taking another analogy (I love those), the split you like is [100ml water + 100ml oil], (but water is just my ignorance and doesn't really exist), and you don't like the density matrix representation of [200ml fluid total, oil content 50%]. Their is no "problem" here per se but I think it helps underline how the two descriptions seem equally valid. When someone else measures your state they either kill its coherence (drop oil % to zero), or they transform its oil into water. Equivalent descriptions.
All of that said, your position is fully reasonable, I am just trying to point out that the way density matrices are usually introduced in teaching or textbooks does make the issue seem a lot more clear cut than I think it really is.
I just looked up the breakfast hypothetical. Its interesting, thanks for sharing it.
So, my understanding is (supposedly) someone asked a lot of prisoners "How would you feel if you hadn't had breakfast this morning?", did IQ tests on the same prisoners and found that the ones who answered "I did have breakfast this morning." or equivalent were on average very low in IQ. (Lets just assume for the purposes of discussion that this did happen as advertised.)
It is interesting. I think in conversation people very often hear the question they were expecting, and if its unexpected enough they hear the words rearranged to make it more expected. There are conversations where the question could fit smoothly, but in most contexts its a weird question that would mostly be measuring "are people hearing what they expect, or what is being actually said". This may also correlate strongly with having English as a second language.
I find the idea "dumb people just can't understand a counterfactual" completely implausible. Without a counterfactual you can't establish causality. Without causality their is no way of connecting action to outcome. How could such a person even learn to use a TV remote? Given that these people (I assume) can operate TV remotes they must in fact understand counterfactuals internally, although its possible they lack the language skills to clearly communicate about them.
The question of "why should the observed frequencies of events be proportional to the square amplitudes" is actually one of the places where many people perceive something fishy or weird with many worlds. [https://www.sciencedirect.com/science/article/pii/S1355219809000306 ]
To clarify, its not a question of possibly rejecting the square-amplitude Born Rule while keeping many worlds. Its a question of whether the square-amplitude Born Rule makes sense within the many worlds perspective, and it if doesn't what should be modified about the many worlds perspective to make it make sense.
I agree with this. Its something about the guilt that makes this work. Also the sense that you went into it yourself somehow reshapes the perception.
I think the loan shark business model maybe follows the same logic. [If you are going to eventually get into a situation where the victim pays or else suffers violence, then why doesn't the perpetrator just skip the costly loan step at the beginning and go in threat first? I assume that the existence of loan sharks (rather than just blackmailers) proves something about how if people feel like they made a bad choice or engaged willingly at some point they are more susceptible. Or maybe its frog boiling.]
On the "what did we start getting right in the 1980's for reducing global poverty" I think most of the answer was a change in direction of China. In the late 70's they started reforming their economy (added more capitalism, less command economy): https://en.wikipedia.org/wiki/Chinese_economic_reform.
Comparing this graph on wiki https://en.wikipedia.org/wiki/Poverty_in_China#/media/File:Poverty_in_China.svg , to yours, it looks like China accounts for practically all of the drop in poverty since the 1980s.
Arguably this is a good example for your other points. More willing participation, less central command.
I don't think the framing "Is behaviour X exploitation?" is the right framing. It takes what (should be) an argument about morality and instead turns it into an argument about the definition of the word "exploitation" (where we take it as given that, whatever the hell we decide exploitation "actually means" it is a bad thing). For example see this post: https://www.lesswrong.com/posts/yCWPkLi8wJvewPbEp/the-noncentral-fallacy-the-worst-argument-in-the-world. Once we have a definition of "exploitation" their might be some weird edge cases that are technically exploitation but are obviously fine.
The substantial argument (I think) is that when two parties have unequal bargaining positions, is it OK for the stronger party to get the best deal it can? A full-widget is worth a million dollars. I possess the only left half of a widget in the world. Ten million people each possess a right half that could doc with my left half. Those not used to make widgets are worthless. What is the ethical split for me to offer for a right half in this case?
[This is maybe kind of equivalent to the dating example you give. At least in my view the "bad thing" in the dating example is the phrase "She begins using this position to change the relationship". The word "change" is the one that sets the alarms for me. If they both went in knowing what was going on then, to me, that's Ok. Its the "trap" that is not. I think most of the things we would object to are like this, those Monday meetings and that expensive suit are implied to be surprises jumped onto poor Bob.]
The teapot comparison (to me) seems to be a bad. I got carried away and wrote a wall of text. Feel free to ignore it!
First, lets think about normal probabilities in everyday life. Sometimes there are more ways for one state to come about that another state, for example if I shuffle a deck of cards the number of orderings that look random is much larger than the number of ways (1) of the cards being exactly in order.
However, this manner of thinking only applies to certain kinds of thing - those that are in-principle distinguishable. If you have a deck of blank cards, there is only one possible order, BBBBBB.... To take another example, an electronic bank account might display a total balance of $100. How many different ways are their for that $100 to be "arranged" in that bank account? The same number as 100 coins labelled "1" through "100"? No, of course not. Its just an integer stored on a computer, and their is only one way of picking out the integer 100. The surprising examples of this come from quantum physics, where photons act more like the bank account, where their is only 1 way of a particular mode to contain 100 indistinguishable photons. We don't need to understand the standard model for this, even if we didn't have any quantum theory at all we could still observe these Boson statistics in experiments.
So now, we encounter anthropic arguments like Doomsday. These arguments are essentially positing a distribution, where we take the exact same physical universe and its entire physical history from beginning to end, (which includes every atom, every synapse firing and so on). We then look at all of the "counting minds" in that universe (people count, ants probably don't, aliens, who knows), and we create a whole slew of "subjective universes", , , , , etc, where each of of them is atomically identical to the original but "I" am born as a different one of those minds (I think these are sometimes called "centred worlds"). We assume that all of these subjective universes were, in the first place, equally likely, and we start finding it a really weird coincidence that in the one we find ourselves in we are a human (instead of an Ant), or that we are early in history. This is, as I understand it, The Argument. You can phrase it without explicitly mentioning the different s, by saying "if there are trillions of people in the future, the chances of me being born in the present are very low. So, the fact I was born now should update me away from believing there will be trillions of people in the future". - but the s are still doing all the work in the background.
The conclusion depends on treating all those different subscripted s as distinguishable, like we would for cards that had symbols printed on them. But, if all the cards in the deck are identical there is only one sequence possible. I believe that all of the , , , 's etc are identical in this manner. By assumption they are atomically identical at all times in history, they differ only by which one of the thinking apes gets assigned the arbitrary label "me" - which isn't physically represented in any particle. You think they look different, and if we accept that we can indeed make these arguments, but if you think they are merely different descriptions of the same exact thing then the Doomsday argument no longer makes sense, and possibly some other anthropic arguments also fall apart. I don't think they do look different, if every "I" in the universe suddenly swapped places - but leaving all memories and personality behind in the physical synapses etc, then, how would I even know it? I would be a cyborg fighting in WWXIV and would have no memories of ever being some puny human typing on a web forum in the 21s Cent. Instead of imaging that I was born as someone else I could imagine that I could wake up as someone else, and in any case I wouldn't know any different.
So, at least to me, it looks like the anthropic arguments are advancing the idea of this orbital teapot (the different scripted s, although it is, in fairness, a very conceptually plausible teapot). There are, to me, three possible responses:
1 - This set of different worlds doesn't logically exist. You could push this for this response by arguing "I couldn't have been anyone but me, by definition." [Reject the premise entirely - there is no teapot]
2 - This set of different worlds does logically make sense, and after accepting it I see that it is a suspicious coincidence I am so early in history and I should worry about that. [accept the argument - there is a ceramic teapot orbiting Mars]
3 - This set of different worlds does logically make sense, but they should be treated like indistinguishable particles, blank playing cards or bank balances. [accept the core premise, but question its details in a way that rejects the conclusion - there is a teapot, but its chocolate, not ceramic.].
So, my point (after all that, Sorry!) is that I don't see any reason why (2) is more convincing that (3).
[For me personally, I don't like (1) because I think it does badly in cases where I get replicated in the future (eg sleeping beauty problems, or mind uploads or whatever). I reject (2) because the end result of accepting it is that I can infer information through evidence that is not causally linked to the information I gain (eg. I discover that the historical human population was much bigger than previously reported, and as a result I conclude the apocalypse is further in the future than I previously supposed). This leads me to thinking (3) seems right-ish, although I readily admit to being unsure about all this.].
I found this post to be a really interesting discussion of why organisms that sexually reproduce have been successful and how the whole thing emerges. I found the writing style, where it switched rapidly between relatively serious biology and silly jokes very engaging.
Many of the sub claims seem to be well referenced (I particularly liked the swordless ancestor to the swordfish liking mates who had had artificial swords attached).
"Stock prices represent the market's best guess at a stock's future price."
But they are not the same as the market's best guess at its future price. If you have a raffle ticket that will, 100% for definite, win $100 when the raffle happens in 10 years time, the the market's best guess of its future price is $100, but nobody is going to buy it for $100, because $100 now is better than $100 in 10 years.
Whatever it is that people think the stock will be worth in the future, they will pay less than that for it now. (Because $100 in the future isn't as good as just having the money now). So even if it was a cosmic law of the universe that all companies become more productive over time, and everyone knew this to be true, the stocks in those companies would still go up over time, like the raffle ticket approaching the pay day.
Toy example:
1990 - Stocks in C cost $10. Everyone thinks they will be worth $20 by the year 2000, but 10 years is a reasonably long time to wait to double your money so these two things (the expectation of 20 in the future, and the reality of 10 now) coexist without contradiction.
2000 - Stocks in C now cost $20, as expected. People now think that by 2010 they will be worth $40.
Other Ant-worriers are out there!
""it turned out this way, so I guess it had to be this way" doesn't resolve my confusion"
Sorry, I mixed the position I hold (that they maybe work like bosons) and the position I was trying to argue for, which was an argument in favor of confusion.
I can't prove (or even strongly motivate) my "the imaginary mind-swap procedure works like a swap of indistinguishable bosons" assumption, but, as far as I know no one arguing for Anthropic arguments can prove (or strongly motivate) the inverse position - which is essential for many of these arguments to work. I agree with you that we don't have a standard model of minds, and without such a model the Doomsday Argument, and the related problem of being cosmically early might not be problems at all.
Interestingly, I don't think the weird boson argument actually does anything for worries about whether we are simulations, or Boltzmann brains - those fears (I think) survive intact.
I suspect there is a large variation between countries in how safely taxi drivers drive relative to others.
In London my impression is that the taxis are driven more safely than non-taxis. In Singapore it appears obvious to casual observation that taxis are much less safely driven than most of the cars.
At least in my view, all the questions like the "Doomsday argument" and "why am I early in cosmological" history are putting far, far too much weight on the anthropic component.
If I don't know how many X's their are, and I learn that one of them is numbered 20 billion then sure, my best guess is that there are 40 billion total. But its a very hazy guess.
If I don't know how many X's will be produced next year, but I know 150 million were produced this year, my best guess is 150 million next year. But is a very hazy guess.
If I know that the population of X's has been exponentially growing with some coefficient then my best guess for the future is to infer that out to future times.
If I think I know a bunch of stuff about the amount of food the Earth can produce, the chances of asteroid impacts, nuclear wars, dangerous AIs or the end of the Mayan calendar then I can presumably update on those to make better predictions of the number of people in the future.
My take is that the Doomsday argument would be the best guess you could make if you knew literally nothing else about human beings apart from the number that came before you. If you happen to know anything else at all about the world (eg. that humans reproduce, or that the population is growing) then you are perfectly at liberty to make use of that richer information and put forward a better guess. Someone who traces out the exponential of human population growth out to the heat death of the universe is being a bit silly (lets call this the Exponentiator Argument), but on pure reasoning grounds they are miles ahead of the Doomsday argument, because both of them applied a natural, but naïve, interpolation to a dataset, but the exponentiator interpolated from a much richer and more detailed dataset.
Similarly to answer "why are you early" you should use all the data at your disposal. Given who your parents are, what your job is, your lack of cybernetic or genetic enhancements, how could you not be early? Sure, you might be a simulation of someone who only thinks they are in the 21st centaury, but you already know from what you can see and remember that you aren't a cyborg in the year 10,000, so you can't include that possibility in your imaginary dataset that you are using to reason about how early you are.
As a child, I used to worry a lot about what a weird coincidence it was that I was born a human being, and not an ant, given that ants are so much more numerous. But now, when I try and imagine a world where "I" was instead born as the ant, and the ant born as me, I can't point to in what physical sense that world is different from our own. I can't even coherently point to in what metaphysical sense it is different. Before we can talk about probabilities as an average over possibilities we need to know if the different possibilities are even different, or just different labelling on the same outcome. To me, there is a pleasing comparison to be made with how bosons work. If you think about a situation where two identical bosons have their positions swapped, it "counts as" the same situation as before the swap, and you DON'T count it again when doing statistics. Similarly, I think if two identical minds are swapped you shouldn't treat it as a new situation to average over, its indistinguishable. This is why the cyborgs are irrelevant, you don't have an identical set of memories.
I remember reading something about the Great Leap Forward in China (it may have been the Cultural Revolution, but I think it was the Great Leap Forward) where some communist party official recognised that the policy had killed a lot of people and ruined the lives of nearly an entire generation, but they argued it was still a net good because it would enrich future generations of people in China.
For individuals you weigh up the risk/rewards of differing your resource for the future. But, as a society asking individuals to give up a lot of potential utility for unborn future generations is a harder sell. It requires coercion.
I think we might be talking past each other. I will try and clarify what I meant.
Firstly, I fully agree with you that standard game theory should give you access to randomization mechanisms. I was just saying that I think that hypotheticals where you are judged on the process you use to decide, and not on your final decision are a bad way of working out which processes are good, because the hypothetical can just declare any process to be the one it rewards by fiat.
Related to the randomization mechanisms, in the kinds of problems people worry about with predictors guessing your actions in advance its very important to distinguish between [1] (pseudo-)randomization processes that the predictor can predict, and [2] ones that it cannot.
[1] Randomisation that can be predicted by the predictor is (I think) a completely uncontroversial resource to give agents in these problems. In this case we don't need to make predictions like "the agent will randomise", because we can instead make the stronger prediction "the agent will randomize, and the seed of their RNG is this, so they will take one box" which is just a longer way of saying "they will one box". We don't need the predictor to show its working by mentioning the RNG intermediate step.
[2] Randomisation that is beyond the predictor's power is (I think) not the kind of thing that can sensibly be included in these thought experiments. We cannot simultaneously assume that the predictor is pretty good at predicting our actions and useless at predicting a random number generator we might use to choose our actions. The premises: "Alice has a perfect quantum random number generator that is completely beyond the power of Omega to predict. Alice uses this machine to make decisions. Omega can predict Alice's decisions with 99% accuracy" are incoherent.
So I don't see how randomization helps. The first kind, [1] doesn't change anything, and the second kind [2], seems like it cannot be consistently combined with the premise of the question. Perfect predictors and perfect random number generators cannot exist in the same universe.
Their might be interesting nearby problems where you imagine the predictor is 100% effective at determining the agents algorithm, but because the agent has access to a perfect random number generator that it cannot predict their actions. Maybe this is what you meant? In this kind of situation I am still much happier with rules like "It will fill the box with gold if it knows their is a <50% chance of you picking it", [the closest we can get to "outcomes not processes" in probabilistic land], (or perhaps the alternative "the probability that it fills the box with gold is one-minus the probability with which it predicts the agent will pick the box".). But rules like "It will fill the box with gold if the agents process uses either randomisation or causal decision theory" seem unhelpful to me.
I see where you are coming from. But, I think the reason we are interested in CDT (for any DT) in the first place is because we want to know which one works best. However, if we allow the outcomes to be judged not just on the decision we make, but also on the process used to reach that decision then I don't think we can learn anything useful.
Or, to put it from a different angle, IF the process P is used to reach decision X, but my "score" depends not just on X but also P then that can be mapped to a different problem where my decision is "P and X", and I use some other process (P') to decide which P to use.
For example, if a student on a maths paper is told they will be marked not just on the answer they give, but the working out they write on the paper - with points deducted for crossings outs or mistakes - we could easily imagine the student using other sheets of paper (or the inside of their head) to first work out the working they are going to show and the answer that goes with it. Here the decision problem "output" is the entire exame paper, not just the answer.
I like this framing.
An alternative framing, which I think is also part of the answer is that some art is supposed to hit a very large audience and give each a small amount of utility, and other art is supposed to hit a smaller, more specialized, audience very hard. This framing explains things like traditional daytime TV, stuff that no one really loves but a large number of bored people find kind of unobjectionable. And how that is different from the more specialist TV you might actually look forward to an episode off but might hit a smaller audience.
(Obviously some things can hit a big audience and be good, and others can be bad on both counts. But the interesting quadrants two compare are the other two).
Random thoughts. You can relatively simply get a global phase factor at each timestep if you want. I don;t think a global phase factor at each step really counts as meaningfully different though. Anyway, as an example of this:
So that, at each (classical) timestep every single element of the CA tape just moves one step to the right. (So any patterns of 1's and 0's just orbit the tape in circles forever, unchanging.). Its quite a boring CA, but a simple example.
We can take the quantum CA that is exactly the same, but with some complex phase factor:
Where the delta function is saying "1 iff , else 0."
This is exactly the same as the old classical one (everything moves on step to the right), but this time we also have a global phase factor applied to the total system. The total phase factor is , where N is the total number of cells on the tape.
Tiny bit more interesting:
Now we only gain phase factors on values of 1, so the global phase depends on the total number of 1's on the tape, rather than its length.
To get proper quantum stuff we need phase factors that are not global. (IE some relative phases). I feel like this equation below is a reasonable kind of place to start, but I have run out of time for now so might return to this later.
After finding a Unitary that comes from one of your classical Cellular Automata then any power of that unitary will also be a valid unitary. So for example in classical logic their is a the "swap" gate for binary inputs, but in quantum computing the "square-root swap" gate also exists.
So you can get one of your existing unitary matrices, and (for example) take its square root. That would kind of be like a quantum system doing the classical Cellular Automata, that is interrupted halfway through the first step. (Because applying the root matrix twice is the same as applying the matrix). Similarly you can look at the 1/3rd step by applying the cube root of the matrix.
So would you consider the square root matrix a quantum elementary CA? Its not exactly equivalent to anything classical, because classically you can't look "between the steps".
[This is a long winded way of me saying that I don't "get" the question. You want a unitary, U, of the form given in that equation for <y|U|x>, but you also don't want U to be "basically equivalent" to a classical CA. How are you defining "basically equivalent", is anything satisfying your equation automatically "basically equivalent"?]
I think the limitations to radius set by material strength only apply directly to a cylinder spinning by itself without an outer support structure. For example, I think a rotating cylinder habitat surrounded by giant ball bearings connecting it to a non-rotating outer shell can use that outer shell as a foundation, so each part of the cylinder that is "suspended" between two adjacent ball bearings is like a suspension bridge of that length, rather than the whole thing being like a suspension bridge of length equal to the total cylinder diameter. Obviously you would need really smooth, low-friction bearings for this to be a plan to consider, although they would also help with wobble. One way of reducing the friction would be a Russian doll configuration of nested cylinders where each one out was rotating less fast than the previous, which (along with bearings etc) could maybe work.
On a similar vein, you could replace the mechanical bearings with a gas or fluid, in which the cylinder is immersed. Similar advantages in damping the wobble modes and (for fluids or very high pressure gases) helping support the cylinder against its own centrifugal weight. The big downside again would be friction.
If this was the setup I would bet on "hard man" fitness people swearing that running with the spin to run in a little more than earth normal gravity was great for building strength and endurance and some doctor somewhere would be warning people that the fad may not be good for your long term health.
Yes, its a bit weird. I was replying because I thought (perhaps getting the wrong end of the stick) that you were confused about what the question was, not (as it seems now) pointing out that the question (in your view) is open to being confused.
In probability theory the phrase "given that" is a very important, and it is (as far as I know) always used in the way used here. ["given that X happens" means "X may or may not happen, but we are thinking about the cases where it does", which is very different from meaning "X always happens"]
A more common use would be "What is the probability that a person is sick, given that they are visiting a doctor right now?". This doesn't mean "everyone in the world is visiting a doctor right now", it means that the people who are not visiting a doctor right now exist, but we are not talking about them. Similarly, the original post's imagined world involves cases where odd numbers are rolled, but we are talking about the set without odds. It is weird to think about how proposing a whole set of imaginary situations (odd and even rolls) then talking only about a subset of them (only evens) is NOT the same as initially proposing the smaller set of imaginary events in the first place (your D3 labelled 2,4,6).
But yes, I can definitely see how the phrase "given that", could be interpreted the other way.
That Iran thing is weird.
If I were guessing I might say that maybe this is happening:
Right now the more trade China has with Iran the more America might make a fuss. Either complaining politically, putting tariffs, or calling on general favours and good will for it to stop. But if America starts making a fuss anyway, or burns all its good will, then their is suddenly no downside to trading with Iran. Now substitute "China" for any and all countries (for example the UK, France and Germany, who all stayed in the Iran Nuclear Deal even after the USA pulled out).
"given that all rolls were even" here means "roll a normal 6 sided dice, but throw out all of the sequences that included odd numbers." The two are not the same, because in the case where odd numbers can be rolled, but they "kill" the sequence it makes situations involving long sequences of rolls much less likely to be included in the dataset at all.
As other comments explain, this is why the paradox emerges. By stealth, the question is actually "A: How long do I have to wait for two 6s in a row, vs B: getting two 6's, not necessarily in a row, given that I am post selecting in a way that very strongly favors short sequences of rolls".
I suppose its the difference between the LW team taking responsibility for any text the feature shows people (which you are), and the LW team endorsing any text the feature shows (which you are not). I think this is Richard's issue, although the importance is not obvious to me.
Could be an interesting poll question in the next LW poll.
Something like:
How often do you use LLMs?
Never used them
Messed about with one once or twice
Monthly
Weekly
Every Day