How Risky Is Trick-or-Treating?
post by jefftk (jkaufman) · 2022-10-27T14:10:03.440Z · LW · GW · 18 commentsContents
18 comments
content warning: discussion of child fatalities
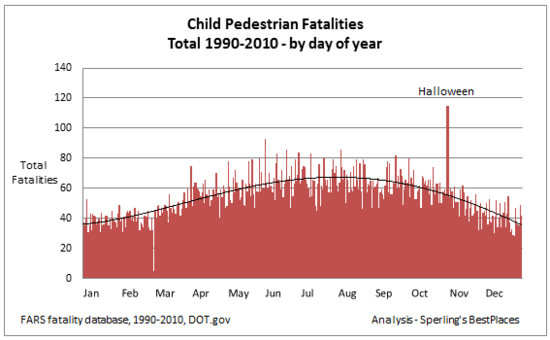
I've seen this chart going around:
Sperling and Smith created it from NHTSA FARS data, and there have been lots of news stories about it or the underlying data. Some people have a "lets make costumes brighter" angle, some see this as ammunition in the fight against car-centric culture (which I support), some people want to move Halloween earlier in the day. But I think everyone is missing the point: Halloween is actually one of the safest days to be out walking as a kid.
Let's look at the data. I started by replicating the chart to verify I was doing it correctly (code):
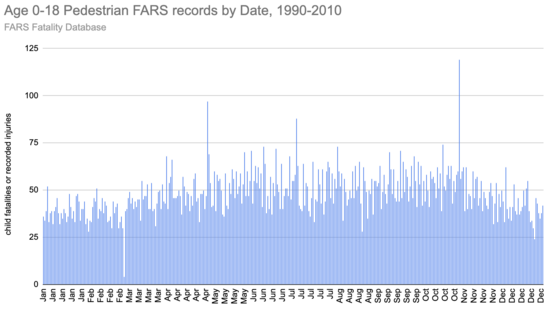
The dip on February 29th is mostly just that this day only occurs once every four years, so it has about a quarter the deaths you'd expect. But what about the spike on 4/30? On April 30th 1992 someone drove their car into a large group of 3rd graders on a field trip, and one of them died. It turns out the FARS records can include cases where someone else died in the same accident. Filtering to just the records representing deaths I get:
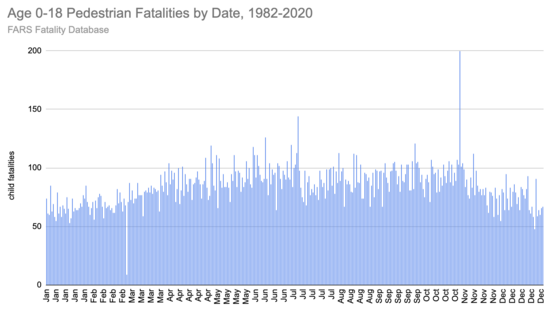
The data available actually goes back to 1982 and now runs to 2020, so we can expand it a bit:
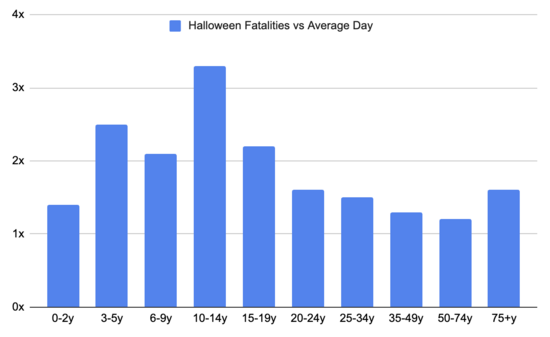
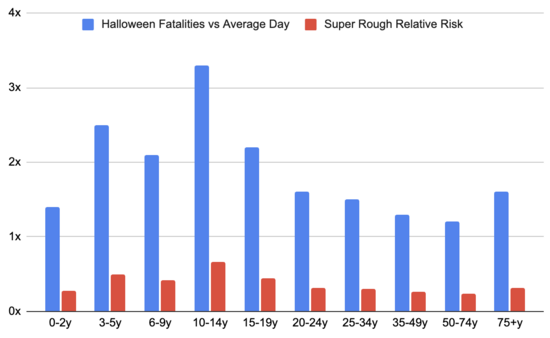
We can also break down by age, since "child" can mean a range of things and most people are likely thinking mostly about younger children:
All this is to confirm the original chart: more children do die on Halloween than on a typical day. But I still don't think that's a reason to keep your kids home on Halloween, even when you set aside the consideration that Halloween is an especially fun time to be a child pedestrian.
The issue is, there are a lot more kids out on Halloween than the typical day. If you learned that more people died in car crashes in the US (42k/y) than in Paraguay (1.6k/y) you wouldn't conclude that Paraguay's roads are safer: the US has 50x more people than Paraguay! We would ideally scale the initial chart by the number of children out walking on any given day, but I don't think anyone has numbers on this. Very roughly, though, maybe it's something like 5x as many people (kids, parents, grandparents) out on foot on Halloween than the typical day?
Every pedestrian is rolling some dice. The dice are pretty favorable: of the ~70M people under age 18 in the US only 320 died in 2020 by being hit by a car. But you still might want to avoid days where the dice were less favorable than usual. Looking at the original chart you might conclude that Halloween was one of those days, but if there are a lot more kids walking around on Halloween it's actually one of the safer days.
Comment via: facebook
18 comments
Comments sorted by top scores.
comment by paulfchristiano · 2022-10-27T16:55:38.233Z · LW(p) · GW(p)
Quantifying average costs:
- This graph is saying that about 200 child pedestrians died on halloween from 1982 to 2020, vs 75 on a normal fall day, so 125 extra deaths over 38 years. Is that right?
- That's about 3.3 excess deaths per year.
- There are about ~40 million kids aged 5 to 14. Some older and younger kids also trick-or-treat, I'd guess overall something close to ~33 million trick-or-treaters per year (farmers' almanac gives an unsourced guess of 35 million).
- So that's about 1/10 million risk of death per trick-or-treater.
- If a parent would pay about 100 years of their life to save their child's life, they'd be willing to pay about 5 minutes to eliminate the trick-or-treat accident risk.
(There were fewer trick-or-treaters in the past, so the denominator is lower than 33 million per year, but I think that's overwhelmed by road safety improvements making modern trick-or-treating safer---total pedestrian fatalities are currently similar to their long-term average despite our growing population, and child fatalities in particular seem even lower.)
comment by tgb · 2022-10-27T18:12:33.654Z · LW(p) · GW(p)
While I appreciate the analysis, I also recently saw this article circulating: https://jamanetwork.com/journals/jamapediatrics/article-abstract/2711459
It compares just 6pm-midnight on Halloween versus the corresponding time one week early and one week later. They estimate a 10x increase in deaths in age 4-8 children - see Figure 1. This doesn't look like subgroup fishing since the 9-12 group is also quite large (6x increase). By your 5x correction factor, Halloween would still be more dangerous than other days for kids.
I still think it could be true that Halloween is less dangerous since this hasn't measured pedestrian activity and trick-or-treat really might be a greater than 10x increase in 4-8 year olds out on the street. But this definitely makes it look less good to me than your presentation.
Replies from: jkaufman↑ comment by jefftk (jkaufman) · 2022-10-27T19:08:50.330Z · LW(p) · GW(p)
That analysis is using the same dataset I'm using, but restricting to 6pm-midnight. This has even more of the denominator problem I'm talking about: almost no kids are out walking 6pm-midnight on a typical day, since that's dinner and bedtime. So 5x is likely much too small a factor to account for how many more kids are out.
Replies from: tgb↑ comment by tgb · 2022-10-27T20:13:14.563Z · LW(p) · GW(p)
Your graph shows an ~40% risk compared to the normal day in that age group. Using their risk ratio you would need about 25x times the child pedestrian activity to achieve that risk reduction. That could be the case, but I'm not certain. I'm not even that confident that you'd get the >10x needed to ensure a decrease in risk. Kids tend to go to hot spots for trick-or-treating, so the really busy streets that get >25x and spring to mind easily might be hiding the (relatively) depleted streets elsewhere that account for a larger fraction of typical walking. Hence I think your presentation is optimistic: it's right to push back on the raw numbers but I don't think it's clear that Halloween is substantially safer than other nights per pedestrian-hour as you claim.
I also read the denominator problem differently. I took your argument to claim that 5x number to be a lower bound for the "trick-or-treating streets compared to the same streets on a typical night" and for that, it's definitely true. But then you had to gloss over the fact that we're comparing entire days (and non-trick-or-treating streets) and it's much less clear that 5x is true for all-of-Halloween compared to all-of-another-day. Therefore, their analysis justified using your 5x number while I think your analysis was stretching the truth.
↑ comment by jefftk (jkaufman) · 2022-10-27T21:30:49.552Z · LW(p) · GW(p)
I would believe 25x, or even 50x for fall evenings, and I think 10x+ is overwhelmingly likely: it's just very few children that are out during those hours normally.
I'm not approaching this by thinking about how many children you see on the street during Halloween, but about how what fraction of children are typically out in the evening and what fraction of children trick or treat.
comment by jmh · 2022-10-28T00:36:47.541Z · LW(p) · GW(p)
While this doesn't change the basic conclusion from the data, does the data provide any data related to:
- adults also injured in the accidents for the children 5 and under?
- Age of the driver of the vehicle?
↑ comment by jefftk (jkaufman) · 2022-10-28T11:44:59.182Z · LW(p) · GW(p)
1: while the data is supposed to include all traffic fatalities, it includes few injuries, and in recent data it only includes fatalities
2: I don't see that in the data:
$ head -n 1 2020/person.csv | tr ',' '\n' | cat -n
1 STATE
2 STATENAME
3 ST_CASE
4 VE_FORMS
5 VEH_NO
6 PER_NO
7 STR_VEH
8 COUNTY
9 DAY
10 DAYNAME
11 MONTH
12 MONTHNAME
13 HOUR
14 HOURNAME
15 MINUTE
16 MINUTENAME
17 RUR_URB
18 RUR_URBNAME
19 FUNC_SYS
20 FUNC_SYSNAME
21 HARM_EV
22 HARM_EVNAME
23 MAN_COLL
24 MAN_COLLNAME
25 SCH_BUS
26 SCH_BUSNAME
27 MAKE
28 MAKENAME
29 MAK_MOD
30 BODY_TYP
31 BODY_TYPNAME
32 MOD_YEAR
33 MOD_YEARNAME
34 TOW_VEH
35 TOW_VEHNAME
36 SPEC_USE
37 SPEC_USENAME
38 EMER_USE
39 EMER_USENAME
40 ROLLOVER
41 ROLLOVERNAME
42 IMPACT1
43 IMPACT1NAME
44 FIRE_EXP
45 FIRE_EXPNAME
46 AGE
47 AGENAME
48 SEX
49 SEXNAME
50 PER_TYP
51 PER_TYPNAME
52 INJ_SEV
53 INJ_SEVNAME
54 SEAT_POS
55 SEAT_POSNAME
56 REST_USE
57 REST_USENAME
58 REST_MIS
59 REST_MISNAME
60 AIR_BAG
61 AIR_BAGNAME
62 EJECTION
63 EJECTIONNAME
64 EJ_PATH
65 EJ_PATHNAME
66 EXTRICAT
67 EXTRICATNAME
68 DRINKING
69 DRINKINGNAME
70 ALC_DET
71 ALC_DETNAME
72 ALC_STATUS
73 ALC_STATUSNAME
74 ATST_TYP
75 ATST_TYPNAME
76 ALC_RES
77 ALC_RESNAME
78 DRUGS
79 DRUGSNAME
80 DRUG_DET
81 DRUG_DETNAME
82 DSTATUS
83 DSTATUSNAME
84 HOSPITAL
85 HOSPITALNAME
86 DOA
87 DOANAME
88 DEATH_DA
89 DEATH_DANAME
90 DEATH_MO
91 DEATH_MONAME
92 DEATH_YR
93 DEATH_YRNAME
94 DEATH_HR
95 DEATH_HRNAME
96 DEATH_MN
97 DEATH_MNNAME
98 DEATH_TM
99 DEATH_TMNAME
100 LAG_HRS
101 LAG_HRSNAME
102 LAG_MINS
103 LAG_MINSNAME
104 WORK_INJ
105 WORK_INJNAME
106 HISPANIC
107 HISPANICNAME
108 LOCATION
109 LOCATIONNAME
110 HELM_USE
111 HELM_USENAME
112 HELM_MIS
113 HELM_MISNAME
114 VPICMAKE
115 VPICMAKENAME
116 VPICMODEL
117 VPICMODELNAME
118 VPICBODYCLASS
119 VPICBODYCLASSNAME
120 ICFINALBODY
121 ICFINALBODYNAME
↑ comment by braces · 2022-10-28T14:58:34.212Z · LW(p) · GW(p)
You can get the age of the driver by looking at the age of the person with PER_TYP equal to one.
Replies from: jkaufman↑ comment by jefftk (jkaufman) · 2022-10-28T16:33:04.435Z · LW(p) · GW(p)
I'm not that familiar with the data, but how would I link that record to a child death?
Replies from: braces↑ comment by braces · 2022-10-28T19:50:34.553Z · LW(p) · GW(p)
Within years, the combo of ST_CASE and STATE uniquely identifies accidents, so an intermediate step is tracking the accident ID. Then you could classify accidents as involving a child as those where at least one of the decedents is under a certain age, then use that PER_TYP variable to look at the ages of the driver(s) for those accidents. Cool analysis, btw--I've worked a lot with the FARS and happy to help :)
comment by dmav · 2022-10-27T19:04:32.038Z · LW(p) · GW(p)
In other words, we should be telling children 'be careful of roads/cars' (including on Halloween) Not 'be careful of Halloween'
I agree with the post, but I will point out that you really do need to emphasize the utility per micromort here. If you keep your utility constant, it is the total risk that matters. Just like if you were going to go on a long car ride tomorrow (on safer-than-usual roads, but not enough to outweigh the total driving) and someone points out you're much more likely to die than usual - sure, you can point out 'ah yes, but the chance I die per-mile is lower than usual!' but that's not the right reference point if your utility isn't a function of the driving-amount.
All that said, the total number of deaths is only ~double on Halloween? That feels so insane, roads must be SO much safer than usual.
Replies from: jkaufman↑ comment by jefftk (jkaufman) · 2022-10-27T19:19:22.258Z · LW(p) · GW(p)
we should be telling children 'be careful of roads/cars' (including on Halloween) Not 'be careful of Halloween'
Yes, but even then not unduly so. Child pedestrian deaths per FARS are about 0.5 per 100k (320 per 72M), which puts them below guns (5 per 100k), driving (4 per 100k), drugs (3 per 100k), suffocation (2 per 100k), drowning (1 per 100k), etc: https://www.jefftk.com/goldstick-causes-of-child-deaths.jpg. Total deaths under age 18 are about 37k/y, so 320 pedestrian deaths is about 1% of deaths. Worth careful teaching, but still fine to let them walk to their friends' houses once they're ready.
utility per micromort here
Not sure I understand what this paragraph is getting at?
roads must be SO much safer than usual.
But it's also dark and chaotic.
comment by RamblinDash · 2022-10-27T15:24:24.337Z · LW(p) · GW(p)
Yeah, the most promising by-day interventions I've heard proposed is day-specific regulation of driving, such as lower speed limits, closing of residential roads to thru traffic, etc.
comment by Daniel V · 2022-10-27T14:55:26.793Z · LW(p) · GW(p)
Nicely done!
The question is what should be the denominator of the risk?
For societal aggregate worries: Day? Sure.
For personal risk: Day? Nope. Person-day? Yes!
↑ comment by jefftk (jkaufman) · 2022-10-27T14:58:36.220Z · LW(p) · GW(p)
Even for societal aggregate worries it's only worth using days as the denominator if the potential interventions you're considering scale by days and not person-days. For example "stay-home on Halloween" or "move Halloween earlier" has costs proportional to the latter.
comment by rvnnt · 2022-10-28T10:43:47.332Z · LW(p) · GW(p)
What about (e.g.) risks from kids ingesting candy sourced from multiple unknown people (any one of whom could poison a whole lotta kids with decent chances of not getting caught)? Maybe tell kids to only accept cash?
Replies from: jkaufman↑ comment by jefftk (jkaufman) · 2022-10-28T11:42:52.838Z · LW(p) · GW(p)
risks from kids ingesting candy sourced from multiple unknown people (any one of whom could poison a whole lotta kids with decent chances of not getting caught)?
This is something people have worried about a bunch, but it doesn't actually happen: https://en.wikipedia.org/wiki/Poisoned_candy_myths
Replies from: mr-hire↑ comment by Matt Goldenberg (mr-hire) · 2022-10-28T16:41:08.796Z · LW(p) · GW(p)
Afaict that page says it happened twice - William Shyne gave out candy coated laxative pills, and Ronald O'Bryce gave out poison-laced pixie stix