The economics of space tethers

post by harsimony · 2024-08-22T16:15:22.699Z · LW · GW · 22 comments

This is a link post for https://splittinginfinity.substack.com/p/the-economics-of-space-tethers

Contents

  Tether materials and characteristic velocity
  Delta V and fuel savings
  Tether mass ratio and fuel costs
  Numerical examples
  Conclusion
  Appendix
    Tether materials
    Why tether material doesn’t matter too much
    Single stage to orbit is silly
None
22 comments

Some code for this post can be found here.

Space tethers take the old, defunct space elevator concept and shorten it. Rockets can fly up to a dangling hook in the sky and then climb to a higher orbit. If the tether rotates, it can act like a catapult, providing a significant boost in a location where providing thrust is expensive. Kurzgesagt has a nice explainer and ToughSF has a great piece explaining the mechanics and some applications.

Tethers make it cheaper to explore space, but how much cheaper? Let’s look at the benefits.

Tether materials and characteristic velocity

The key performance metric for the tether material is the characteristic velocity:

$V_c=\sqrt{\frac{2T}{\rho }}$

Where T is the tensile strength and rho is the density.

The stronger and lighter the material is, the faster the tether can spin, boosting payloads to higher speeds and saving more fuel. This quickly leads to thinking about exotic materials. Hexagonal boron nitride! Carbon nanotubes! I’m not immune to this kind of speculation, so I’ve added an appendix on the topic. But as I argue in another part of the appendix, we already have good enough materials to make a space tether. The potential gain from studying exotic materials is actually pretty small.

For what it’s worth, I like glass fibers. They’re pretty easy to make, the material can be be sourced in space, they can handle large temperature ranges, and they’re resistant to atomic oxygen environments and UV [1]. They can also get pretty good performance, S-2 glass fibers have a characteristic velocity close to 2 km/s while the best currently available material clocks in at 2.7 km/s.

Now let’s look at why the speed of the tether matters.

Delta V and fuel savings

Rockets have to reach a certain speed in order to orbit any object. For low earth orbit, that’s roughly 7.9 km/s; that’s over Mach 20 here on Earth. The change in velocity, or delta V (dV), required to reach orbit is the currency of spaceflight. You can essentially map out the solar system based on the delta V needed to reach different places:

Source

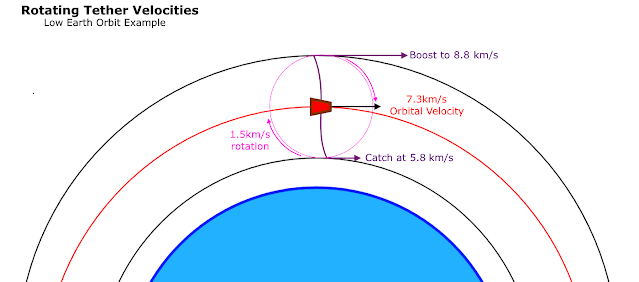
It takes a lot of fuel and engineering to get a payload up to these speeds, making launches expensive [2][3]. Tethers are exciting because they can wait in orbit and offer a rocket some extra delta V. A tether spinning at 1.5 km/s in LEO can grab a rocket moving at 5.8 km/s and release it at 8.8 km/s:

Source

It takes a while to visualize how these work. Staring at this gif helps:

Source

Even a small delta V boost saves a lot of fuel. That’s because the total fuel required for a mission increases exponentially with delta V requirements, as we can see from the Tsiolkovsky rocket equation:

$\Delta V=I_{sp}{g}_0\ln \left(\frac{m_i}{m_p}\right)$

I_sp is the specific impulse of the rocket, g_0 is the gravitational acceleration (often just called *g *in Earth’s gravity), m_i is the total initial mass of the rocket including fuel, and m_p is the payload mass of the rocket after the fuel has been expended. Note that m_p includes both the literal payload and the mass of the rocket itself.

Rearranging to see the exponential:

$m_i=m_p\exp \left(\frac{\Delta V}{I_{sp}{g}_0}\right)$

m_i is the sum of the payload mass m_p and the fuel mass m_x. We can rewrite the above in terms of fuel mass:

$m_x=m_p\left(\exp \right(\frac{\Delta V}{I_{sp}{g}_0})-1)$

By offering a free delta V boost, tethers can save literal tons of fuel. If the tether is spinning at a certain velocity V_t, the tether provides a boost twice that size. You can subtract that boost from the dV requirements for the rocket:

$\Delta V^{\prime }=\Delta V-2V_t$

The new initial mass is:

$m_i^{\prime }=m_p\exp \left(\frac{\Delta V-2V_t}{I_{sp}{g}_0}\right)$

The new fuel requirement is:

$m_x^{\prime }=m_i^{\prime }-m_p=m_p\left(\exp \right(\frac{\Delta V-2V_t}{I_{sp}{g}_0})-1)$

As an example, let’s imagine a tether orbiting in LEO [4] at an orbital velocity of 7.5 km/s and spinning at 2 km/s. Our rocket only needs to reach 5.5 km/s in order to be boosted to 9.5 km/s. A Starship mission could involve:

1. A Superheavy booster that lifts Starship out of the atmosphere and up to a speed of 1.5 km/s
2. Starship then adds an additional 4 km/s to catch the tether at 5.5 km/s (instead of needing 8 km/s without the tether)
3. The tether boosts starship up to 9.5 km/s to something like geostationary transfer orbit.

Plugging in these numbers along with the I_sp (380s), m_p (200 t), and m_x (3400 t) for Starship, we get a fuel requirement that is 8.83 times smaller!

As you can see, tethers dramatically lower fuel requirements. But designing one isn’t easy. In the next section, we’ll see how to choose the overall mass and tip speed of a tether to minimize fuel costs for a particular orbital transfer.

Tether mass ratio and fuel costs

For a tether to swing hundreds of tons of mass at high speeds, it needs to be pretty large. The “mass ratio” is the number of times larger the tether needs to be relative to its payload:

$MR=\sqrt{\pi }s\frac{V_t}{V_c}\exp \left(\frac{V_t^2}{V_c^2}\right)$

Where *s *is a safety factor greater than 1 that we include to make sure the tether always has sufficient mass to handle its payload.

The mass ratio is really the number of launches we need to perform just to get a tether with sufficient mass into orbit, assuming that the “construction launches” have the same size payload as the “production launches” when the tether is acutally in use. This is the key cost of building a tether since we have to invest a bunch of launches just to build it [5]. The total fuel cost for these launches is:

$C=m_p\left(\exp \right(\frac{V_o}{I_{sp}{g}_0})-1)\sqrt{\pi }s\frac{V_t}{V_c}\exp \left(\frac{V_t^2}{V_c^2}\right)$

The only difference between this equation and the last one is the term I’ve added to the front. This is the fuel required to get a payload to the tether’s orbit, V_o is the delta V required to get there.

If the tether only performs a fixed number of boosts (L) before it breaks down, we can divide this initial fuel cost up amongst all the future boosts:

$\frac{C}{L}=\frac{m_p\sqrt{\pi }s}{L}\left(\exp \right(\frac{V_o}{I_{sp}{g}_0})-1)\frac{V_t}{V_c}\exp \left(\frac{V_t^2}{V_c^2}\right)$

That’s the per-launch fuel cost just to build the tether. We know the per-launch fuel requirements from the last section. Putting them together, we get the total fuel cost per mission:

With reusable rockets, fuel is a major cost driver. We want to minimize this overall cost. The equation is pretty messy, but there’s really only one variable here, the velocity V_t that the tether operates at. With a little code, we can find the optimal V_t for a particular delta V required for a particular orbital transfer.

Numerical examples

So let’s plug in some numbers. To keep things simple, let’s assume our rocket is already in LEO so we don’t have to worry about a booster [6]. To boost the rocket to the moon we’re going to need 5.66 km/s of delta V.

Let’s keep all of the parameters the same from the last example and assume that the tether has a characteristic velocity of 2 km/s, can survive for 100 launches and use a safety margin (s) of 2x. However, instead of a tether that rotates at 2 km/s like the last example, we’re going to choose an optimal speed.

Optimizing the equation [7], a tether that spins at 1.74 km/s is ideal. The fuel cost per launch is 293 tonnes, less than half the 713 tonnes we needed originally. The required size of a tether spinning at this speed is 1310 tonnes, which should take roughly 10 launches to build.

As a second example, we can try boosting all the way to Mars for a dV of 9.31 km/s. In this case the ideal tether spins at 2.19 km/s and has a mass of 2560 tonnes. Now, the trip requires 813 tonnes of fuel versus 2230 tonnes without a tether.

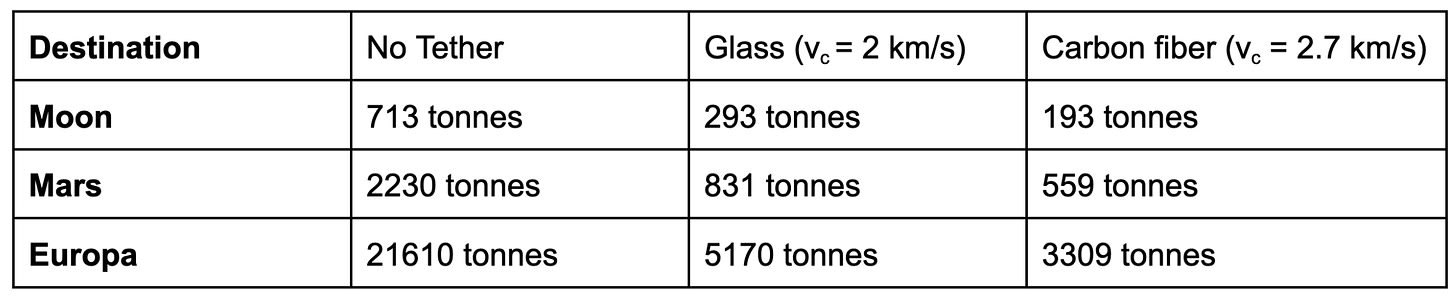
In the table below, I list the fuel requirements before and after a tether for several destinations:

Even short-lived tethers can lower fuel costs 2-4x to nearby destinations. But they really shine for faraway locations like Europa. A carbon fiber tether can reduce fuel costs by almost an order of magnitude.

These gains are pretty nice, but in an era where launch costs have fallen by orders of magnitude, that’s not that much. On top of this, the size of the optimal tether means that we need 10’s to 100’s of launches just to get it constructed. In other words, we’re investing dozens of launches in order to save fuel on the subsequent 100 launches.

The large upfront investment means that tethers will only become viable after we have a mature space industry. We haven’t reused a single Starship yet, so it doesn’t make sense to build a tether anytime soon.

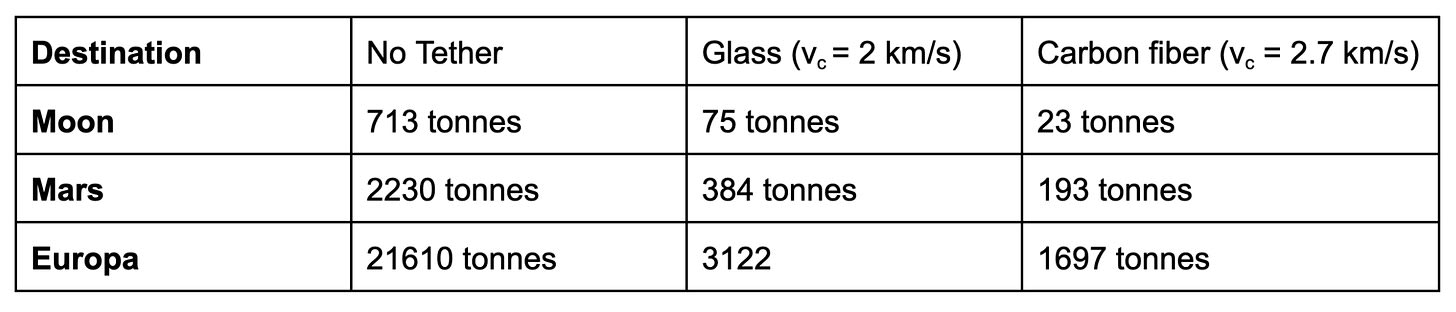
What happens if the tether lasts longer? Say 1000 launches instead of 100. Then we get:

That’s a big difference! By spreading construction costs over more launches, we can lower fuel costs even to nearby destinations by an order of magnitude or more. The optimal tethers here spin much faster, close to double their characteristic velocity, and are much heavier, requiring hundreds of launches to construct. Their size means that these tethers won’t become a reality for a long time, but once they’re built, they will have a dramatic effect on launch costs.

My big takeaway here is that designing a tether for longevity is much more important than using fancy materials. Tethers that are resistant to damage, easy to inspect, and easy to repair in orbit will be of more practical value than ones with slightly higher characteristic velocity.

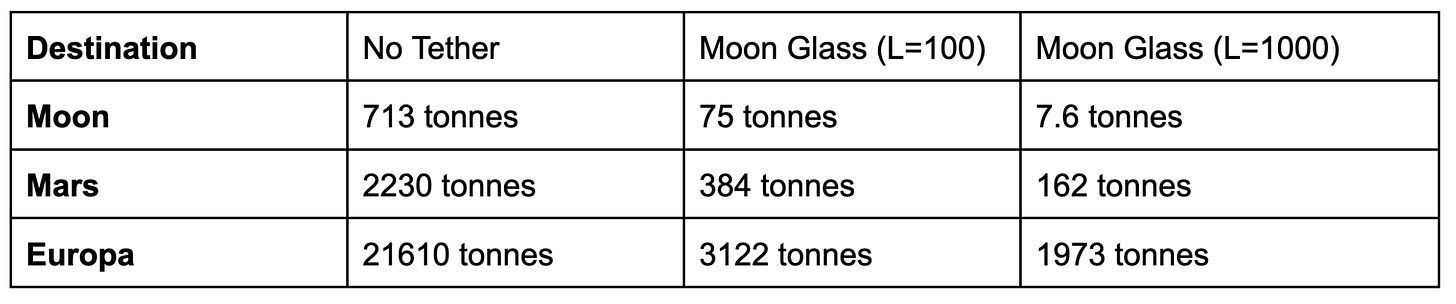
One last possibility I want to consider is sourcing material for a glass tether from the moon. I’ve estimated that lunar industry could reduce launch costs to LEO by an order of magnitude or more. Let’s factor that in by scaling the initial cost of building a glass tether by 10x [8]. Now we get:

Lunar glass starts looking better than carbon fiber for nearby destinations (low dV), though it still struggles with longer distances and higher velocities.

Conclusion

After staring at tether design for a while, I’ve come to a few realizations:

1. Tethers help you “skip a level” by bouncing you out of the orbit they’re in. They specialize in a particular transfer you want to make. That’s not always desirable. For instance, there’s not really a way to use tethers to get you from Earth to LEO, since the tether has to be in LEO already. The tether could do a “half-lift” to get you to LEO, but that doesn’t utilize their strengths very well.
2. Tethers generally want to operate close to their characteristic velocity. Slower and you’re wasting their potential, higher and your tether needs to be huge in order to handle the payload.
3. Tether repair is a big deal. The longer a material can last, the more it justifies building bigger and faster tethers, which leads to big fuel savings.
4. Tethers are surprisingly complex for a piece of string. Matching up rocket and tether velocities sometimes requires the tether to be in a weird orbit, and tethers orbit differently than a condensed object would. On top of that, you need to damp vibrations, keep the tether material cool and repair defects in order to get high performance. And they need a regular supply of falling mass to counter the momentum lost from boosting rockets. These considerations mean that tethers have to constantly adapt to their conditions, frequently repositioning and doing maintenance.

Tethers work best at a scale that is currently out of reach for modern launch industry. The fastest way to make them a reality is having a booming space industry and maybe a moon base. Once those things are in place, small tethers can deliver fuel savings, spurring investment in larger tethers and better materials [9].

Large, established tethers can lower costs by 1 to 2 orders of magnitude or more. Farther in the future, tethers on other planets can unlock the entire solar system [10]. Launch industry may adapt by building simpler, cheaper, lower-performance rockets.

These benefits stack on top of sourcing building materials and fuel from the Moon, Mars, or asteroids [11]. For example, a tether in LEO can boost a Starship up to GTO where they can rendezvous with LOX, metals, and nuclear fuel sourced from the moon. Tethers can also be used as atmospheric scoops to gather useful resources from the upper atmosphere. In addition, they can help slow spacecraft for reentry, reducing heat shielding requirements.

I think it’s clear that tethers will usher in another revolution in space travel, though we will have to wait a while for that day to come. The engineering involved is far from simple, making tethers a worthy challenge on our quest to reach space.

Appendix

Tether materials

At an atomic level, we want a material with light atoms that make multiple strong bonds. Single-bonding atoms like hydrogen or fluorine add weight without making connecting bonds, so should be avoided. Instead, carbon-based materials, boron-nitrides, and oxides like glass are promising.

Defects are critical to material strength. Even under loads well below the tensile strength of a material, defects can propagate and eventually weaken it. This creep behavior is important to study and fundamentally limits how many times a tether can be reused. Fracture toughness is another key performance metric.

Materials with no flaws or defects are much, much stronger than everyday materials, so we want to make something at scale that is nearly flawless. This is why like carbon nanotubes end up looking so good in the lab, because the sample is so small there are no defects. But when we scale up production, some nanotubes will inevitably contain defects and be much weaker[12]. If we can make a material without defects at scale, that would lead to higher performance than using an exotic composition.

We could use semiconductor manufacturing techniques to make very pure rods of pure silicon or silicon carbide. Another option is to use chemical vapor deposition and related techniques to make sheets of defect-free silicon dioxide, diamond, or other materials. Data from this page suggests that sheets of silicon dioxide could have a characteristic velocity of 2.88 km/s. Though turning this into a roll-to-roll process at high volume would be a challenge.

Carbon fiber is the best material we have right now. In fact, Toray just made an improved version of their composite that has a characteristic velocity of 2.97 km/s. Can carbon fiber just keep getting better? I think things would move faster of these companies were designing explicitly for space, but the last iteration of their fiber debuted in 1986. I’m not sure how much more we can squeeze out of current methods, but maybe this would improve with scale.

Tether materials operate in a pretty interesting environment. For one, there isn’t much atmosphere in LEO, but what remains is a dilute atomic oxygen environment that’s pretty corrosive. This is another reason why I like glass as a tether material, it resists corrosion[13][14].

For another, heat can’t dissipate quickly in a vacuum. Solar irradiation and repeated mechanical strain will heat up the tether material, which is important because heat lowers the strength of most materials.

It’s possible to use this to our advantage; materials that reflect infrared radiation and radiate heat away effectively (e.g. glass) tend to stay quite cold even under solar illumination. The tether itself can spin in Earth’s orbital plane, minimizing solar irradiation and reducing collisions with atomic oxygen.

Low temperature makes materials like glass stronger by preventing cracks from propagating. This means that glass fibers can be even stronger than we’re used to on Earth. We can push this further by actively cooling the fibers using liquid nitrogen sourced from the upper atmosphere[15]. Under these conditions, glass fibers could exceed characteristic velocities of 2.6 km/s. Perhaps with even purer glass fibers and low temperatures, they could get close to their theoretical strength, achieving 4 km/s characteristic velocity[16]. Cooling carbon fibers will also probably help, but I’m not sure how much.

Why tether material doesn’t matter too much

Despite the exciting research opportunities above, I’ve become less interested in tether material research of late. For one, we haven’t seen much progress in finding strong materials that we can mass produce. Our current best candidate is Toray 1200G carbon fiber with a characteristic velocity of 2.97 km/s and we haven’t seen a new contender recently. That’s great and all, but boring glass fiber has a characteristic velocity of roughly 2 km/s and cooling can get that up to 3 km/s or higher.

Is it really worth it to make a tether out of something fancy when you can already get pretty good performance out of sand? Perhaps, but one of the nice things about tethers is that you can stack them in separate orbits. So if a material A offers you twice the characteristic velocity of material B, you can match that by building 2 separate tethers out of material B and boosting twice.

As we’ve seen, tethers that have a characteristic velocity much higher than their mission requires aren’t of much use, they’ll still need to operate near the mission dV. Momentum storage requirements also mean the mass ratio has to be 10-100x the payload, so more characteristic velocity doesn’t help much [17].

Worse, the crazy numbers we get for things like carbon nanotubes or graphene are on tiny, perfect materials that are unlikely to scale. The strength of a macroscale material with all its imperfections is 10-100 times lower than a perfect crystal. Additionally, most of the measurements on things like graphene are just wrong.

But let’s grant the gaudy numbers we see on wikipedia. Graphene gives us a characteristic velocity of 11.4 km/s. Pretty remarkable, but we could replicate that by stacking 3 or 4 tethers made out of materials we have today. Alternatively, tethers of a worse material can operate at higher speeds if you make them (much) larger.

So the stuff we have is already good enough, there are ways to get much more performance without better materials, and there isn’t a clear path to making materials at scale that are far stronger than what we have already. I think the decision over what material to use will really come down to little details like manufacturing scalability, ease of repair, material fatigue, predictability, and ability to withstand the harsh environment of space.

Single stage to orbit is silly

I’ll admit that after looking at exotic tether materials I spent time on another dead end: single-stage to orbit. Tethers and other contraptions have been offered as a way to use a single rocket to get to orbit. Ostensibly, this would save on costs because you only need to design and fuel a single rocket stage with no need for different engines, hot staging, etc.

But I realized this dream was impractical when I looked at at SpaceX’s superheavy booster. It only offers a delta V of roughly 1.5 km/s and an altitude of 100 km. At such a low speed and altitude, a tether would have to spin impractically fast to get a rocket to orbit. There are rockets capable of much higher performance, so why does Superheavy go so slow?

I realized that the engineers at SpaceX choose this for a reason. The faster a reusable booster goes, the more work you have to do to slow it down and land (and the more heat shielding you need) all of which add cost. In addition, rockets aren’t as efficient in the atmosphere and moving faster means more drag.

This means that the first stage should specialize in leaving the atmosphere at a minimally sufficient speed. From there you can use rockets optimized for space[18].

In the future, a different system such as catapaults, cannons, railguns, or hypersonic planes could perform the job of the first stage, but I’m not holding my breath. Building a bigger rocket is probably a better idea for the time being.

Ironically, none of these can actually get up to orbital velocity on their own, so you’d still have to put a rocket on the end regardless. It’s cheaper and simpler to use a rocket as the first stage and the fuel savings you get from these designs probably isn’t enough to outweigh their development cost and risk, especially since methalox fuel might get cheap.

[1] Though I could quickly change my mind if someone brought up a different consideration.

[2] You’ll notice that just getting to low earth orbit is hugely expensive, which is why it’s common to say that LEO is “halfway to anywhere”.

[3] You’ll also notice that the delta-V required to get to LEO is higher than orbital velocity. This is because you need additional fuel to overcome aerodynamic drag and gravity drag. Once in orbit, these are no longer a problem.

[4] Locating a tether in LEO is nice because you don’t have to worry as much about radiation or micrometeorites. Any new produced fragments produced from micrometeor collisions will also have orbital decay.

[5] The fuel costs for the first stage are only included implicity in the original delta V, modelling this completely would be more complex. I’m also not incuding costs of the material itself or the cost to spin up the tether. A BOTEC says that material cost will add 10-20% to the cost while spinning up will only add 1 or 2 additional launches (or perhaps some ion engines or solar sails). Including these costs would lead to smaller, slower tethers overall.

[6] Another wrinkle is that I’m assuming the tether is swinging an empty Starship. In reality, it would have a little fuel so that it can provide the last bit of dV. For now, we can assume that Starship provides all of its dV first and the tether boosts it afterwards.

[7] Under the constraint that the tether can’t boost us more than the desired dV.

[8] Since I’m just multiplying C/L by 0.1 this is the same as multiplying L by 10, so column 2 is the same as the glass column on the previous table.

[9] Small tethers make building large tethers cheaper by lowering the cost to reach higher orbits. So we can bootstrap to larger sizes.

[10] In fact, you could even spin the tether up near Earth and ship it to other planets. Once there, tethers can harvest gravitational energy to keep the whole network spinning.

[11] Tethers also “subsidize” moon mining and asteroid mining since they need falling mass to spin them up.

[12] Though you can get high performance by making many nanosized structures and putting them together. This is how carbon fiber works. The small size limits the number of defects a material can have, making things like monocrystalline whiskers and small glass fibers very strong.

[13] Glass might also perform better in space because there’s no water to weaken the fibers and fibers pulled in microgravity can be stronger than their counterparts on Earth.

[14] One option is for a glass tether to boost carbon fiber tether to higher orbit where safe from atomic oxygen.

[15] I imagine it rolling along the surface of the tether. The LN2 can also shield the material from atomic oxygen and radiation somewhat.

[16] These papers find glass fibers can hit 11-14 GPa tensile strength (3-3.5 km/s), though these bending tests might not be fair:

Strength of Optical Silica Fibers Measured in Liquid Nitrogen

Using the two-point bend technique to determine failure stress of pristine glass fibers

[17] Though there is probably a maximum size the tether can be to avoid danger to Earth.

[18] Though it sounds simpler to use a single stage for the whole flight, in reality building a single rocket to handle atmosphere and space is far more complex and less efficient.

22 comments

Comments sorted by top scores.