PD-alikes in two dimensions

philh

PD-alikes in two dimensions

post by philh · 2022-04-23T09:52:38.765Z · LW · GW · 5 comments

This is a link post for http://reasonableapproximation.net/2022/04/03/pdlikes-2d.html

5 comments

Some time after writing Classifying games like the prisoner's dilemma [LW · GW], I read a paper (I forget which) which pointed out that these games can be specified with just two numbers.

Recall that they have the following payoff matrix:

		Player 2
		Krump	Flitz
Player 1	Krump		$(X, Y)$
	Flitz	$(Y, X)$	$(Z, Z)$

where $W > Z$ .^[1] We can apply a positive affine transformation (that is, $n \mapsto a n + b$ where $a > 0$ ) to all of $W, X, Y, Z$ without changing the game. So let's pick the function $n \mapsto \frac{n - Z}{W - Z}$ . This sends $W$ to $1$ and $Z$ to $0$ , leaving us with just two parameters: $R = \frac{X - Z}{W - Z}$ and $S = \frac{Y - Z}{W - Z}$ .

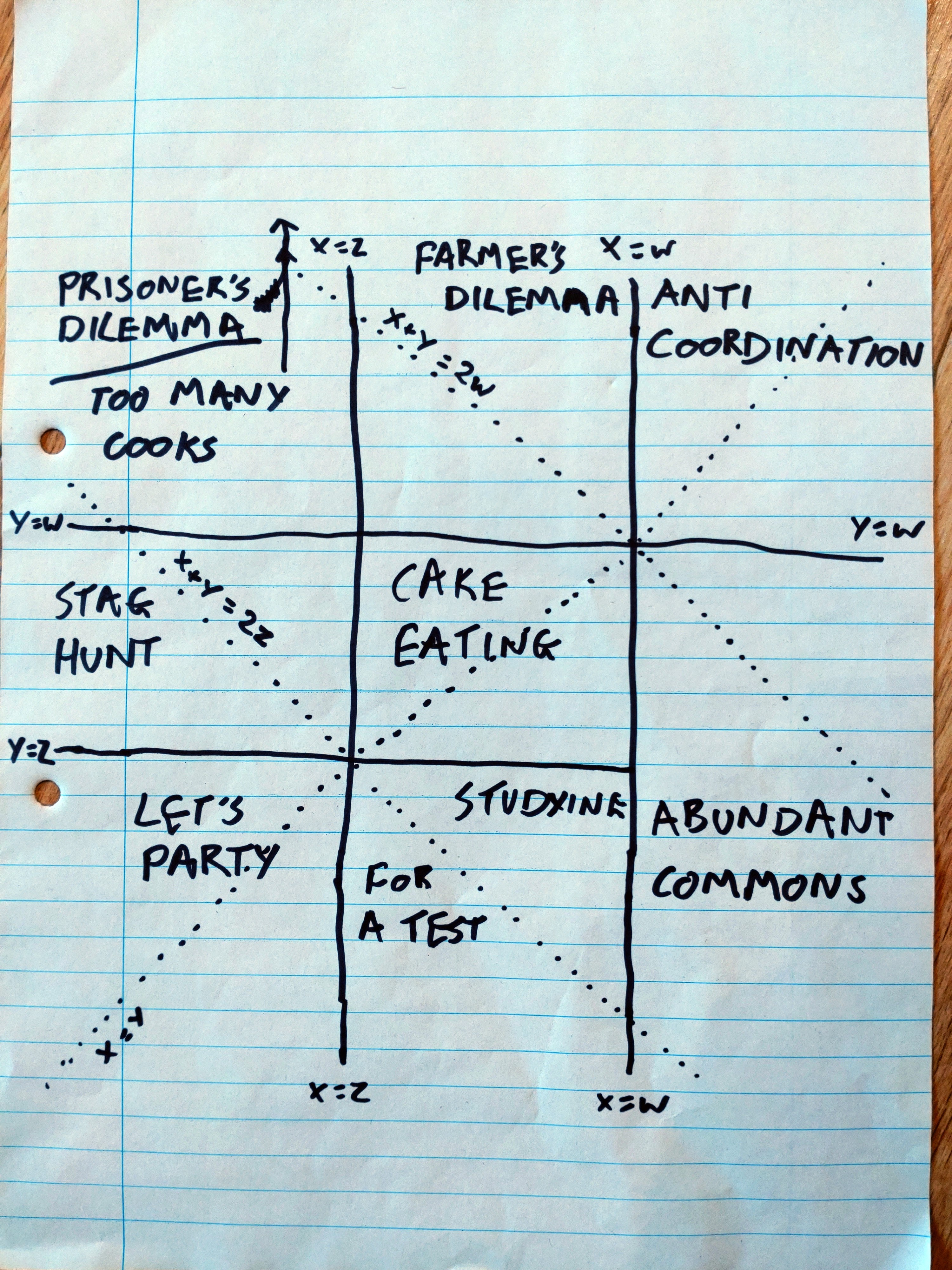
So what happens if we plot the space of these games on a graph? The lines ${X, Y} = {W, Z}$ become ${R, S} = {0, 1}$ , i.e. vertical and horizontal lines. The lines $X + Y = 2 W$ and $X + Y = 2 Z$ become the diagonals $R + S = 2$ and $R + S = 0$ ; and $X = Y$ becomes the diagonal $R = S$ . Drawing those lines, and relabelling in terms of $W, X, Y, Z$ , it looks like this:

Note: I tried to limit the vertical size of that but couldn't figure out how. Sorry!

Note that Cake Eating (my favorite game) is the only one with a finite boundary; the other boxes extend to infinity. There are also finite components in the Farmer's Dilemma (with $X + Y < 2 W$ ), and Stag Hunt and Studying For a Test (with $X + Y > 2 Z$ ). As drawn, Prisoner's Dilemma occupies almost all of the box it shares with Too Many Cooks; but Too Many Cooks (above the line $X + Y = 2 W$ ) is also infinite. (I initially got those the wrong way around, so the drawing isn't very clear there.)

I don't know if we learn much from this, but here it is.

In the previous post I mostly ignored equalities because it was mildly convenient to do so. But the analysis here completely fails if we allow $W = Z$ . So now I'm ignoring them because it's considerably more convenient to do so. ↩︎

5 comments

Comments sorted by top scores.

comment by Jerdle (daniel-amdurer) · 2023-06-23T10:40:01.269Z · LW(p) · GW(p)

Loosely and non-rigorously, x/0 is infinite, and so all games with W=Z are extreme forms of the corner games (unless X=W=Z or Y=W=Z). X~Y>W=Z gets you an anti-coordination game and W=Z>X~Y gets you a pure or relatively pure coordination game (Let's Party).

Y>W=Z>X and X>W=Z>Y are interesting, because they equate games as different as the PD (or Too Many Cooks) and Abundant Commons. I would describe this game as more similar to the Abundant Commons than the PD, as Flitz/Flitz is a perfectly acceptable equilibrium. The value transfer here is neither hyperefficient nor inefficient, but merely efficient.

The triple equalities here are equivalent under name change, so, WLOG, let's take X=W=Z. Then, there are two games: Y>X and Y<X. Looking at the diagram, X=W=Z>Y should resemble Studying for a Test, while Y>X=W=Z should resemble the Farmer's Dilemma.

The former game has a primary theme of avoiding Y, and so, while Flitz/Flitz is an equilibrium, I would expect to see more Krump/Krump, as it is never beneficial to play Flitz when there's any risk of Krump.

The latter game is more complex, but the equilibrium you actually see is Flitz/Flitz, because the only way to get Y is if you play Flitz.

Finally, with all four equal, there is no longer much of a game. All strategies are equilibria, the payoff is identical in each case. This is the trivial game.

Replies from: daniel-amdurer

↑ comment by Jerdle (daniel-amdurer) · 2023-08-15T15:17:15.483Z · LW(p) · GW(p)

Expanding on the Y>W=Z>X and X>W=Z>Y, I would split Abundant Commons at Y=Z, into Abundant Commons above the line and Deadlock below it. Then, the games equated are Deadlock and the PD, and those form a natural continuum.

comment by aphyer · 2022-04-23T11:53:23.023Z · LW(p) · GW(p)

I'm not familiar with all these games by name, and feel like the post would be better with some explanations. I'm particularly confused by how Y very negative and X very positive becomes 'abundant commons'. Attempting to Google 'abundant commons game' doesn't immediately clarify.

Replies from: MondSemmel

↑ comment by MondSemmel · 2022-04-23T12:19:49.509Z · LW(p) · GW(p)

These are all explained (albeit very briefly) in philh's original essay [LW · GW], mentioned in the first sentence.

Replies from: aphyer

↑ comment by aphyer · 2022-04-23T12:26:06.672Z · LW(p) · GW(p)

Ah indeed, thank you!

PD-alikes in two dimensions

Contents

5 comments