Extreme risk neutrality isn't always wrong

post by Grant Demaree (grant-demaree) · 2022-12-31T04:05:18.556Z · LW · GW · 19 comments

Contents

  Intro to the Kelly Criterion
  Implicit assumption in the Kelly Criterion
  Treat risk preferences like any other preference
  Did these ideas affect the FTX crash?
  Conclusions
None
20 comments

Risk neutrality is the idea that a 50% chance of $2 is equally valuable as a 100% chance of $1. This is a highly unusual preference, but it sometimes makes sense:

Exampleville is infested with an always-fatal parasite. All 1,024 residents have the worm.

For each gold coin you pay, a pharmacist will deworm one randomly selected resident.

You only have one gold coin, but the pharmacist offers you a bet. Flip a weighted coin, which comes up heads 51% of the time. If heads, you double your money. If tails, you lose it all.

You can play as many times as you like.

It makes sense to be risk neutral here. If you keep playing until you've saved everyone or go broke, each resident gets a 0.51^10 = 1 in 840 chance of survival. If you don't play, it's 1 in 1,024.

For a few of the most scalable interventions, I think EA is like this. OpenPhil seems to expect a very low rate of diminishing returns for GiveWell's top charities. I wouldn't recommend a double-or-nothing on GiveWell's annual budget at a 51% win rate, but I would at 60%.^[1] 

Most interventions aren't like this. The second $100M of AI safety research buys much less than the first $100M. Diminishing returns seem really steep. 

SBF and Caroline Ellison had much more aggressive ideas. More below on that.

Intro to the Kelly Criterion

Suppose you're offered a 60% double-or-nothing bet. How much should you wager? In 1956, John Kelly, a researcher at Bell Labs, proposed a general solution. Instead of maximizing Expected Value, he maximized the geometric rate of return. Over a very large number of rounds, this is given by

$(1+l{)}^P(1-l{)}^{1-P}$

Where $l$ is the proportion of your bankroll wagered each round, and $P>0.5$ is the probability of winning each double-or-nothing bet.

Maximizing with respect to $l$ gives

$l=2P-1$

Therefore, if you have a 60% chance of winning, bet 20% of your bankroll on each round. At a 51% chance of winning, bet 2%.

This betting method, the Kelly Criterion, has been highly successful in real life. The MIT Blackjack Team bet this way, among many others.

Implicit assumption in the Kelly Criterion

The Kelly Criterion sneaks in the assumption that you want to maximize the geometric rate of return. 

This is normally a good assumption. Maximizing the geometric rate of return makes your bankroll approach infinity as quickly as possible!

But there are plenty of cases there the geometric rate of return is a terrible proxy for your underlying preferences. In the Parasite example above, your bets have a negative geometric rate of return, but they're still a good decision.

Most people also have a preference for reducing the risk of losing much of their starting capital. Betting a fraction of the Kelly Bet gives a slightly lower rate of return but a massively lower risk of ruin. 

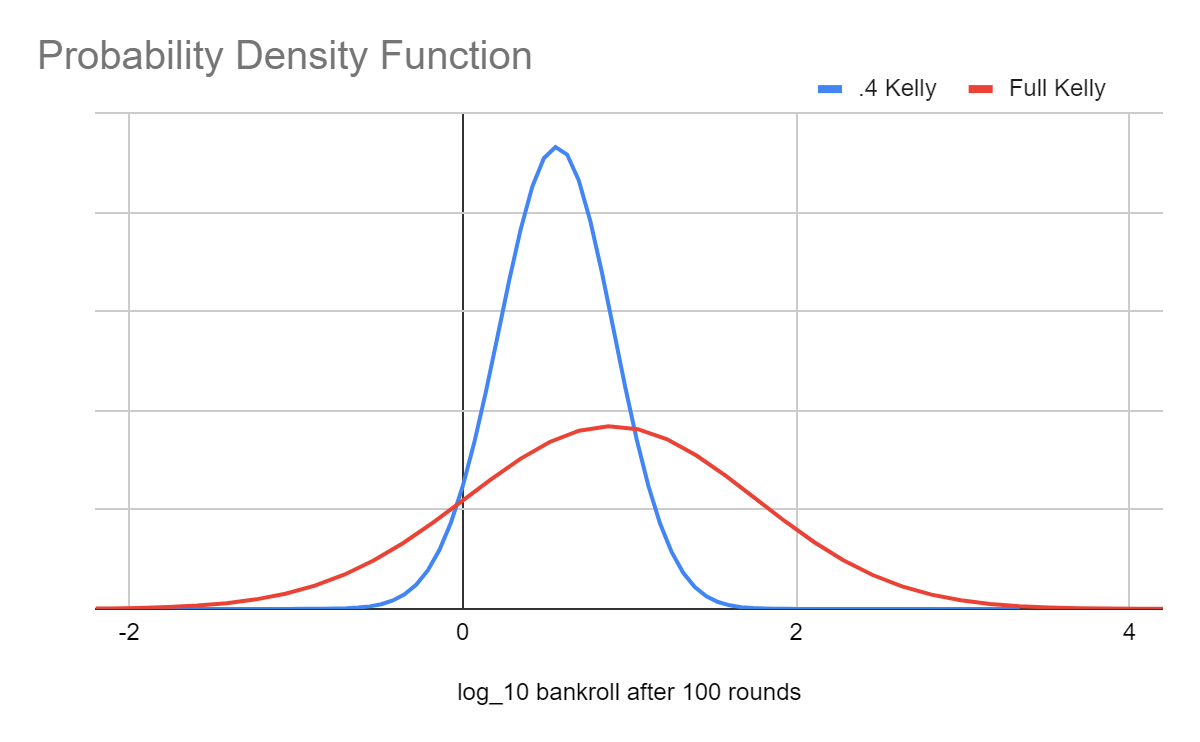
Above: distribution of outcomes after 100 rounds with a 60% chance of winning each bet. The red line (Full Kelly) wagers 20% of the bankroll on each round, while the blue (.4 Kelly) wagers 8%. The x-axis uses a log scale: "2" means multiplying your money a hundredfold, while "-1" means losing 90% of your money.

Crucially, neither of these distributions is better. The red gets higher returns, but the blue has a much more favorable risk profile. Practically, 0.2 to 0.5 Kelly seems to satisfy most peoples' real-world preferences.

Treat risk preferences like any other preference

SBF and Caroline Ellison both endorsed full Martingale strategies. Caroline says:

Most people don’t do this, because their utility is more like a function of their log wealth or something and they really don’t want to lose all of their money. Of course those people are lame and not EAs; this blog endorses double-or-nothing coin flips and high leverage.

She doesn't specify that it's a weighted coin, but I assume she meant a positive EV bet. 

Meanwhile, SBF literally endorses this strategy for the entire Earth in an interview with Tyler Cowen

[TYLER] ... 51 percent, you double the Earth out somewhere else; 49 percent, it all disappears. Would you play that game? And would you keep on playing that, double or nothing?

SBF agrees to play the game repeatedly, with the caveats that the new Earths are created in noninteracting universes, that we have perfect confidence that the new Earths are in fact created, and that the game is played a finite number of times.

I strongly disagree with SBF. But it's a sincerely held preference, not a mistake about the underlying physical reality. If the game is played for 10 rounds, the choices are

A. One Earth, as it exists today
B. 839/840 chance that Earth is destroyed and a 1/840 chance of 1,024 Earths

I strongly prefer A, and SBF seems to genuinely prefer B

One of my objections to B is that I don't believe a life created entirely makes up for a life destroyed, and I don't believe that two Earths, even in noninteracting universes, is fully twice as valuable as one. I also think population ethics is very unsolved, so I'd err toward caution.

That's a weak objection though. Tyler could keep making the odds more favorable until he's overcome each of these issues. Here's Tyler's scenario on hard mode:

50%, you create a million Earths in noninteracting universes. 50 percent, it all disappears. Would you play that game? For how many rounds? Each round multiplies the set of Earths a millionfold.

I still wouldn't play. My next objection is that it's terrible for SBF to make this decision for everyone, when I'm confident almost the entire world would disagree. He's imposing his preferences on the rest of the world, and it'll probably kill them. That sounds like the definition of evil.

That's still a weak objection. It doesn't work in the least convenient world [LW · GW], because Tyler could reframe the scenario again:

50%, you create a million Earths in noninteracting universes. 50 percent, it all disappears. In a planetwide election, 60% of people agreed to play. You are a senior official with veto power. If you don't exercise your veto, then Earth will take the risk.

I won't answer this one. I'm out of objections. I hope I'd do the right thing, but I'm not sure what that is.

Did these ideas affect the FTX crash?

I think so. I predict with 30% confidence that, by end of 2027, a public report on the FTX collapse by a US Government agency will identify SBF or Caroline Ellison's attitude towards risk as a necessary cause of the FTX crash and will specifically cite their writings on double-or-nothing bets.

By "necessary cause" I mean that, if SBF and Caroline did not have these beliefs, then the crash would not have occurred. For my prediction, it's sufficient for the report to imply this causality. It doesn't have to be stated directly.

I have about 80% confidence in the underlying reality (risk attitude was a necessary cause of the crash), but that's not a verifiable prediction.

Of course, it's not the only necessary cause. The fraudulent loans to Alameda are certainly another one!

Conclusions

A fractional Kelly Bet gets excellent results for the vast majority of risk preferences. I endorse using 0.2 to 0.5 Kelly to calibrate personal risk-taking in your life.

I don't endorse risk neutrality for the very largest EA orgs/donors. I think this cashes out as a factual disagreement with SBF. I'm probably just estimating larger diminishing returns than he is.

For everyone else, I literally endorse wagering 100% of your funds to be donated on a bet that, after accounting for tax implications, amounts to a 51% double-or-nothing. Obviously, not in situations with big negative externalities.

I disagree strongly with SBF's answer to Tyler Cowen's Earth doubling problem, but I don't know what to do once the easy escapes are closed off. I'm curious if someone does.

Cross-posted to EA Forum

1. ^{^}

   Risk neutral preferences didn't really affect the decision here. We would've gotten to the same decision (yes on 60%, no on 51%) using the Kelly Criterion on the total assets promised to EA

19 comments

Comments sorted by top scores.